|
|
 |
| Àâòîðèçàöèÿ |
|
|
 |
| Ïîèñê ïî óêàçàòåëÿì |
|
 |
|
 |
|
|
 |
 |
|
 |
|
| Carslaw H.S. — Introduction to the Theory of Fourier's Series and Integrals |
|
|
 |
| Ïðåäìåòíûé óêàçàòåëü |
 -test for convergence of integrals 104 116 -test for convergence of integrals 104 116
Abel 124 146
Abel’s theorem on the power series 146
Abel’s theorem on the power series, extensions of 149
Absolute convergence of integrals 103
Absolute convergence of series 44
Absolute value 32
Aggregate, bounded 30
Aggregate, bounded above (or on the right) 29
Aggregate, bounded below (or on the left) 29
Aggregate, general notion of 29
Aggregate, limiting points of 31
Aggregate, upper and lower bounds of 30
Aggregate, Weierstrass’s theorem on limiting points of 32
Approximation curves for a series 124 (see also “Gibbs’s phenomenon”)
Baker 122
bernoulli 1 3
BLISS 77
Bocher 8 234 243 263 268—271 275 282
Bocher’s treatment of Gibbs’s phenomenon 268
Bonnet 97
Bounds (upper and lower) of an aggregate 30
Bounds (upper and lower) of f(x) in an interval 50
Bounds (upper and lower) of f(x, y) in a domain 72
Boussinesq 7
Bromwich 28 48 150 157 163 165 192 193
Bromwich’s theorem 151
Brunei 119 193
Burkhardt 2 15
Byerly 198
Cantor 12 14 15 26 27 29
Carse and Shearer 298
Carslaw 155 264
Cauchy 8 9 77 124
Cesaro 12 151
Cesaro’s method of summing series (CI) 151 238—240
Change of order of terms in a conditionally convergent series 47
Change of order of terms in an absolutely convergent series 45
Chapman 13
Chrystal 148
Clairaut 3 5
Closed interval, definition of 49
Conditional convergence of series, definition of 45
Continuity of 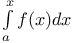 when f(x) is bounded and integrable 93 when f(x) is bounded and integrable 93
Continuity of functions 59
Continuity of infinite integrals involving a single parameter 179 183
Continuity of ordinary integrals 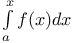 , involving a single parameter 169 , involving a single parameter 169
Continuity of the power series (Abel’s theorem) 146
Continuity of the sum of a uniformly convergent series of continuous functions 135
Continuous functions of two variables 73
Continuous functions, integrability of 84
Continuous functions, non-differentiable 77
Continuous functions, theorems on 60
Continuum, arithmetical 25
Continuum, linear 25
Convergence of functions 51
convergence of integrals 98 (see also “Absolute convergence” “Conditional and
Convergence of sequences 33
Convergence of series 41
Cosine integral (Fourier’s integral) 284 292
Cosine series (Fourier’s series) 197 215
Darboux 4 6 7
Darboux’s theorem 79
De La Vallee Poussin 10 12 28 48 75 77 119 163 192 193 239 243 262 263
Dedekind 18 19 23 25—28
Dedekind’s axiom of continuity 24
Dedekind’s sections 20
Dedekind’s theorem on the system of real numbers 23
Dedekind’s theory of irrational numbers 18
Definite integrals containing an arbitrary parameter (Chapter VI.), continuity, integration and differentiation of 169 179
Definite integrals containing an arbitrary parameter (Chapter VI.), infinite integrals 173
Definite integrals containing an arbitrary parameter (Chapter VI.), ordinary integrals 169
Definite integrals containing an arbitrary parameter (Chapter VI.), uniform convergence of 174
Definite integrals, ordinary (Chapter IV.), considered as functions of the upper limit 93
Definite integrals, ordinary (Chapter IV.), Darboux’s theorem 79
Definite integrals, ordinary (Chapter IV.), definition of 81
Definite integrals, ordinary (Chapter IV.), definition of upper and lower integrals 81
Definite integrals, ordinary (Chapter IV.), first theorem of mean value 92
Definite integrals, ordinary (Chapter IV.), necessary and sufficient conditions for existence 82
Definite integrals, ordinary (Chapter IV.), second theorem of mean value 94 (see also “Dirichlet’s integrals” “Fourier’s “Infinite
Definite integrals, ordinary (Chapter IV.), some properties of 87
Definite integrals, ordinary (Chapter IV.), the sums S and s 77
Descartes 26
Differentiation of Fourier’s series 261
Differentiation of infinite integrals 182
Differentiation of ordinary integrals 170
differentiation of power series 148
Differentiation of series 143
Dini 12 28 112 119 163 193 243
Dirichlet 4 8 9 11 77 200 208
Dirichlet’s conditions, definition of 206
Dirichlet’s integrals 200
Discontinuity of functions 64
Discontinuity, classification of 65 (see also “Infinite discontinuity” and “Points of infinite discontinuity”)
Divergence of functions 51
Divergence of integrals 98
Divergence of sequences 37
Divergence of series 41
Donkin 124
du Bois-Reymond 12 34 77 97 112
D’Alembert 1 3
Euclid 25 26
Euler 1—5
Fejer 13 234 258
Fejer’s theorem 234
Fejer’s theorem and the convergence of Fourier’s series 240 258
FOURIER 2—9 243 294
Fourier’s constants, definition of 196
Fourier’s integrals (Chapter X.), cosine and sine integrals 292
Fourier’s integrals (Chapter X.), more general conditions for 287
Fourier’s integrals (Chapter X.), simple treatment of 284
Fourier’s integrals (Chapter X.), Sommerfeld’s discussion of 293
Fourier’s series (Chapters VII. and VIII.) for even functions (the cosine series) 215
Fourier’s series (Chapters VII. and VIII.) for intervals other than $(-\pi, \pi) 228
Fourier’s series (Chapters VII. and VIII.) for odd functions (the sine series) 220
Fourier’s series (Chapters VII. and VIII.), definition of 196
Fourier’s series (Chapters VII. and VIII.), differentiation and integration of 261
Fourier’s series (Chapters VII. and VIII.), Fejer’s theorem 234 240
Fourier’s series (Chapters VII. and VIII.), Lagrange’s treatment of 198
Fourier’s series (Chapters VII. and VIII.), more general theory of 262
Fourier’s series (Chapters VII. and VIII.), order of the terms in 248
Fourier’s series (Chapters VII. and VIII.), Poisson’s discussion of 230
Fourier’s series (Chapters VII. and VIII.), proof of convergence of, under certain conditions 210
Fourier’s series (Chapters VII. and VIII.), uniform convergence of 253
Functions of a single variable of bounded variation 207
Functions of a single variable, bounded in an interval 50
Functions of a single variable, continuous 59
Functions of a single variable, definition of 49
Functions of a single variable, discontinuous 64
Functions of a single variable, integrable 84
Functions of a single variable, inverse 68
Functions of a single variable, limits of 50
Functions of a single variable, monotonic 66
Functions of a single variable, oscillation in an interval 50
Functions of a single variable, upper and lower bounds of 50
Functions of several variables 71
General principle of convergence of functions 56
General principle of convergence of sequences 34
Gibb 301
Gibbs 268—270 282
Gibbs’s phenomenon in Fourier’s series (Chapter IX.) 264
Gibson 15 195 262
Gmeiner see “Stolz and Gmeiner”
Goursat 28 48 59 75 77 94 119 153 163 165 193 243
Gronwall 270
Hardy 13 19 20 48 75 77 94 130 239
Hardy’s theorem 239
Harmonic analyser (Kelvin’s) 295
Harmonic analysis (Appendix I.) 295
Harnack 112
| Heine 11 12 14 26
Hildebrandt 77
Hobson 12 17 28 69 89 94 138 163 193 234 243 263 275
Improper integrals, definition of 113
Infinite aggregate see “Aggregate”
Infinite discontinuity see “Points of infinite discontinuity”
Infinite integrals (integrand function of a single variable),  -test and other tests for convergence of 114 -test and other tests for convergence of 114
Infinite integrals (integrand function of a single variable),  -test for convergence of 104 -test for convergence of 104
Infinite integrals (integrand function of a single variable), absolute convergence of 103
Infinite integrals (integrand function of a single variable), integrand bounded and interval infinite 98
Infinite integrals (integrand function of a single variable), integrand infinite 111
Infinite integrals (integrand function of a single variable), mean value theorems for 109
Infinite integrals (integrand function of a single variable), necessary and sufficient condition for convergence of 100
Infinite integrals (integrand function of a single variable), other tests for convergence of 106
Infinite integrals (integrand function of a single variable), with positive integrand 101
Infinite integrals (integrand function of two variables), continuity, integration and differentiation of 179
Infinite integrals (integrand function of two variables), definition of uniform convergence of 174
Infinite integrals (integrand function of two variables), tests for uniform convergence of 174
Infinite sequences and series see “Sequences” and “Series”
Infinity of a function, definition of 65
Integrable functions 84
Integration of integrals, infinite 180 183 190
Integration of integrals, ordinary 172
Integration of series (ordinary integrals) 140
Integration of series (ordinary integrals), (infinite integrals) 154
Integration of series (ordinary integrals), Fourier’s series 261
Integration of series (ordinary integrals), power series 148
Interval: open, closed, open at one end and closed at the other 49
Inverse functions 68
Irrational numbers see “Numbers”
Jackson 270
JORDAN 12 207 243 294
Jourdain 14
Kelvin, Lord 6
Kneser 14
Kowalewski 119 163
Lagrange 2 3 5 6 198
Laplace 6
Lebesgue 10 12 77 112 119 243 263 294
legendre 6
Leibnitz 26
Limit repeated 127
Limiting points of an aggregate 31
limits of functions 50
Limits of functions of two variables 72
Limits of sequences 33
Lipschitz 12
Littlewood 239
Lower integrals, definition of 81
M-test for convergence of series 134
Mean value theorems of the integral calculus, (infinite integrals) 109
Mean value theorems of the integral calculus, first theorem (ordinary integrals) 92
Mean value theorems of the integral calculus, infinite integrals 109
Mean value theorems of the integral calculus, second theorem (ordinary integrals) 94
Modulus see “Absolute value”
Monotonic functions 66
Monotonic functions, admit only ordinary discontinuities 67
Monotonic functions, integrability of 84
Monotonic in the stricter sense, definition of 39
Moore 150
Neighbourhood of a point, definition of 52
Neumann 243 294
Newton 26
Numbers (Chapter I.), Dedekind’s theorem on the system of real 23
Numbers (Chapter I.), Dedekind’s theory of irrational 18
Numbers (Chapter I.), development of the system of real 25 (see also “Dedekind’s axiom of continuity” and “Dedekind’s sections”)
Numbers (Chapter I.), irrational 17
Numbers (Chapter I.), rational 16
Numbers (Chapter I.), real 21
Open interval, definition of 49
Ordinary or simple discontinuity, definition of 65
Oscillation of a function in an interval 50
Oscillation of a function in an interval of a function of two variables in a domain 72
Oscillatory, functions 52
Oscillatory, integrals 99
Oscillatory, sequences 38
Oscillatory, series 41
Osgood 45 58 75 119 129 163 193
Partial remainder 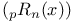 , definition of 123 , definition of 123
Partial remainder 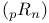 , definition of 42 , definition of 42
Periodogram analysis 299
Picard 14 234
Pierpont 75 83 86 93 110 119 163 193
Poincare 7
Points of infinite discontinuity, definition of 66
Points of oscillatory discontinuity, definition of 66
poisson 7 8 230 231 293
Poisson’s discussion of Fourier’s series 230
Poisson’s integral 231
Power series, Abel’s theorem on 146
Power series, integration and differentiation of 148
Power series, interval of convergence of 145
Power series, nature of convergence of 146
Pringsheim 16 25 28 34 48 75 163 287 291
Proper integrals, definition of 113
Raabe 165
Rational numbers and real numbers see “Numbers”
Remainder after n terms, (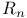 ), definition of 43 ), definition of 43
Remainder after n terms, (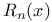 ) 123 ) 123
Repeated integrals, (infinite) 180 183 190
Repeated integrals, (ordinary) 172
Repeated limits 127
Riemann 5 9—11 15 77 112 243
Runge 268 297
Russell 26 28
Sachse 15 124
Schuster 301
Sections see “Dedekind’s sections”
Seidel 11 130
Sequences, convergent 33
Sequences, divergent and oscillatory 37
Sequences, limit of 33
Sequences, monotonic 39
Sequences, necessary and sufficient condition for convergence of (general principle of convergence) 34
Series with positive terms 43
Series, absolute and conditional convergence of 44
Series, convergent 41
Series, definition of sum of an infinite 41
Series, definition of sum, when terms are functions of a single variable 122
Series, divergent and oscillatory 41
Series, necessary and sufficient condition for convergence of 42
Series, necessary and sufficient condition for uniform convergence of 132
Series, term by term differentiation and integration of 140 (see also “Differentiation of series” “Fouriefs “Integration “Poiver
Series, uniform convergence and continuity of 135
Series, uniform convergence of 129
Series, Weierstrass’s M-test for uniform convergence of 134
Shearer see “Carse and Shearer”
Simple (or ordinary) discontinuity, definition of 65
Sine integral (Fourier’s integral) 284 292
Sine series (Fourier’s series) 197 220
Sommerfeld 293
Stekloff 14
Stokes 11 130
Stolz 119 163 193
Stolz and Gmeiner 16 28 48
Summable series (CI), definition of 151
Sums S and s, definition of 77
Tannery 28
Topler 14
Trigonometrical series 196
Turner 299
Uniform continuity of a function 62
uniform convergence of integrals 174
Uniform convergence of series 129
Upper integrals, definition of 81
Van Vleck 14
Watson see “Whittaker and Watson”
Weber — Riemann 243 294
Weierstrass 14 26 27 77 97 134
Weierstrass’s M-test for uniform convergence 134
|
|
 |
| Ðåêëàìà |
 |
|
|
 |
|
Î ïðîåêòå
|
|
Î ïðîåêòå



 -test for convergence of integrals
-test for convergence of integrals 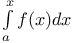 when f(x) is bounded and integrable
when f(x) is bounded and integrable 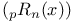 , definition of
, definition of 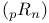 , definition of
, definition of 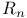 ), definition of
), definition of 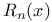 )
)