|
|
 |
| Авторизация |
|
|
 |
| Поиск по указателям |
|
 |
|
 |
|
|
 |
 |
|
 |
|
| Bergman S., Schiffer M. — Kernel Functions and Elliptic Differential Equations in Mathematical Physics |
|
|
 |
| Предметный указатель |
I(P, Q) 298
I(P, Q), assumption of semi-definiteness 302
Incompressible fluid flow 29 30 71
Induction coefficients 160
Induction coefficients, variation 194—195
Induction matrix 159 164—165
Induction matrix, definiteness 164—165
Integral equation, axially symmetric flow 124
Integral equation, fundamental theorem 265
Integral equation, kernel function 309
Integral equation, magnetostatics 170—177
Integral equation, plane strain 257
Integral operator, convergence proof 289—291
Integral operator, first kind 286 289
Integral operator, generating function 288
Integral operator, inversion formula 291
Integral operator, second kind 289
Irrotationality condition 28 72
Isotropic body 3
Isotropic material 213
Jeans, J. 157 160 178 188
Jeffreys, H., and Jeffreys, B.S. 7 2&
Joukowski's principle 91
Joukowski, N. 89 91
Julia, G. 98 26b 326
Kaczmarz, S., and Steinhaus, H. 286
Kantorovitch, L.V., and Kriloff, V. L 246
Karman, Th. v. 123 124
Kellogg, O.D. 7 8 34 41 188 263 311 331 332
Kelvin's transformation 34
Kelvin, Lord 19 34 180
Kernel function, complex 112—118 243—246
Kernel function, for  275—276 280 281 275—276 280 281
Kernel function, for biharmonic equation 240—241 249
Kernel function, for Laplace's equation 198—200 202
Kernel function, for unit circle 117
Kernel function, inequalities for 51 204 277 303 328 337 354 383 387 400
Kernel function, integral equation for 309
Kernel function, properties, asymptotic properties 118
Kernel function, properties, dependence on differential equation 387
Kernel function, properties, dependence on domain 337 354 362—364
Kernel function, properties, minimum property 277 279
Kernel function, properties, non-negativity 51 383
Kernel function, properties, reproducing property 116 201 240 244 249 275
Kernel function, properties, symmetry 115 240 244 249
Kernel function, properties, unit multiplier 201 275
Kernel function, relation to capacity 204
Kernel function, relation to Green's function 112
Kernel function, relation to mapping function 118
Kernel function, series development in geometric expressions 314—315 327—329 371
Kernel function, series development in geometric expressions, error term 355—357
Kernel function, use, in calculating mapping function 117—118
Kernel function, use, in construction of Green's and Neumann's functions 205—206 277
Kernel function, use, in interpolation problems 279
Kernel function, use, in solving boundary value problems 119—200 240 276
Kernel function, use, variational and comparison formulas 113 336 362—364
Kernel tensor field 226
Kibel, I.A. see Kochin Kibel and
kinetic energy see Energy
Kirchhoff, G. 65
Kneser, A. 268 273. 334
Kochin, N.E., Kibel, I.A., and Rose, N.B. 132
Kriloff, V.I. see Kantorovitch and Kriloff
Kutta — Joukowski formulas 89
Kutta, W.M. 89
l-kernel 303
l-kernel, definition extended 340
l-kernel, eigenvalues 315
l-kernel, regularity 303—8
l-kernel, series development 326
l-monogenic functions 133
Lamb, H. 28 32
Lame constants 214
Laplace — Poisson equation 8 155 178 243 264
Laplace's equation 4 30 73
Laplace's equation, axially symmetric case 119
Laplace's equation, generalization 4 21 261
Laplace's equation, singular solutions 7—8
Laplace's equation, spherical coordinates 181
Lauricella, G. 246
Lavrentieff, M.A. 98 100.
Lavrentieff, M.A., and Shabat, B.V. 98. 101
Lax, P.D. 381
Lehto, O. 381
Leray, J. 66
Levi-Civita, T. 65
Levy, P. 20 64 98 111
Lewy, H. see Garabedian Lewy and
Lichnerowicz, A. 326
Lichtenstein, L. 28 268 273 309 334
Lift, extremum problem 98
Lift, of arc, monotonic property 99
Lift, theory of 70 89 93
Lin, C. C 154
Linear operator, completely continuous 323
Linear operator, symmetric 323
Linear space  259 259
Linear space  260 260
Linear space  260 260
Linear space decomposition of 260 371—372
Liouville, J. 313
Lokki, O. 381
Love, A.E. H. 208 232
Lovitt, W.V. 265 268
M-kernel 313
Magnetic field, existence of solutions of transformer problem 174
Magnetic field, integral equation 170
Magnetic field, Neumann series solution 172
Magnetic field, transformer core 168
Magnetic permeability 166
Magnetostatic field 165
Magnetostatic field, general equation 166
Magnetostatic field, saltus condition 168
Magnetostatic potential 166
Mapping function 80 84
Mapping function, relation to complete orthonormal system 119
Mapping function, relation to complex kernel function 118
Mapping function, relative to boundary value problems 81 84
Mapping function, variational formulas 96—97
Mapping radius 80
Mapping radius, in terms of complete orthonormal system 119
Mapping radius, Poincare's inequality 105
Mapping radius, variational formulas 98
Mass forces 209
Maximum principle, for solutions of  261 261
Maximum problems, for solutions of partial differential equations 58
Maximum problems, for virtual mass 66
Maxwell, J.C. 159 160 178 232
Michell, J.H. 246
Mikhtin, S. 257 268
Milne-Thomson, L.M. 28 93 132
Minimum problems for solutions of partial differential equations 19 23 31 32 147 162 188 277 301
Minkowski inequality 260
Mises, R.V. 93; see also Frank and Mises
Mises, R.V., and Friedrichs, K. O. 28 132 154
Mises, R.V., and Schiffer, M. 141 147
Modulus of elasticity 247
Moment in fluid flow 89—90
Morera, G. 232
Multivalued velocity potential 76
Multivalued velocity potential, solution in hodograph plane 135
Murnaghan* F.D. 7. 268
Muskhelishvili, N.I. 236 246 257
Nadai, A. 236
Nehari, Z. 119
Neuber, H. 232
Neumann's function, definition 13 22 35 38 78
Neumann's function, for  , three dimensions 21 , three dimensions 21
Neumann's function, for 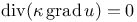 13 13
Neumann's function, for biharmonic equation 239
| Neumann's function, for Laplace's equation, three dimensions 35
Neumann's function, for Laplace's equation, two dimensions 78 263
Neumann's function, in the case of infinite domains 38—39
Neumann's function, in the case of unit circle 304
Neumann's function, inequalities for 22 49 50 52 360 382 383 386
Neumann's function, properties, dependence on differential equation 386
Neumann's function, properties, monotonicity 386
Neumann's function, properties, non-negativity 22 39 382
Neumann's function, properties, symmetry 14 22 36 39 78 263 271
Neumann's function, properties, unit multiplier 273—274
Neumann's function, series expansion 395
Neumann's function, series expansion at infinity 41—3
Neumann's function, two dimensions 271
Neumann's function, use in solving boundary value problems 15 22 30 36—38 41 126 239 271 334
Neumann's function, variational and comparison formulas 60 358 364 366
Neumann's series 172 313
Neumann's series, improvement of convergence 174
Neumann's tensor field 223
Neumann, C. 13 313
Neumann, J.V. 261 326
Non-linear partial differential equations 3 29
Non-linear partial differential equations, compressible fluid flow potential 129
Non-linear partial differential equations, potential of magnetic field 178
Non-linear partial differential equations, solution by successive approximation 178
Non-linear partial differential equations, variational equation 145
Normal functions 260
Ollendorff, F. 157
Orthogonal functions 260
Orthogonal functions, application of operators 355
Orthogonal functions, spherical harmonics 181
Orthogonal functions, Stekloff functions 397—398
Orthogonal harmonic functions 198
Orthogonal projection 372
Orthogonal subspaces 260
Orthonormal systems 116 202 226 241 243 249 280
Orthonormal systems of eigenfunctions 319 324 347—348 352
Orthonormal systems, construction of kernel function 116—117 249—250 281
Papkovitch, R.F. 232
Paradox of D'Alembert 88
Parseval's identity 323
Partial differential equations see Differential equations
Peres, J. 28; see also Volterra-Peres
Picard, E. 262
Planck, M. 208
Plane flows, boundary value problems 77 81 82
Plane flows, free boundaries 103
Plane flows, fundamental solutions 76—81
Plane flows, obstacles 82
Plane strain 246
Plane strain, boundary value problems 249 254
Plates, thin 232
Plemelj, J. 315 334
Poincare's inequality 105
Poincare, H. 15 333
Point source, in fluid flow 33 74
Point source, in heat flow 7 10
Poisson — Laplace equation 8 155 178 243 264
Poisson's formula 82
Poisson's ratio 214
Polarization see Electrostatic polarization
Poly A.G. 108
Potential function, electrostatic field 155
Potential function, fluid flows 29 74
Potential function, magnetostatic field 166
Potential function, reaction field 189—90
Potential-theoretic terms 49
Principal dilatations 208
Privaloff, J.J. 315
Pseudo-logarithmic plane 132
Rayleigh — Green identity 237
Reaction potential, grounded conductor 189
Reaction potential, isolated conductor 190
Regularity at infinity, harmonic functions 34
Regularity at infinity, solutions of 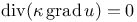 157 157
Reissner, E. 246
Reproducing kernel 397; see also Kernel function reproducing
Resolvent kernel 313
Riemann's function 269
Riemann's mapping theorem 80 84
Riemann, B. 19
Riemann, B., and Weber, H. 3 17 28
Robin's function, definition 11 272
Robin's function, for  , three dimensions 21 25 , three dimensions 21 25
Robin's function, for  , two dimensions 272 , two dimensions 272
Robin's function, for 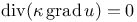 11 25 11 25
Robin's function, for Laplace's equation 268
Robin's function, inequalities for 13 22 382—383 388
Robin's function, properties, dependence on differential equation 388
Robin's function, properties, dependence on parameter  13 22 383 13 22 383
Robin's function, properties, nonnegativity 22 382
Robin's function, symmetry 11—12 272
Robin's function, unit multiplier 274
Robin's function, use in solving boundary value problems 12 272 346
Robin's tensor field 223
Rose, N.B. see Kochin Kibel and
Sauer, R. 132 147
Scalar product 259
Schauder, J. 66
Schiffer, M. 98 119 246 402; Lewy and
Schiffer, M., and Spencer, D.C. 315 346
Schiffer, M., and Szego, G. 43 64 188 193 206 301 360
Schmidt, E. 315
Schroder, K. 246
Schwarz inequality 55 107 260
Shabat, B.V. see Lavrentieff and Shabat
Shapiro, G.S. 232
Sherman, D.I. 257
Simply-supported edge 236
Singular points, of harmonic functions 33—35
Singular solutions, axially symmetric flow 121—122
Singular solutions, Laplace's equation, three dimensions 7 8 33 35
Singular solutions, Laplace's equation, two dimensions 74
Singular solutions, pseudo-logarithmic plane 136—137 147
Singularities at infinity 34—35
Sinks, at infinity 35 75
Sinks, fluid flow 32 151
Sinks, heat flow 4 8
Smirnoff, V. L 15 268 334
Smith, T.L. see Sternberg and Smith
Sokolnikoff, I.S. 208
Solenoidal field 31 56
Somigliana, C. 220
Sommerfeld, A. 3 10 132 208 273
Source strength 7 33 75
Sources, at infinity 34—35
Sources, fluid flow 32 74
Sources, heat flow 4 7
Specific heat 2
Spencer, D.C. see Garabedian and Spencer; Schiffer and Spencer
Spherical harmonics 181—184
Stability in wing theory 101
Stability of eigenvalues 388
Stability of fundamental functions 384
Stagnation points 92
Stationary fluid flow 28 30
Steady heat flow 3
Steinhaus, H. see Kaczmarz and Steinhaus
Stekloff's functions 391—398
Stekloff, W. 397 402
Sternberg, W., . and Smith, T.L. 188 263 334
Stokes' function 121
Stone, M.H. 261 326 355
Strain quadric 208
Strain tensor field 208
Stream function 73
Stream function, axially symmetric flow 121—122
Stream function, two-dimensional compressible flow 129
Stream lines 73
Stream lines, branching of 92
Stress 209
|
|
 |
| Реклама |
 |
|
|
 |
|
О проекте
|
|
О проекте