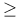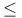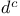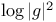|
|
 |
| Авторизация |
|
|
 |
| Поиск по указателям |
|
 |
|
 |
|
|
 |
 |
|
 |
|
| Cherry W., Ye Z. — Nevanlinna's Theory of Value Distribution: The Second Main Theorem and Its Error Terms |
|
|
 |
| Предметный указатель |
 32 32
Absolute value 29
Absolute value at infinity 29
Absolute value for meromorphic functions 30
Absolute value, Archimedean 29
Absolute value, Archimedean on a number field 152
Absolute value, equivalence between 29
Absolute value, non-Archimedean 29
Absolute value, non-Archimedean on a number field 153
Absolute value, p-adic 29 153
Absolute value, trivial 29
Ahlfors — Beurling Inequality 79
Ahlfors — Shimizu characteristic (or height) see “Characteristic function”
Ahlfors, L. vi 15 39 47 49 61 62 74 79
Ahlfors’s theory of covering surfaces 74
Algebraic integer 153
Algebraic number 151
Archimedean absolute value see “Absolute value Archimedean”
Artin — Whaples Product Formula 30 152 154 156
AV 1-3 29
AV 3' 29
Baker, I.N. 146
Bergweiler, W. 74 150
Beurling, A. 79
Bohr, H. 146
Bonk, M. 139 183
Borel — Nevanlinna growth lemma 98
Borel, E. v 98
Boundary term (in Stokes’s Theorem) 33
Bryuno, A. 159
Calculus lemma 52 53
Caratheodory, C. 142
Carleman, T. 80
Carlson, J. 31
Cartan, H. 3 43 107
Cartan’s Theorem equating height or characteristic with the average of the counting function 43
Cauchy — Riemann equations 31
Characteristic function 2
Characteristic function, expressed as average of counting function 43 45
Characteristic function, expressed as height transform 57
Characteristic function, geometric (Ahlfors — Shimizu) 17 39
Characteristic function, invariance under linear fractional transformation 23
Characteristic function, Nevanlinna 17
Chem class 32
Chem operator see “First Chem operator”
Chem, S.S. vi 61 62
Chen, H. 117 172 173
Cherry, W. 139
Chordal distance 15
Compensation function see “Mean proximity function”
Complex conjugate ix
Concave 47
Concavity of the logarithm 48
Conjugate, complex see “Complex conjugate”
Constants x
Convergence class 27
Convex 41
Convexity of  (in log r) 41 (in log r) 41
Convexity of T(f, r) (in log r) 44
Cosine, Nevanlinna functions for 27
Cosine, order of 28
Counting function 1 12
Counting function, associated to ramification divisor 22 125
Counting function, number theoretic analogue of 156
Counting function, truncated 13 125
Counting function, unintegrated 12
Counting function, unintegrated, compared to A 42
Counting function, upper bound on 20
Curvature 37 60
Curvature computation 37 61
Dcvaney, M. 159
Defect 124
Defect, ramification 125
Defect, truncated 125
Deficient 125
Density, upper 171
Differential equations 129
Dufresnoy, J. 76
Eremenko, A. 3
Error term vi 3 60
Euclidean form 36
Euler’s constant 180
Euler’s gamma function see “Gamma function”
Exceptional set 3 50
Exponential function 5 20
Exponential function, Lang type of 180
Exponential function, Nevanlinna functions for 25
Exponential function, order of 27
Exterior derivative 31
Finite order see “Order finite”
Finite order, Second Main Theorem for functions of 117
First Chem operator 32
First Main Theorem 2 5 17
First Main Theorem constant 17
First Main Theorem, equivalent to Jensen’s Formula 19
First Main Theorem, unintegrated 42
Fixed point 146
Fixed point of order n 146
Frank, G. 136
Fubini — Study form 35
Fubini’s Theorem 33
Fundamental Theorem of Algebra, v, I 11 19 42 120
Gamnui function 180
Gamnui function, derivative of 180
Gamnui function, Lang type of 185
Gauss — Bonnet formula 74
GJ 1-4 34
Goldberg Grinshtein inequality 3 92 96 98
Goldberg, A.A. v 3 42 89 92 96 98 107
Green — Jensen Formula 33
Greens function, relation to Poisson kernel 83
Green’s function 83
Griffiths, P. 31
Grinshtein, A. 3 89 92 96 98 107
Growth lemma 52 53
Growth order see “Order growth”
Growth type see “Type growth”
Hadamard, J. v
Harmonic 31
Harmonic on closed disc 6
Hayman — Stewart Theorem 43
Hayman, W. v 3 42 107 146 147
Height function 2
Height function, expressed as average of counting function 43 45
Height function, geometric (Ahlfors — Shimizu) 17 39
Height function, invariance under linear fractional transformation 23
Height function, Nevanlinna 17
Height transform 54
Height transform, Ahlfors — Shimizu characteristic expressed as 57
Height transform, fundamental estimate for 54
Height transform, second derivative expressed as integral on circle 54
Height, absolute logarithmic 155
Height, number theoretic 154 155
Hempel, J. 142
Hinkkanen, A. 107 110 162 163
Holomorphic on closed disc 6
HT 1-3 53
Huber, A. 78
Imaginary part ix
Infinite order see “Order infinite”
Infinite order, example 28
Initial Laurent coefficient 9
Integers, in a number field 153
Interior term (in Stokes’s Theorem) 33
Isoperimctric inequality, planar 79
Isoperimctric inequality, spherical 81
Jank, G. vi 3
Jankowski, M. 171
Jenkins, J. 142
Jensen formula 11
| Jensen, J. 9
Jensen’s Inequality 48
Khinchin convergence condition 49 159
Khinchin function 49 159
Khinchin, A. 159
Khinchin’s Theorem 159 177
Kolokolnikov, A. 90
Kolokolnikov’s inequality 90
Laguerre’s Theorem 186
Laine, I. vi
Landau type theorem see “Theorem of Landau type”
Landau, E. 141
Lang type 177
Lang type of exponential function 180
Lang type of gamnui function 185
Lang type of rational function 178
Lang type of trigonometric functions 180
Lang type, greater than or equal 178
Lang type, Lang type 1 178
Lang type, less than or equal 177
Lang type, precise 178
Lang, S. vi 3 50 53 60 61 151 159 177
Lemma on the Logarithmic Derivative 89 104
Lemma on the Logarithmic Derivative, sharpness of 161
Li, P. 136
Logarithmic derivative 89
Logarithmic measure 50
Lower order see “Order growth lower”
LT 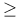 178 178
LT 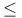 177 177
Malmquist, J. 130
Malmquist’s theorem 129
Maximal type 27
Maximum modulus function 28
Mean proximity function, difference between Nevanlinna and geometric 15
Mean proximity function, geometric 15
Mean proximity function, Nevanlinna 14
Mean proximity function, number theoretic analog of 155
Mean proximity function, sometimes called “compensation” function 20
Mean type 27
Mean-proximity function 2
Meromorphic on closed disc 6
Metropolis, N. 159
Miles, J. 42 43 89 162
Minimal type 27
Monic polynomial 153
Moving targets 144
Mues, E. 136
Nevanlinna characteristic function see “Characteristic function”
Nevanlinna defect see “Defect”
Nevanlinna functions 17
Nevanlinna functions for exponential function 25
Nevanlinna functions for rational functions 24 121
Nevanlinna functions for sine and cosine 27
Nevanlinna functions for sums and products 23
Nevanlinna functions, how they are related on average 43
Nevanlinna growth lemma 52 53
Nevanlinna Inverse Problem 127
Nevanlinna theory 1 17
Nevanlinna, F. vi 2 3 49 61
Nevanlinna, R. v vi 2—4 14 49 53 61 89 98 107 127 129 135 144
Ngoan, V. 106
Non-archimedean see “Absolute value non-Archimedean”
Number field 30
Number theoretic digression 4 29
Numbering conventions x
o notation ix
Order of a zero or pole 9
Order, finite 27
Order, growth 27
Order, growth, lower 27
Order, infinite 27
Osgood, C.F. vi 30 144 151
Ostrovskii, I, V. v 3 106
Ostrowski, A. 29
p-adic absolute value see “Absolute value p-adic”
Picard, E. v 128
Picard’s Theorem 20 128
Picard’s Theorem, number theory analogue of 158
Poincane, H. 31
Poisson formula 7
Poisson kernel 6
Poisson kernel, harmonic 6
Poisson kernel, relation to Green’s function 83
Poisson kernel, transofmation under Mobius automorphism 6
Poisson — Jensen formula 9
Poisson, S. 7
Polar coordinate form of 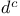 31 31
Polya — Laguerre class 186
Product formula 29
Product into sum 16 67
Projective line 6
Proximity function see “Mean proximity function”
Pseudo area form 37
Pseudo volume (area) form 59
Pull back 32
Radius bound 138
Ramification 20
Ramification for polynomials and rational functions 21
Ramification Theorem 58 71 111
Ramification Theorem, coefficient 1/2 best possible 60
Ramification Theorem, open question about improving error term 72 112
Ramification, alternative to Wronskian 22
Ramification, counting function see “Counting function associated to ramification divisor”
Ramification, defect 125
Ramification, divisor 21
Ramification, index 21
Ramification, point 20
Ramification, term see “Counting function asssociated to ramification divisor”
Rational function, Lang type of 178
Rational functions, Nevanlinna functions for 24 121
Real part ix
Reinders, M. 136
Riccati equation 130
Richtmyer, R. 159
Riemann sphere 6
Riemann zeta function 186
Riemann — Hurwitz Theorem 74
Ring of integers 153
Roth, K. 151
Roths Theorem 151 154 156 158
Roths Theorem, conjectural improvement of 159
Ru, M. 144
Schottky type theorem see “Theorem of Schottky type”
Schottky, F. 142 143
Second Main Theorem vi 2 60 72 77 108
Second Main Theorem for functions of finite order 117
Second Main Theorem for moving targets 144
Second Main Theorem, sharpness of 161
Second Main Theorem, uniform 77
Second Main Theorem, via curvature 60 72
Second Main Theorem, via logarithmic derivatives 108
Sharpness of the Lemma on the Logarithmic Derivative 161
Sharpness of the Second Main Theorem 161
Shimizu, T. vi 15 39
Sine, Nevanlinna functions for 27
Sine, order of 28
Singular term (in Stokes’s Theorem) 33
Singular term (in Stokes’s Theorem)contribution from 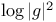 37 37
Singular volume (area) form 62
Slowly moving 144
Small function 144
Smirnov, V. 89 90
Smirnov’s inequality 90
Soas, L. 177 183 186
Sodin, M. 3
Solution to the Dirichlet Problem 8
Spherical area form 35
Spherical derivative 37
Steinmetz, N. 144
|
|
 |
| Реклама |
 |
|
|
 |
|
О проекте
|
|
О проекте




 (in log r)
(in log r)