Авторизация
Поиск по указателям
Boyd J.P. — Chebyshev and Fourier Spectral Methods
Обсудите книгу на научном форуме Нашли опечатку?
Название: Chebyshev and Fourier Spectral MethodsАвтор: Boyd J.P. Аннотация: Completely revised text focuses on use of spectral methods to solve boundary value, eigenvalue, and time-dependent problems, but also covers Hermite, Laguerre, rational Chebyshev, sinc, and spherical harmonic functions, as well as cardinal functions, linear eigenvalue problems, matrix-solving methods, coordinate transformations, methods for unbounded intervals, spherical and cylindrical geometry, and much more. 7 Appendices. Glossary. Bibliography. Index. Over 160 text figures.
Язык: Рубрика: Математика /Анализ /Продвинутый анализ /Статус предметного указателя: Готов указатель с номерами страниц ed2k: ed2k stats Год издания: 2000Количество страниц: 665Добавлена в каталог: 02.04.2005Операции: Положить на полку |
Скопировать ссылку для форума | Скопировать ID
Предметный указатель
Polar coordinates, Bibliography Table: annular domains 391 Polar coordinates, Bibliography Table:Unbounded Domain or External to Cylinder 390 Polar coordinates, boundary conditions in 383 Polar coordinates, One-Sided Jacobi polynomial basis for 387 Polar coordinates, parity in radius 383—385 Polar coordinates, radial basis sets and grids 385—390 Polar coordinates, spectral methods in 381—390 Polar coordinates, unbounded domain 390 Power Series with Definite Parity 161 Preconditioning (of iteration) as easy way to raise finite differences to spectral accuracy 301 Preconditioning (of iteration) by finite difference matrix 293—297 Preconditioning (of iteration) by finite element matrices 301 Preconditioning (of iteration) by incomplete LU factorizations of finite difference matrix 299 Preconditioning (of iteration) by small block-and-diagonal Galerkin matrix 307 Preconditioning (of iteration) for nonlinear problems 321 Pseudoarclength continuation 546—549 Pseudospectral method, boundary conditions see boundary conditions Pseudospectral method, checking by decrease of coefficients 123 Pseudospectral method, checking by varying trunction N 123 Pseudospectral method, checking through finite difference residual 121 Pseudospectral method, choice of basis functions 109 Pseudospectral method, choice of interpolation grid 116 Pseudospectral method, common mistakes 155 Pseudospectral method, comparisons with finite differences see finite difference methods with Pseudospectral method, defined 62 Pseudospectral method, derivatives, computing 116 Pseudospectral method, Fast Multipole Method (FMM), and 196 Pseudospectral method, halving grid and basis due to parity 165 Pseudospectral method, inferior to Galerkin for constant coefficient ODEs 313 Pseudospectral method, slow manifold: Chebyshev differentiation matrix 236—238 Pseudospectral method, special difficulties of high order derivatives 142 Pseudospectral method, zero phase and amplitude errors in Fourier basis 224 Quadrature,spectrally-accurate of periodic integrands 457 Quadrature,spectrally-accurate of singular integrands 459 Quadrature,spectrally-accurate, Clenshaw — Curtis adaptive 455 Quadrature,spectrally-accurate, Gaussian (Gauss — Jacobi) Theorem 87 Quadrature,spectrally-accurate, mechanics of non-Gaussian 456 Quadrature,spectrally-accurate, non-Gaussian infinite interval 456 458 Quasi-geostrophic Eq., time-marching 181 Quasi-Sinusoidal Rule-of-Thumb 54 Rational Chebyshev functions SB 365 Rational Chebyshev functions TB 356—361 Rational Chebyshev functions TB, Bibliography Table 357 Rational Chebyshev functions TB, collected identities 507 Rational Chebyshev functions TB, eigenvalue example 131 Rational Chebyshev functions TB, expansions of functions that decay algebraically at infinity 363—366 Rational Chebyshev functions TB, numerical examples 366—368 Rational Chebyshev functions TB, Table of derivative-computing formulas 555 Rational Chebyshev functions TB, Table of Explict Basis Functions 358 Rational Chebyshev functions TL 327 369—370 Rational Chebyshev functions TL, collected identities 509 Rational Chebyshev functions TL, numerical examples 370—372 Rational Chebyshev functions TL, Table of derivative-computing formulas 557 Rational Chebyshev functions TL, Table of Explict Basis Functions 369 Regularized Long Wave (RLW) Eq., time-marching 181 Richardson extrapolation 261 Richardson iteration see iteration Richardson defined Robert functions (spherical basis) 434 Root-finding by Chebyshev algorithms 450—452 Rule-of-Thumb, Assumption of Equal Errors 32 Rule-of-Thumb, Behavioral Boundary Conditions at Infinity 362 Rule-of-Thumb, Boundary Layer Resolution Requirement 59 Rule-of-Thumb, CFL Stability Limit: Physics 173 Rule-of-Thumb, Dealiasing/Energy-Conserving 215 Rule-of-Thumb, Eigenvalue 132 Rule-of-Thumb, Explicit-Demanding 230 Rule-of-Thumb, Implicit Scheme Forces Physics Slowdown 230 Rule-of-Thumb, Last Coefficient Error Estimate 51 Rule-of-Thumb, Optimizing Infinite Interval Map Parameter 377 Rule-of-Thumb, Penalties of Unbounded Interval 338 Rule-of-Thumb, Quasi-Sinusoidal Resolution Requirement 55 Rule-of-Thumb, Teller’s Law 54 Rule-of-Thumb, Two-Thirds Rule (for dealiasing) 212 Rule-of-Thumb, Witch-of-Agnesi Resolution Estimate 57 Runge phenomenon see interpolation divergence Sawtooth function 21 Scattering of waves, special basis functions for 448—450 Semi-implicit see time-marching semi-implicit Semi-infinite interval see unbounded domain Semi-Lagrangian (SL) time-marching, accuracy improves with increasing time step 279 Semi-Lagrangian (SL) time-marching, advantages and disadvantages 271 Semi-Lagrangian (SL) time-marching, Bibliography Table 287 Semi-Lagrangian (SL) time-marching, computational diffusion of 281 Semi-Lagrangian (SL) time-marching, iteration for departure points 275 Semi-Lagrangian (SL) time-marching, methods of characteristics and 272 Semi-Lagrangian (SL) time-marching, noninterpolating variants 281 Semi-Lagrangian (SL) time-marching, off-grid interpolation for 283 Semi-Lagrangian (SL) time-marching, three-level SI scheme 273 Semi-Lagrangian (SL) time-marching, two-level SI scheme 280 Separable PDEs, Haidvogel — Zang direct method 314 Shamrock Principle 178 Sideband truncation 443—446 Sinc function as infinite interval basis 341—346 Sinc function, Bibliography Table 345 Sinc function, connection with trigonometric interpolation 102 Sinc function, defined 99 Sinc function, derivative formulas 569 Sinc function, expansions in 343—344 Singular basis functions 330 Skew-symmetric advection 213 Slow manifold, defined 232 Slow manifold, forced linear oscillator 233 Slow manifold, initialization onto 239—243 Slow manifold, Korteweg — deVries equation 234 Slow manifold, Lorenz — Krishnamurthy Quintet 233 Slow manifold, multiple scale perturbation theory and 241 Slow manifold, numerically-induced 236 Slow manifold, steady-state (trivial slow manifold) 233 Slow manifold, three-part strategy 249 Slow manifold, tracking with implicit scheme 248 Slow manifold, weather forecasting 231—232 Spectral blocking, defined 207 Spectral blocking, delayed blow-up 210 Spectral blocking, frontogenesis and 217 Spectral blocking, linear example 209 Spectral blocking, remedies for 218 Spectral coefficients, computation by matrix multiplication see Matrix Multiplication Transform(MMT) Spectral coefficients, integral for 305 Spectral convergence, defined 25 Spectral elements, Bibliography Table:Surface of Sphere 438 Spectral elements, cardinal basis (only) gives diagonal mass matrix 485 Spectral elements, choice of basis 486 Spectral elements, choice of grid 486 Spectral elements, defined 479 Spectral elements, degree of inter-element continuity 485 Spectral elements, influence matrix method 488—491 Spectral elements, matrix inversion 487 Spectral elements, patching versus variational formalism 486 Spectral elements, sectorial elements 492 Spectral elements, spherical coordinates 437 Spectral elements, two-dimensional maps 491 Spectral elements, variational formalism 484—485 Spectral elements, weak element-to-element coupling 481—484 Spherical coordinates, Bibliography Table: Gridpoint Methods 432 Spherical coordinates, Bibliography Table: Legendre transforms 408 Spherical coordinates, Bibliography Table: spectral elements 438 Spherical coordinates, Bibliography Table: variable resolution 410 Spherical coordinates, Glatzmaier stellar/mantle convection model 430 Spherical coordinates, mapping of sphere into a sphere 409 Spherical coordinates, non-tensor and icosahedral grids 432 Spherical coordinates, parity factor 393—397 Spherical coordinates, parity-modified Fourier series in latitude 434 Spherical coordinates, radial coordinate basis & grid 429 Spherical coordinates, resolution and unresolved scales 425—427 Spherical coordinates, Robert basis functions 434 Spherical coordinates, slow Legendre transforms in latitude 402—407 Spherical coordinates, spectral elements 437 Spherical coordinates, spherical harmonics see spherical harmonics Spherical coordinates, variable resolution (limited-area) models 409 Spherical coordinates, vector basis functions 428 Spherical coordinates, “pole problem” (severe CFL limit) 398 Spherical harmonics, Addition Theorem/Group Property 408 Spherical harmonics, alternatives to spherical harmonics 433 Spherical harmonics, asymptotic approximations near equator 412 Spherical harmonics, asymptotic approximations near poles 411 Spherical harmonics, Bibliography Table: Alternatives to Spherical Harmonics 435 Spherical harmonics, Bibliography Table: comparisons with finite differences 438 Spherical harmonics, Bibliography Table: Reviews & Model Descriptions 441 Spherical harmonics, comparisons with finite differences 438 Spherical harmonics, defined 399 Spherical harmonics, equiareal resolution property 409 Spherical harmonics, PMMT in latitude 404 Spherical harmonics, reduced grid (near-pole deletions) 405 Spherical harmonics, shallow water wave algorithm 416 Spherical harmonics, software and libraries 414 Spherical harmonics, triangular truncation table 401 Spherical harmonics, triangular truncation, defined 400 Spherical harmonics, triangular versus rectangular truncation 388 Spherical projective filter, Bibliography Table: Projective Filters 437 Splitting see time-marching Sponge layer 341 Subgeometric convergence, defined 26 Subgeometric convergence, examples, Fourier series 23 Supergeometric convergence, defined 26 Symbolic manipulation language and spectral methods 95 114 461—472 Symbolic manipulation language example 2 Symbolic manipulation language: Table of Precepts 463 Symmetry, halving grid and basis due to parity 165 Symmetry, multi-dimensional 166 Symmetry, parity 159—165 Symmetry, rotation and dihedral groups 165 Tau-method, Bibliography Table 477 Tau-method, canonical polynomials 478 Tau-method, defined 473 Tau-method, for approximating a rational function 474 Tau-method, linear differential equation 476 Taylor — Green vortex 166 Theorems, Asymptotic Equality of Coefficients if Singularities Match 32 Theorems, Cauchy Interpolation Error 85 Theorems, Chebyshev Asymptotic Rate of Convergence 49 Theorems, Chebyshev Ellipse-of-Convergence 48 Theorems, Chebyshev Interpolation and its Error Bound 95 Theorems, Chebyshev Minimal Amplitude 85 Theorems, Chebyshev Truncation 47 Theorems, Convergence Domain in Complex Plane 35 Theorems, Darboux’s Principle:Singularities Control Convergence 32 Theorems, Differentiation and Parity 162 Theorems, Elliptical Coordinates: Parity in Quasi-Radial Coordinate 440 Theorems, Fourier Interpolation Error 94 Theorems, Fourier Truncation Error Bound 50 Theorems, Gaussian Quadrature (Gauss — Jacobi Integration) 87 Theorems, Hermite Rate-of-Convergence 350 Theorems, Hille’s Hermite Width-of-Convergence 350 Theorems, Inner Product for Spectral Coefficients 66 Theorems, Integration-by-Parts Coefficient Bound 42 Theorems, Interpolation by Quadrature 92 Theorems, Legendre Rate of Convergence 52 Theorems, LU Decomposition of a Banded Matrix 518 Theorems, Matrices Whose Elements Are Matrices 520 Theorems, Matrices Whose Elements Depend on a Parameter 466 Theorems, Mean-Square Minimization with a Truncated Series 305 Theorems, Orthogonality under the Discrete Inner Product 90 Theorems, Parity Decomposition 163 Theorems, Parity Matrix Multiplication Transform (PMMT) 190 Theorems, Parity of Basis Functions 160 Theorems, Parity of the Powers of x 161 Theorems, Polar Coordinates: Parity in Radius 383 Theorems, Shannon-Whittaker Sampling Theorem 343 Theorems, Singularities of the Solution to a Linear ODE 36 Theorems, Strip of Convergence, Fourier Series 45 Theorems, Symmetry Properties of an ODE 164 Theorems, Trapezoidal Rule Error for Periodic Integrands 457 Theorems, Trigonometric Interpolation 93 Three-Halves Rule see Two-Thirds Rule Time-dependent problems 15 16 Time-marching, (Adams — Moulton 2d order) implicit scheme see time-marching Crank Time-marching, A-stable property 229 Time-marching, Adams — Bashforth scheme, 3rd order (AB3) 173 Time-marching, Adams — Bashforth, 2d order (AB2) 174 Time-marching, Adams — Bashforth/Crank — Nicholson (AB3CN) semi-implicit scheme 229 Time-marching, Adams — Moulton 1st order implicit scheme see time-marching Backwards Time-marching, adaptive Runge — Kutta (RK45) 174 Time-marching, Alternating-Direction Implicit (ADI) 261 Time-marching, Backward Differentiation (BDF) implicit schemes 228 Time-marching, Backwards Euler (BE) implicit scheme 228 Time-marching, Bibliography Table: Fourier basis, multidimensional 181 Time-marching, Bibliography Table: Fourier basis, one-dimensional 180 Time-marching, consistency of schemes 258 Time-marching, Crank — Nicholson (CN) implicit scheme 228 Time-marching, Crank — Nicholson, fourth order 260 Time-marching, Explicit-Demanding Rule-of-Thumb 231 Time-marching, hybrid grid point/Galerkin algorithm 176 Time-marching, Implicit Scheme Forces Physics Slowdown Rule-of-Thumb 230 Time-marching, implicit schemes 228—229 Time-marching, implicitly-implicit problems 181 Time-marching, KdV Eq. example (Fourier basis) 179 Time-marching, leapfrog 174 Time-marching, operator theory of 259 Time-marching, Runge — Kutta, 4th order (RK4) 173 Time-marching, semi-implicit, defined 229 Time-marching, semi-Lagrangian (SL) see semi-Lagrangian Time-marching, splitting for diffusion 255—256 Time-marching, splitting for fluid mechanics 263 Time-marching, splitting, basic theory 252—254 Time-marching, splitting, boundary difficulties 256 Time-marching, splitting, high order for noncommuting operators 262 Time-marching, trapezoidal implicit scheme see time-marching Crank Toroidal coordinates, basis functions for 392 Toroidal coordinates, defined 381 Toroidal coordinates, illustrated 391 Transfinite interpolation 114 transform 190 Transforms (grid/spectral & inverse) to points off the grid 198—199 Transforms (grid/spectral & inverse), Bibliography Table 196 Transforms (grid/spectral & inverse), Fast Fourier Transform (FFT) see Fast Fourier Transform Transforms (grid/spectral & inverse), Generalized FFTs 195—198 Transforms (grid/spectral & inverse), Matrix Multiplication Transform (MMT) see Matrix Multiplication Transforms (grid/spectral & inverse), partial summation 184—187 Triangular truncation see spherical harmonics triangular Trigonometric interpolation 93 Trigonometric interpolation, cardinal functions 101—104 Trigonometric interpolation, infinite series for interpolant coefficients 93 Trigonometric interpolation, solving differential equations (BVP) 103 Truncation error, defined 31 Two-h waves 206 Two-Thirds Rule see aliasing instability Rule Two-Thirds Rule, preconditioning odd derivatives 296 Unbounded domain, behavioral versus numerical boundary conditions 361—363 Unbounded domain, comparison of logarithmic, algebra and exponential maps 355 Unbounded domain, domain truncation 326 339—340 Unbounded domain, functions that decay algebraically at infinity 363—366 Unbounded domain, functions with non-decaying oscillations 372—374 Unbounded domain, Hermite basis 346—353 Unbounded domain, need to pick scaling or map parameter 338 348 369 378 Unbounded domain, rational Chebyshev 356—361 Unbounded domain, rational Chebyshev 369 Unbounded domain, sinc basis 341—346 Unbounded domain, Weideman — Cloot map 374—377 van der Pol equation 532—534 Weak form of a differential equation 68 Weakly nonlocal solitary waves, special basis functions for 450 Weather forecasting, numerical, Lorenz — Krishnamurthy Quintet 233 Weather forecasting, numerical, multiple scales perturbation & iteration 243 Weather forecasting, numerical, slow manifold 231—232
Реклама
 |
|
О проекте
|
|
О проекте



![$(y \in[\gets \infty, \infty])$](/math_tex/408186070e366aec84fc68c2bc3bec2182.gif)
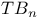
![$(y \in [\gets \infty, \infty])$](/math_tex/f38f63fb1de48e0a7728d63647530a4b82.gif)

![$(y \in [0, \infty])$](/math_tex/bf9544d192b147218e52f2e4139cad1682.gif)