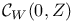Авторизация
Поиск по указателям
Koosis P. — The Logarithmic Integral (Vol. 1)
Обсудите книгу на научном форуме Нашли опечатку?
Название: The Logarithmic Integral (Vol. 1)Автор: Koosis P. Аннотация: The theme of this unique work, the logarithmic integral, lies athwart much of twentieth century analysis. It is a thread connecting many apparently separate parts of the subject, and so is a natural point at which to begin a serious study of real and complex analysis. Professor Koosis' aim is to show how, from simple ideas, one can build up an investigation which explains and clarifies many different, seemingly unrelated problems; to show, in effect, how mathematics grows.This, the first of two volumes, is self-contained, but more importantly, by following the theme, Professor Koosis has produced a work that can be read as a whole. He has brought together here many results, some unpublished, some new, and some available only in inaccessible journals.
Язык: Рубрика: Математика /Анализ /Продвинутый анализ /Статус предметного указателя: Готов указатель с номерами страниц ed2k: ed2k stats Год издания: 1997Количество страниц: 606Добавлена в каталог: 02.04.2005Операции: Положить на полку |
Скопировать ссылку для форума | Скопировать ID
Предметный указатель
41 Akhiezer’s description of entire functions, arising in weighted approximation 160 174 Akhiezer’s theorems about weighted polynomial approximation 158ff 424 523 Akhiezer’s theorems on weighted approximation by sums of imaginary exponentials 174 424 432 445 Approximation index 293 Approximation index M(A), Beurling’s 275 Approximation, weighted 145ff 385 424 Benedicks, M. 434ff Benedicks’ lemma on harmonic measure for slit regions bounded by a circle 400 Benedicks’ theorem on existence of a Phragmen — Lindelof function 418 431 Benedicks’ theorem on harmonic measure for slit regions 404 Bernstein approximation problem 146ff Bernstein intervals associated with a set of points on (0, oo) 454ff Bernstein’s lemma 102 Bernstein’s theorem on weighted polynomial approximation 169 Beurling quasianalyticity 275ff Beurling quasianalyticity for 292ff Beurling — Dynkin theorem on the Legendre transform 333 Beurling, A. And Malliavin, P. 550 568 Beurling’s approximation indices see under “Approximation index” Beurling’s gap theorem 237 305 Beurling’s identity for certain bilinear forms 484 Beurling’s theorem about Fourier — Stieltjes transforms vanishing on a set of positive measure 268 Beurling’s theorem about his quasi-analyticity 276 Beurling’s theorem on his Lp quasi-analyticity 293 Boundary values, non-tangential 10 43ff 265 269 286ff Canonical product 21 Carleman’s criterion for quasianalyticity 80 Carleman’s criterion for quasianalyticity, its necessity 89 Carleman’s inequality 96 Carleson’s lemma on linear forms 392 398 Carleson’s theorem on harmonic measure for slit regions 394 404 430 Cartan — Gorny theorem 104 Cauchy principal value, definition of 533 Cauchy transform, planar 320ff Class 79 Class 80 Convex logarithmic regularization 83ff 92ff 104ff 130 226 de Branges’ lemma 187 de Branges’ theorem 192 Density, of a measurable sequence 178 Dirichlet integral 479 500 510ff Dirichlet problem 251 360 387 388 Discussion about 198ff Dynkin’s extension theorem 339 359 373 Energy of a measure on 479ff 549ff 562 568 Energy of a measure on 482 487 494ff 508 512ff 551 552 553 563 566 Energy of a measure on 479 485 497 512 Energy of a measure on 482 493 Entire functions of exponential type 15ff Entire functions of exponential type as majorants on subsets of R 555ff 562 564 568 Entire functions of exponential type, arising in weighted approximation 160 174 218 219 525 Entire functions of exponential type, expansions 203ff 205 Exponential type, entire functions of see “Entire functions of exponential type expansions” Extension of domain, principle of 259 289 368 372 529 531 Extension of positive linear functionals 111ff 116 Extreme point of a convex 186ff Fejer and Riesz, lemma of 281 Function of exponential type, entire 15ff (see also under “Entire functions”) Function T{r) used in study of quasianalyticity 80ff Gap theorem, Beurling’s 237 Gauss quadrature formula 134 137ff Green potential 479 551 552 553 560 562 563 566 Green, George, homage to 419-22 Green’s function 400ff 406 407 410 418ff 439 479 526ff 547ff 550 Green’s function, estimates for in slit regions 401 439 442 548 Green’s function, symmetry of 401 415 418ff 530 Hadamard factorization for entire functions of exponential type 16 19 22 54 56 70 201 556 561 Hall of mirrors argument 157 158 184 208 375 523 Hall, T., his theorem on weighted polynomial approximation 169 Hankel matrix 117 Harmonic conjugate 46 59 61 Harmonic conjugate, existence a.e. Of 47 532 537 Harmonic estimation, statement of theorem on it 256 Harmonic functions, positive, representations for, in half plane 41 Harmonic functions, positive, representations for, in unit disk 39 Harmonic measure 251ff Harmonic measure in curvilinear strips, use of estimate for 355 Harmonic measure in slit regions 385 389ff 394 403 404 430 437 443 444 446 522 525ff 530 541 545ff 554 562 565 Harmonic measure, approximate identity property of 253 261 Harmonic measure, boundary behaviour of 261ff 265 Harmonic measure, definition of 255 Harmonic measure, Volberg’s theorem on 349 353 362 364 366 Harnack’s inequality 254 372 410 430 Hilbert transform 47 61 62 63 65 532 534 538ff Jensen’s formula 2 4 7 21 76 163 291 559 Kargaev’s example on Beurling’s gap theorem 305ff 315 Kolmogorov’s theorem on the harmonic conjugate 62ff Krein — Milman theorem, its use 186 199 Krein’s theorem on certain entire functions 205 Kronecker’s lemma 119 Legendre transform 323ff Levinson (and Cartwright), theorem on distribution of zeros for functions with real zeros only 66 Levinson (and Cartwright), theorem on distribution of zeros, general form of 69 Levinson (and Cartwright), theorem on distribution of zeros, use of 175 178 Levinson’s log log theorem 374ff 376 379ff Levinson’s theorem about Fourier — Stieltjes transforms vanishing on an interval 248 347 361 Levinson’s theorem on weighted approximation by sums of imaginary exponentials 243 Lindelof’s theorem on conformal mapping 264 Lindelof’s theorems about the zeros of entire functions of exponential type, statements 20 21 Log log theorem see under “Levinson” Lower polynomial regularization 158 Lower regularization 175 428 Lower regularization 236 Lower regularization 242 Lower regularizations 428 Markov — Riesz — Pollard trick 139 155 171 182 190 Maximum principle, extended, its statement 23 Measurable sequence 178 Mergelian’s theorems about weighted, polynomial approximation 147ff Mergelian’s theorems on weighted approximation by sums of imaginary exponentials 173 174 432 Moment problem see under “Riesz” Moment sequences, definition of 109 Moment sequences, determinacy of 109 126 128 129 131 141 143 Moment sequences, indeterminacy of 109 128 133 143 Moment sequences, Riesz’ characterization of 110 Moment sequences, same in terms of determinants 121 Newton polygon 83ff Non-tangential limit 11 Paley and Wiener, their construction of certain entire functions 100 Paley and Wiener, theorem of 31 Paley and Wiener, theorem of, 36 Phragmen — Lindelof argument 25 405 406 553 Phragmen — Lindelof function 25 386 406 407 418 431 441 525ff 541 555 Phragmen — Lindelof theorems, fifth 29 Phragmen — Lindelof theorems, first 23 Phragmen — Lindelof theorems, fourth 28 Phragmen — Lindelof theorems, second 25 Phragmen — Lindelof theorems, third 27 Poisson kernel for half plane 38 42 384 534 536 539 Poisson kernel for rectangle 299 Poisson kernel for unit disk 7 8 10ff Poisson kernel, pointwise approximate identity property of latter 10 Pollard’s theorem 164 433 Pollard’s theorem for weighted approximation by sums of imaginary exponentials 181 428 Polya maximum density for a positive increasing sequence 176ff Polya’s theorem 178 Quasianalytic classes 91 Quasianalyticity of a class 80 Quasianalyticity of a class 80 Quasianalyticity of a class 89 Quasianalyticity, Beurling’s 275ff Representations for positive harmonic functions see under “Harmonic functions” Riesz — Fejer theorem 55 556 Riesz, F. and M. 259 276 286 Riesz’ criterion for existence of a solution to moment problem 110 121 Riesz’ criterion for indeterminacy of the moment problem 133 Simultaneous polynomial approximation, Volberg’s theorem on 344 349 Slit regions (whose boundary consists of slits along real axis) 384 3861T 401 402 418 430 439 441 525ff 540ft 545 553 564 568 Spaces 212 Spaces 223 226 Spaces 229ff 244ff Spaces 281ff Spaces of functions used in studying weighted approximation, their definitions 145 Spaces of functions used in studying weighted approximation, their definitions, 211 Spaces of functions used in studying weighted approximation, their definitions, 424 Spaces of functions used in studying weighted approximation, their definitions, 522 Szego’s theorem 7 291 292 Szego’s theorem, extension of same by Krein 9 Two constants, theorem on 257 Volberg’s theorems on harmonic measures 349 353 362 364 366 Volberg’s theorems on simultaneous polynomial approximation 344 349 Volberg’s theorems on the logarithmic integral 317ff 357 Weight 145ff Weighted approximation 145ff 385 424 Weighted approximation by polynomials 147ff 169 247 433 445 Weighted approximation by sums of imaginary exponentials 171ff Weighted approximation by sums of imaginary exponentials on closed unbounded subsets of R 428 444 Weighted approximation by sums of imaginary exponentials with a Lip 1 weight 236 Weighted approximation by sums of imaginary exponentials with a weight increasing on 243 247 Mergelian”) Weighted approximation on 447ff 523 Mergelian”) Well disposed, definition of term 452
Реклама
 |
|
О проекте
|
|
О проекте



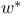 convergence
convergence 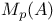 , Beurling’s
, Beurling’s  , functions
, functions 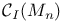 of infinitely differentiate functions
of infinitely differentiate functions 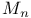 of a sequence
of a sequence 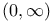
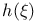 of an increasing function
of an increasing function 
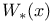 of a weight W(x) its definition
of a weight W(x) its definition 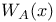 of a weight W(x) by entire functions of exponential type
of a weight W(x) by entire functions of exponential type 
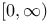
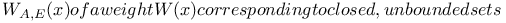 E\subseteq R$
E\subseteq R$ 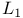 version of same
version of same 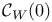 and
and 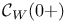
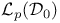


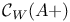
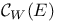 ,
, 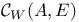 ,
, 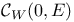
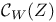 ,
,