Авторизация
Поиск по указателям
Kriegl A., Michor P.W. — The Convenient Setting of Global Analysis
Обсудите книгу на научном форуме Нашли опечатку?
Название: The Convenient Setting of Global AnalysisАвторы: Kriegl A., Michor P.W. Аннотация: This book lays the foundations of differential calculus in infinite dimensions and discusses those applications in infinite dimensional differential geometry and global analysis not involving Sobolev completions and fixed point theory. The approach is simple: a mapping is called smooth if it maps smooth curves to smooth curves. Up to Fréchet spaces, this notion of smoothness coincides with all known reasonable concepts. In the same spirit, calculus of holomorphic mappings (including Hartogs' theorem and holomorphic uniform boundedness theorems) and calculus of real analytic mappings are developed. Existence of smooth partitions of unity, the foundations of manifold theory in infinite dimensions, the relation between tangent vectors and derivations, and differential forms are discussed thoroughly. Special emphasis is given to the notion of regular infinite dimensional Lie groups. Many applications of this theory are included: manifolds of smooth mappings, groups of diffeomorphisms, geodesics on spaces of Riemannian metrics, direct limit manifolds, perturbation theory of operators, and differentiability questions of infinite dimensional representations.
Язык: Рубрика: Математика /Анализ /Продвинутый анализ /Статус предметного указателя: Готов указатель с номерами страниц ed2k: ed2k stats Год издания: 1997Количество страниц: 618Добавлена в каталог: 02.04.2005Операции: Положить на полку |
Скопировать ссылку для форума | Скопировать ID
Предметный указатель
Extension, k-jet 431 Exterior algebra 57 Exterior derivative, covariant 392 399 Exterior derivative, global formula for 342 E’, space of bounded linear functionals 8 f-dependent 366 F-evaluating 184 F-foliated diffeomorphism 272 f-related vector fields 329 f-related vector valued differential forms 366 Fast converging sequence 17 18 Fast falling 17 Fiber bundle 375 Fiber bundle, gauge group of a 479 Fiber bundle, principal 380 Fiber of the operational tangent bundle 284 Fiber of the tangent bundle 284 Fibered composition of jets 431 Final smooth mapping 272 Finite type polynomial 60 First uncountable ordinal number 49 Flat at 0, infinitely 61 Flatness, order of 539 Flip, canonical 293 Flow line of a kinematic vector field 329 Flow of a kinematic vector field, local 331 Foliation 272 Foot point projection 284 Formally real commutative algebra 305 Frame bundle 477 Frame bundle, nonlinear 477 Frechet space 577 Frechet space, graded 557 Frechet space, tame graded 559 Frechet-differentiable 128 Frobenius theorem 330 331 Frolicher space 238 Frolicher — Nijenhuis bracket 361 Functor, smooth 290 Fundamental theorem of calculus 17 Fundamental vector field 375 375 G-atlas 379 G-bundle 379 G-bundle, homomorphism of 384 G-structure 379 G?ateaux-differentiable 128 Gauge group of a fiber bundle 479 Gauge transformations 385 General curve lemma 118 Generating set of functions for a Frolicher space 239 Germ of f along A 274 Germs along A of holomorphic functions 92 Global resolvent set 549 Globally Hamiltonian vector fields 460 Graded derivations 358 Graded Frechet space 557 Graded Frechet space, tame 559 Graded-commutative algebra 57 Graph topology 435 Grassmann manifold 514 Group, diffeomorphism 454 Group, holonomy 426 Group, Lie 369 Group, reduction of the structure 381 Group, regular Lie 410 Group, restricted holonomy 426 Group, smooth 432 Groups, extension of 412 Hamiltonian vector field 460 Hausdorff, smoothly 265 Holder mapping 128 Holomorphic atlas 264 Holomorphic curve 81 Holomorphic diffeomorphisms 264 Holomorphic mapping 83 Holomorphic mappings, initial 268 Holomorphic vector bundle 287 Holonomy group 426 Homogeneous operational tangent vector of order d 277 Homomorphism of G-bundles 384 Homomorphism of principal fiber bundles 381 Homomorphism of vector bundles 289 Homomorphism over 381 Homotopy operator 355 Horizontal bundle 376 Horizontal differential forms 392 Horizontal G-equivariant W-valued differential forms 401 Horizontal lift 376 Horizontal projection 376 Horizontal space of a connection 366 Horizontal vectors of a fiber bundle 376 Induced connection 394 394 Inductive limit 577 Infinite polygon 18 Infinitely flat at 0 61 Initial mapping 268 Inner automorphism 373 insertion operator 341 399 Integral curve of a kinematic vector field 329 Integral mapping 136 Integral, definite 16 Integral, Riemann 15 Interpolation polynomial 228 Invariant kinematic vector field 370 Involution, canonical 293 Isomorphism of vector bundles 289 Isomorphism, bornological 8 Jets 431 K', set of accumulation points of K 143 k-jet extension 431 k-jets 431 kE 37 Kelley-fication 37 Killing form on 520 Kinematic 1-form 337 Kinematic cotangent bundle 337 Kinematic differential forms, vector valued 359 Kinematic tangent bundle 284 Kinematic tangent vector 276 Kinematic vector field 321 Kinematic vector field, flow line of a 329 Kinematic vector field, left invariant 370 Kinematic vector field, local flow of a 331 Kothe sequence space 71 581 L(E, F) 33 Lagrange submanifold 460 Leaf of a foliation 273 Left invariant kinematic vector field 370 Left logarithmic derivative 404 Left Maurer — Cartan form 406 Left trivialized derivative 374 Legendre mapping 468 Legendre submanifold 468 Leibniz formula 54 Lie bracket of vector fields 324 Lie derivative 347 360 Lie derivative, covariant 399 Lie group 369 Lie group, regular 410 Lift, horizontal 376 Lift, vertical 293 LIMIT 577 Limit, inductive 577 Limit, projective 577 Linear connection 396 397 Linear mapping, bounded 8 Liouville form 523 Lipschitz bound, absolutely convex 17 Lipschitz condition 9 Lipschitz mapping 128 Lipschitzian curve, locally 9 Local addition 441 Local flow of a kinematic vector field 331 Locally complete space 20 Locally convex space 575 Locally convex space, barrelled 579 Locally convex space, bornological 575 Locally convex space, bornologification of a 575 Locally convex space, bornology of a 8 575 Locally convex space, completion of a 16 Locally convex space, nuclear 580 Locally convex space, reflexive 579 Locally convex space, Schwartz 579 Locally convex space, strong dual of a 579 Locally convex space, strongly nuclear 580 Locally convex space, ultrabornologification of a 575 Locally convex space, weakly realcompact 196 Locally convex vector space, ultra-bornological 580 Locally Hamiltonian vector field 460 Locally Lipschitzian curve 9 Locally uniformly rotund norm 147 Logarithmic derivative, left or right 404 M-convergence condition 39 M-convergent net 12 M-converging sequence 12 m-evaluating 184 m-small zerosets 205 Mackey adherence 48 51 Mackey adherence of order 49 Mackey approximation property 70 Mackey complete space 15 Mackey convergent net 12 Mackey convergent sequence 12 Mackey — Cauchy net 14 Mackey, second countability condition of 159 Mackey-closure topology 19 Mackey’s countability condition 236 Manifold 264 Manifold 105 Manifold, contact 467 Manifold, natural topology on a 265 Manifold, pure 265 Manifold, symplectic 460 Manifoldstructure of 439 Mapping 1-homogeneous 34 Mapping between Frolicher spaces, smooth 239 Mapping, biholomorphic 264 Mapping, bornological 19 Mapping, bounded 19 Mapping, bounded linear 8 Mapping, carrier of a 153 Mapping, complex differentiable 81 Mapping, exponential 372 Mapping, final 272 Mapping, Holder 128 Mapping, holomorphic 83 Mapping, initial 268 Mapping, integral 136 Mapping, Legendre 468 Mapping, Lipschitz 128 Mapping, nuclear 136 Mapping, proper 445 Mapping, real analytic 102 Mapping, smooth 30 Mapping, support of a 153 Mapping, tame smooth 563 Mapping, transposed 326 Mapping, zero set of a 153 Maurer — Cartan form 373 Maurer — Cartan formula 378 Maximal atlas 264 Mean value theorem 10 Mesh of a partition 15 Minkowski functional 11 575 Modeling convenient vector spaces of a manifold 265 Modular 1-form 337 Modules, bounded 63 Monomial of degree p 60 Montel space 579 Multiplicity 539 n-th derivative 58 n-transitive action 472 Natural bilinear concomitants 367 Natural topology 488 Natural topology on a manifold 265 Net, M-convergent 12 Net, Mackey convergent 12 Net, Mackey — Cauchy 14 Nijenhuis tensor 368 Nijenhuis — Richardson bracket 359 Nonlinear frame bundle of a fiber bundle 477 Norm, locally uniformly rotund 147 Norm, rough 135 Norm, strongly rough 158 Norm, uniformly convex 204 Normal bundle 438 Normal smoothly 165 Norming pair 582 Nuclear locally convex space 580 Nuclear mapping 136 Nuclear operator 580 One parameter subgroup 371 Operational 1-form 337 Operational 1-forms of order < k 337 Operational cotangent bundle 337 Operational tangent bundle 283 Operational tangent vector 276 Operational tangent vector of order d, homogeneous 277 Operational vector field 321 Operator, differentiation 33 Operator, homotopy 355 Operator, insertion 341 399 Operator, nuclear 580 Operator, strongly nuclear 580 Operator, trace class 580 Operator, trace of an 580 Order of a derivation 277 Order of flatness 539 Ordinal number 49 Paracompact, smoothly 165 Parallel transport on a fiber bundle 378 Partition of unity 165 Pincipal bundle 380 Pincipal bundle of embeddings 474 Pincipal connection 387 Pincipal right action 380 Plaque of a foliation 273 Poduct of manifolds 264 Poduct rule 54 Poincare lemma 350 Poincare lemma, relative 461 Polar 578 Polynomial 60 Polynomial, finite type 60 Power series space of infinite type 72 Precompact 576 PRI, projective resolution of identity on a Banach space 588 Projection of a fiber bundle 376 Projection of a vector bundle 287 Projection, foot point 284 Projection, horizontal 376 Projection, vertical 293 376 Projective generator 584 Projective limit 577 Projective resolution of identity 588 Projective resolution of identity, separable 588 Proper mapping 445 Pseudo-isotopic diffeomorphisms 510 Pullback 377 Pullback of vector bundles 290
Реклама
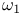
of principal bundles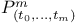 
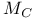(complexification of m), complex 
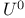of a set 
|
|
О проекте