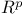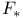|
|
 |
| Авторизация |
|
|
 |
| Поиск по указателям |
|
 |
|
 |
|
|
 |
 |
|
 |
|
| Frankel T. — The geometry of physics: An introduction |
|
|
 |
| Предметный указатель |
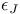 67 67
 336 336
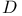 200 200
 200 200
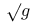 88 88
(Cross) section 50 416 466
Absolute temperature 187
Acceleration 4-vector 194
Accessibility 181 182
Accumulation point 106
action 152 274 524
Action, Euclidean 551
Action, first variation of 154
Action, group 454
Action, Hamilton's principle of stationary action 154
Action, Jacobi's principle of least action 281
Action, relativistic 196
AD 486
Ad, bundle 487 489
Ad, connection 487
Adiabatic distribution and leaf 183
Adiabatic process 180
Adjoint 392 640
Adjoint group 486
Adjoint representation 486
Admissible boundary form 378
Admittance matrix 645
Affine connection 242
Affine group of the line A(1) 394
Affine parameter 272
Aharonov — Bohm effect 447—448 554
Aharonov — Susskind and spinors 517
Algebra homomorphism 78
Ampere — Maxwell law 121 163
Annihilator subspace 167
Anticommutator 478
Antiderivation 89 135
Antiquark 649
antisymmetric 66
Associated bundle 482
Associated bundle, connection 483—487
Atiyah — Singer index theorem 465
Atlas 15
Bernoulli's theorem 234
Berry phase 468—472
Berry phase, equation 472
Bertrand — Puiseux and Diguet 288
Betti numbers 157 346
Bi-invariant, connection on a Lie group 580
Bi-invariant, forms on a Lie group 561
Bi-invariant, Riemannian metric and their geodesics 563
Bianchi identities 300 489
Binormal 196
Bochner's theorems 374 530
Bonnet's theorem 229
Boson 658
Bott's version of Morse theory 673 675
Boundary (of a manifold) = edge 106
Boundary conditions, essential or imposed 527
Boundary conditions, natural 527
Boundary group 344
Boundary homomorphism 338 601
Boundary operator 335
Bracket, anticommutator 478
Bracket, commutator 408
Bracket, Lagrange 80 100
Bracket, Lie 126 402
Bracket Lie of
Bracket, Poisson 154
Brillouin and the stress form 635
Brouwer degree 210—213 360
Brouwer degree, fixed point theorem 217
Bump form 107
Bundle, associated 482
Bundle, complex line 433
Bundle, cotangent 52
Bundle, determinant 487
Bundle, dual 482
Bundle, electromagnetic 441
Bundle, fiber 415
Bundle, frame 453
Bundle, gauge 490
Bundle, line 433
Bundle, local trivialization 417
Bundle, monopole 444 473
Bundle, normal 419
Bundle, orientable 611
Bundle, principal 454 481
Bundle, product 418
Bundle, projection 415
Bundle, pull back 622
Bundle, section 50 416 466
Bundle, space 415
Bundle, structure group 433 452
Bundle, tangent 48
Bundle, transition functions 24 254 414
Bundle, trivial 418
Bundle, unit tangent 51
Bundle, vector 413—417
Bundle, volume 488
Canonical form 394
Canonical map 149
Caratheodory's formulation of the second law of thermodynamics 181
Caratheodory's theorem 182
Cartan's, 3-form on a Lie group 566
Cartan's, bi-invariant forms 562
Cartan's, exterior covariant differential 250 430
Cartan's, method for computing curvature 257
Cartan's, structural equations 249
Cartan's, theorem 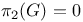 606 606
Cauchy equations of motion 618 620 628
Cauchy stress form 619
Cauchy stress form Lie
Cauchy stress form, symmetry of 621
Cauchy — Green tensor 82
Cauchy — Riemann equations 158 159
Center of a Lie algebra 580
Center of a Lie group 565
centralizer 667
Chain complex 636
Chain group 337
Chain group, integer 336
Chain group, simplicial 343
Chain group, singular 333
CHARACTER 665
Characteristic cohomology class 616
Charge form 118
Chern — Simons form 586
Chern — Weil theorem 589
Chern's forms and classes 587—591
Chern's forms and classes as obstructions
Chern's integral 612
Chern's proof of Gauss — Bonnet — Poincare 462—465 553—557
Chern's theorem 615
Chow's theorem 178 187
Christoffel symbols 229
Circulation 144 377
Clairaut's relation 530
Classical force 195
Classical momentum 194
Classical velocity 193
Clifford algebra 500
Clifford embedding 262
Clifford numbers 503
Closed form 156 158
Closed manifold 120
Closed set 11
Closure 106
Coboundary 638
Cochain 638
Coclosed 370
Cocycle 639
Codazzi equation 229 302 311—313 320
| Codifferential d* 364
Codimension 6
Coefficient group 337
Coefficient group, field 343
Cohomology  356 356
Cohomology  , integral class 615 , integral class 615
Commutative diagram 338
Commutator bracket of matrices 408
Compact 13
Completable relative cycle 387
Complex analytic map 158 214
Complex line bundle 433
Complex line bundle connections
Complex manifold 21
Composing rotations 499
Configuration space 9 50
Conformally related metrics 531
Conjugate point 327
Conjugates 667
Connected space 347
Connection 242
Connection on a Lie group 580
Connection on a Lie group flat
Connection on a vector bundle 428—431
Connection on the associated Ad bundle 486
Connection, coefficients of 243 429
Connection, curvature of 244
Connection, electromagnetic 440
Connection, flat 260
Connection, forms  249 256 249 256
Connection, forms 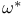 in the frame bundle 462 480 in the frame bundle 462 480
Connection, induced 309
Connection, Levi-Civita or Riemannian 242 245
Connection, Simon 472
Connection, spinor 518—521
Connection, symmetric 245
Connection, torsion of 245
Connection, torsion-free 245
Constraint, holonomic 175
Constraint, nonholonomic 175
continuous 12
Continuum mechanics 617—635
Continuum mechanics equilibrium
Contractible to a point 161
Contraction 89
Contravariant tensor 59
Contravariant vector 23
Coordinate, change of 29
Coordinate, compatible 15
Coordinate, frame 243
Coordinate, homogeneous 17
Coordinate, inertial 192
Coordinate, local 3 4 13
Coordinate, map 20
Coordinate, patch 20
Coset space G/H 456
Coset space G/H, fundamental principle 457
Cotangent space 40
Coupling constant or charge 539
Covariance 430
Covariant components of a tangent vector 43
Covariant constant 267
Covariant derivative 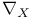 235 241—244 430
Covariant second 235 241—244 430
Covariant second
Covariant derivative 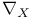 of a tensor 298—299 of a tensor 298—299
Covariant differential  , exterior 248 , exterior 248
Covariant tensor 58
Covariant vector = covector 41
Covector 41
Covector, transformation law 42
Covering space 569—576
Covering space, associated to a subgroup of  575 575
Covering space, orientable 573
Covering space, universal 570
Covering space universal covering
Critical manifolds 673
Critical points and values 28 382—387
Critical points and values, homotopically 382 387
Critical points and values, index 384
Critical points and values, inessential 383
Critical points and values, nondegenerate 383
Curl 93
Current, 2-form  118 118
Current, 3-form 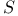 199 199
Current, 3-vector 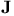 119 119
Current, 4-vector J 199
Current, convective 119
Current, electric, as a chain 644
Curvature and parallel displacement 259—261
Curvature of a connection 243
Curvature of a space curve 191
Curvature of a surface 207
Curvature of a surface of revolution 258
Curvature of the Poincare metric 258
Curvature, extrinsic 318
Curvature, forms  251 256 431
Curvature forms 251 256 431
Curvature forms
Curvature, forms  , , 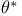 on a principal bundle 481 on a principal bundle 481
Curvature, forms  , of a surface 257
Curvature forms , of a surface 257
Curvature forms
Curvature, Gauss 207
Curvature, geodesic 235
Curvature, intrinsic 318
Curvature, mean 207
Curvature, principal 207
Curvature, Riemann sectional K(X 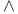 Y) 313 Y) 313
Curvature, Riemann tensor 244
Curvature, total 215
Curvature, transformation R(X, Y) 244
Curvature, vector 192 194
Cycle, absolute 344
Cycle, completeable 387
Cycle, group 344
Cycle, relative 379
D'Alembertian  293 371 293 371
de Rham's theorem 355—360
de Rham's vector space 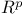 356 356
Deformation, retract 406 506
Deformation, tensor 82
Deformation, theorem 350
Degree of a map see "Brouwer degree"
Derivation 134
Derivative, covariant 235
Derivative, exterior 73
Derivative, intrinsic 235
Derivative, normal 364
Determinant line bundle 487
Dictionary relating forms and vectors 94
Diffeomorphism 27
Differentiable 20
Differential form see "Form"
Differential of a function 40
Differential of a map 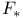 7 27 7 27
Differential, exterior d 73
Differential exterior covariant
Differentiation of integrals 138—143
Dirac (4-component) spinor 513
Dirac adjoint or conjugate spinor 532
Dirac algebra 509
Dirac equation 503
Dirac Lagrangian 531
Dirac matrices 510
Dirac monopole 444
Dirac monopole quantization
Dirac operator 511 514 521
Dirac
Dirac program 502
Dirac representation  512 512
Dirac string 162
Dirichlet's principle 373
Distance from a point to a hypersurface 579
Distribution (of subspaces) 166
Distribution (of subspaces), adiabatic 183
Distribution (of subspaces), horizontal 263
Distribution (of subspaces), integrable 167
Divergence 93 136 304
Divergence of a form 365
Divergence of a symmetric tensor 300
Divergence theorem 139
Divergence, exterior covariant 545
|
|
 |
| Реклама |
 |
|
|
 |
|
О проекте
|
|
О проекте



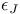

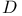

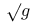
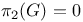


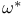 in the frame bundle
in the frame bundle 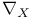
 , exterior
, exterior 

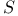
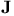

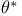 on a principal bundle
on a principal bundle  Y)
Y)