|
|
 |
| Авторизация |
|
|
 |
| Поиск по указателям |
|
 |
|
 |
|
|
 |
 |
|
 |
|
| Burnside W. — Theory of Groups of Finite Order |
|
|
 |
| Предметный указатель |
Abel, quoted 46
Abelian group of order 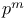 and type (1, 1, ..., 1) 58 and type (1, 1, ..., 1) 58
Abelian group of order 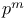 and type (1, 1, ..., 1), group of isomorphisms of 243 244 and type (1, 1, ..., 1), group of isomorphisms of 243 244
Abelian group of order 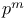 and type (1, 1, ..., 1), holomorph of 245 and type (1, 1, ..., 1), holomorph of 245
Abelian group of order 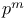 and type (1, 1, ..., 1), number of distinct ways of choosing a set of independent generating operations of 59 and type (1, 1, ..., 1), number of distinct ways of choosing a set of independent generating operations of 59
Abelian group of order 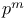 and type (1, 1, ..., 1), number of sub-groups of, whose order is given 60 and type (1, 1, ..., 1), number of sub-groups of, whose order is given 60
Abelian group, definition of 46
Abelian group, definition of, existence of independent generating operations of 52
Abelian group, definition of, invariance of the orders of a set of independent generating operations of 54
Abelian group, definition of, sub-groups of 47 48 55—59
Abelian group, definition of, symbol for, of given type 55
Alternating group, definition of 139
Alternating group, definition of, group of isomorphisms of 246
Alternating group, definition of, is simple, except for degree 4 154
Bochert, quoted 153
Bolza, quoted 162
Burnside, quoted 65 97 157 311 317 335 345 352 365 370
Caucht, quoted 90
Cayley, quoted 306
Characteristic series, definition of 232
Characteristic series, definition of, invariance of 233
Characteristic series, definition of, of a group whose order is a power of a prime 233—235
Characteristic sub-group, definition of 232
Characteristic sub-group, groups with no, are either simple or the direct product of simply isomorphic simple groups 232
Chief composition series, or Chief series, definition of 123
Chief composition series, or Chief series, invariance of 123
Cole and Glover, quoted 137
Cole, quoted 196 370
Colour-groups 306—310
Colour-groups, examples of 310
Complete group, definition of 236
Complete group, group of isomorphisms of a simple group of composite order is a 238
Complete group, groups which contain a, self-conjugately are direct products 236
Complete group, holomorph of a cyclical group of odd order is a 241
Complete group, holomorph of an Abelian group of order 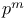 and type (1, 1, ..., 1) is a 239 and type (1, 1, ..., 1) is a 239
Complete group, symmetric group is a, except for degree 6 246
Composite groups, definition of 29
Composite groups, definition of, non-soluble 376—378
Composite groups, definition of, of even order 360—365
Composition factors, definition of 118
Composition-series, definition of 118
Composition-series, examples of 128 129
Composition-series, invariance of 122
Conjugate operations, definition of 27
Conjugate operations, definition of, complete set of 31
Conjugate sub-groups, complete set of 32
Conjugate sub-groups, definition of 29
Conjugate sub-groups, operations common to or permutable with a complete set of, form a self-conjugate sub-group 33
Dedekind, quoted 89
Defining relations for groups of genus unity 301 302
Defining relations for groups of genus zero 291
Defining relations for groups of order 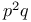 133—137 133—137
Defining relations for groups of order pq 100
Defining relations for groups of orders 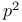 , , 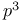 , , 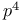 87 88 89 87 88 89
Defining relations for groups whose orders contain no square factor 354
Defining relations for the holomorph of a cyclical group 240 241
Defining relations for the simple group of order 168 305
Defining relations for the symmetric group of degree 5 305
Defining relations of a group, definition of 21
Defining relations, limitation on the number of, when the genus is given 283
Degbee of a substitution group, definition of 138.
Degbee of a substitution group, is a factor of the order, if the group is transitive 140
Direct product of two groups, definition of 40
Direct product of two groups, represented as a transitive group 190
Direct product of two simply isomorphic groups of order n represented as a transitive group of degree n 146 147.
Doubly transitive groups, generally contain simple self-conjugate sub-groups 192
Doubly transitive groups, of degree n and order n(n-1) 155—157
Doubly transitive groups, the sub-groups of, which keep two symbols fixed 212
Doubly transitive groups, with a complete set of triplets 214
Dtcck, quoted 22 44 172 195 255 292 301 305
Dyck’s theorem that a group of order n can be represented as a regular substitution group of degree n 22—24
Factor groups, definition of 38
Factor groups, invariance of 118.
Factor groups, set of, for a given group 118
Forsyth, quoted 280 283
Fractional linear group, analysis of 319—333
Fractional linear group, definition of 311
Fractional linear group, generalization of 334 335
Frobenius and Stickelberger, quoted 46
Frobenius, quoted 39 45 65 71 90 97 108 110 114 166 232 250 251 345 354 358 360
Frobenius’s theorem that, if n is a factor of the order of a group, the number of operations of the group whose orders divide n is a multiple of n 110—112
Galois, quoted 192 334
General discontinuous group with a finite number of generating operations 256
General discontinuous group, relation of special groups to 257 258
Genus of a group, definition of 280
Gierster, quoted 311
Graphical representation, of a cyclical group 260 261 288
Graphical representation, of a general group 262—266
Graphical representation, of a group of finite order 273—278
Graphical representation, of a group of finite order, examples of 269—272 279 288—290 294 296 299 300 303 310
Graphical representation, of a special group 266—269
Group of isomorphisms, contains the group of cogredient isomorphisms self-con jugately 226
Group of isomorphisms, of a cyclical group 239—242
Group of isomorphisms, of an Abelian group of order  and type (1, 1, ..., 1) 243 244 311—317 336—338 and type (1, 1, ..., 1) 243 244 311—317 336—338
Group of isomorphisms, of doubly transitive groups of degree 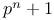 and order and order  246—248 246—248
Group of isomorphisms, of the alternating group 246
Group, abelian 46
Group, alternating 139
Group, complete 236
Group, continuous, discontinuous, or mixed 13 14
Group, cyclical 25
Group, defining relations of 21
Group, definition of 11 12
Group, dihedral 287
Group, fundamental or generating operations of 21
Group, general 256
Group, genus of a 280
Group, group of isomorphisms of a 223
Group, holomorph of a 228
Group, icosahedral 289
Group, multiplication table of 20 49
Group, octohedral 289
Group, order of 14
Group, quadratic 326
Group, simple and composite 29
Group, soluble 130 .
Group, special 257
Group, symbol for a 27 (see also “Substitution group”)
Group, symmetric 139
Group, tetrahedral 289 .
Groups of genus one 295—302
Groups of genus two 302
Groups of genus zero 286—292
Groups whose order is 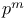 , where p is a prime 61 et seq. , where p is a prime 61 et seq.
Groups whose order is always contain self-conjugate operations 62
Groups whose order is case in which there is only one sub-group of a given order 72—75
Groups whose order is determination of distinct types of orders 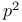 , , 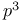 , , 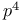 , where p is an odd prime 81—88 , where p is an odd prime 81—88
Groups whose order is every sub-group of, is contained self-conjugately in a greater sub-group 65
Groups whose order is number of sub-groups of given order is congruent to unity, mod. p 71
Groups whose order is number of types which contain self-conjugate cyclical sub-groups of order 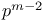 76—81 76—81
Groups whose order is table of distinct types of orders 8 and 16 88 89
Groups whose orders contain, no cubed factor 359
Groups whose orders contain, no squared factor 353 354
Groups whose sub-groups of order 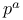 are all cyclical 352 353 are all cyclical 352 353
Heffter, quoted 215
Holder, quoted 38 87 119 137 224 236 246 249 344 353 370 376
Holomorph of a cyclical group 240—242
Holomorph of an Abelian group of order  and type (1, 1, ... 1) 245 and type (1, 1, ... 1) 245
Holomorph, definition of 228
Homogeneous linear group, composition series of 314—317
Homogeneous linear group, definition of 244
| Homogeneous linear group, generalization of 340—342
Homogeneous linear group, represented as a transitive substitution group 336 337
Homogeneous linear group, simple groups denned by 338 339
Hukwitz, quoted 24 280 282
Identical opebation, definition of 12
Imprimitive groups of degree 6 181 182
Imprimitive groups, definition of 171
Imprimitive self-conjugate sub-group of a doubly transitive group 193 194
Imprimitive systems of a regular group 173
Imprimitive systems of any transitive group 176
Imprimitive systems, definition of 172
Imprimitive systems, properties of 185 186
Intbansitive groups, definition of 140
Intbansitive groups, of degree 7 with transitive sets of 3 and 4 163 164
Intbansitive groups, properties of 159—162
Intbansitive groups, transitive sets of symbols in 159 166
Isomoephism of general and special groups 257 258
Isomoephism of two groups, general, definition of 41
Isomoephism of two groups, multiple, definition of 36
Isomoephism of two groups, simple, definition of 32
Isomoephisms of a group with itself, definition of 222
Isomoephisms of a group, class of, definition of 224
Isomoephisms of a group, cogredient and contragredient, definition of 224
Isomoephisms of a group, limitation on the order of 252
Joedan, quoted 46 119 143 146 149 150 153 189 196 198 204 311
Klein and Feicke, quoted 162
Klein, quoted 224 289 292
Limitation on the number of defining relations of a group of given genus 283
Limitation on the order of a group of given genus 282
Maeggeaff, quoted 198
Maillet, quoted 108
Maschke, quoted 310
Mathieu, quoted 155
Maximum self-conjugate sub-group, definition of 35
Maximum sub-group, definition of 35
Millee, quoted 105 196 259
Minimum self-conjugate sub-group is a simple group or the direct product of simply isomorphic simple groups 127
Minimum self-conjugate sub-group, definition of 124
Mooee, quoted 215 335
Multiplication table of a group 20 49
Multiply isomoephic groups, definition of 36
Netto, quoted 141 198 215
Numbee of symbols unchanged by all the substitutions of a group 165 166
Operations common to two groups form a group 27
Operations of a group, which are permutable with a given operation or sub-group, form a group 29 31
Operations, common to or permutable with each of a complete set of conjugate subgroups form a self-conjugate sub-group 33
Order of a group, definition of 14
Order of an operation, definition of 14
Permutability of an operation with a group, definition of 29
Permutable groups, definition of 41
Permutable opeeations, definition of 12
Primitive groups, definition of 171
Primitive groups, limit to the order of, for a given degree 199
Primitive groups, of degree 6 205 206
Primitive groups, of degree 7 206—208
Primitive groups, of degree 8 209—211
Primitive groups, of degrees 3, 4 and 5 205
Primitive groups, of prime degree 201
Primitive groups, when soluble, have a power of a prime for degree 192
Primitive groups, with a transitive sub-group of smaller degree 197 198
Primitivity, test of 184
Regular division of a surface, representation of a group by means of 278
Repeesentation, graphical see “Graphical representation”
Representation of a group, in primitive form 177
Representation of a group, in transitive form. i.e. as a transitive substitution group 22 173—179
Self-conjugate operation, definition of 28
Self-conjugate operation, of a group whose order is the power of a prime 62
Self-conjugate operation, of a transitive substitution group must be a regular substitution 144
Self-conjugate sub-group, definition of 29
Self-conjugate sub-group, generated by a complete set of conjugate operations 34
Self-conjugate sub-group, of a k-ply transitive group is, in general, (k-1)-ply transitive 189
Self-conjugate sub-group, of a primitive group must be transitive 187
Self-conjugate sub-group, of an imprimitive group 187 188
Simple groups, definition of 29
Simple groups, whose orders are the products of not more than 5 primes 367—370
Simple groups, whose orders do not exceed 660 370—375
Simply isomorphic groups, definition of 22
Simply isomorphic groups, said to be of the same type 22
Soluble groups, definition of 130
Soluble groups, properties of 131 132
Soluble groups, special classes of 345—359
Sub-group, definition of 25
Sub-group, order of a, divides order of group 26 (see also “Conjugate” “Self-conjugate”
Substitution group, conjugate substitutions of, are similar 144
Substitution group, construction of multiply transitive 150
Substitution group, degree of transitive, is a factor of its order 140
Substitution group, degree of, definition of 138
Substitution group, doubly transitive, of degree n and order n(n-l) 155—157
Substitution group, limit to the degree of transitivity of 152
Substitution group, multiply transitive, definition of 148
Substitution group, order of a ft-ply transitive, whose degree is n, is a multiple of n(n-1)...(n-k+1) 148
Substitution group, primitive and imprimitive, definition of 171
Substitution group, quintuply transitive, of degree 12 170
Substitution group, regular, definition of 24
Substitution group, representation of any group as a regular 22
Substitution group, transitive and intransitive, definition of 140
Substitution group, transitive, whose substitutions, except identity, displace all or all but one of the symbols 141—144
Substitution group, triply transitive, of degree n and order n(n-l)(n-2) 158
Substitution groups whose orders are powers of primes 218 219
Substitution, circular 7
Substitution, cycles of 2
Substitution, definition of 1
Substitution, even and odd 10
Substitution, identical 4
Substitution, inverse 4
Substitution, order of 6
Substitution, permutable 8
Substitution, regular 7
Substitution, similar 7
Substitution, symbol for the product of two or more 4
Substitutions which are permutable, with a given substitution 215 216
Substitutions with every substitution of a given group 145 146 217
Substitutions with every substitution of a group, whose degree is equal to its order, form a simply isomorphic group 146
Sylow, quoted 62 90
Sylow’s Theorem 92—95
Sylow’s theorem, extension of 110
Sylow’s theorem, some direct consequences of 97—100
Symbol for a group generated by given operations 27
Symbol for the product of two or more operations 12
Symbol for the product of two or more substitutions 4
Symbol, 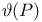 and and 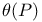 , definition of 250 251 , definition of 250 251
Symmetric group, definition of 139
Symmetric group, is a complete group, except for degree 6 246
Symmetric group, of degree 6 has 12 simply isomorphic sub-groups of order 5! which form two distinct conjugate sets 200
Symmetric group, of degree n has a single set of conjugate sub-groups of order (n-1)! except when n is 6 200
Transforming an operation, definition of 27
Transitive group, definition of 140
Transitive group, number of distinct modes of representing the alternating group of degree 5 as a 179 180
Transitive group, representation of any group as a 175—179
Transpositions, definition of 9
Transpositions, number of, which enter in the representation of a substitution is either always even or always odd 10
Transpositions, representation of a substitution by means of 9
Triply transitive groups of degree n and order n(n-l)(n-2) 158
type of a group see “Simply isomorphic groups”
Types of group 8 or 16 88 89
Types of group 24 101—104
Types of group 60 105—108
Types of group, 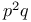 , where p and q are different primes 133—137 , where p and q are different primes 133—137
Types of group, distinct, whose order is 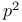 , , 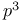 , or , or 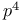 , where p is an odd prime 87 88 , where p is an odd prime 87 88
Types of group, pq, where p and q are different primes 100 101
Weber, quoted 46
Young, quoted 63 68 87 310
|
|
 |
| Реклама |
 |
|
|
 |
|
О проекте
|
|
О проекте



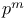 and type (1, 1, ..., 1)
and type (1, 1, ..., 1) 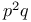
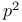 ,
, 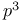 ,
, 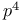
 and type (1, 1, ..., 1)
and type (1, 1, ..., 1) 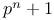 and order
and order 
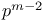
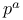 are all cyclical
are all cyclical 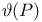 and
and 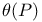 , definition of
, definition of