Авторизация
Поиск по указателям
De LA Pena V., Gine E. — Decoupling: From Dependence to Independence: Randomly Stopped Processes U-Statistics and Processes Martingales and Beyond
Обсудите книгу на научном форуме Нашли опечатку?
Название: Decoupling: From Dependence to Independence: Randomly Stopped Processes U-Statistics and Processes Martingales and BeyondАвторы: De LA Pena V., Gine E. Аннотация: This book presents the theory and several applications of the decoupling princi-ple, which provides a general approach for handling complex problems involving dependent variables. Its main tools consist of inequalities used for breaking (decoupling) the dependence structure in a broad class of problems by introducing enough independence so that they can be analyzed by means of standard tools from the theory of independent random variables.
Язык: Рубрика: Математика /Статус предметного указателя: Готов указатель с номерами страниц ed2k: ed2k stats Год издания: 1999Количество страниц: 392Добавлена в каталог: 19.09.2006Операции: Положить на полку |
Скопировать ссылку для форума | Скопировать ID
Предметный указатель
216 (dual) bounded Lipschitz distance 180 214 Angularly symmetric distribution 268—269 Anscombe's Theorem for degenerate V-statistics 363 366 Anscombe's Theorem for sequences 362 363 Anscombe's Theorem for sums of independent variables 361 Asymptotic equicontinuity criterion 211 Banach space of type 2 249 Bracketing numbers 233 Burkholder — Davis — Gundy inequalities for martingales 34 316 Burkholder — Gundy inequalities for randomly stopped processes 81—82 Burkholder — Gundy type exponential inequality 84 Canonical kernel 137 Central limit theorem for B-valued V-statistics 250 Central limit theorem for martingales (Brown Eagleson) 330 Central limit theorem for two 331 Central limit theorem for U-processes 238—247 Central limit theorem for U-statistics 180—183 Chaining 216—218 CI condition 293 Completely degenerate kernel see canonical kernel Conditional Jensen inequalities for nonnegative, nondecreasing functions 123—125 Conditional Khinchin inequalities for nonnegati ve, nondecreasing functions 122—123 Conditionally symmetric sequences 293 Conditionally symmetric sequences, inequalities 302 308 312—315 Contraction principle 6—7 114 Convergence in law in 209 Covering numbers (of a metric space) 216 Criterion function (for M-estimators) 265 Cumulative hazard function 281 Decoupled sequences 293 Decoupled version 293 Decoupling counter-examples 345 348 Decoupling of autorregressive models 295 Decoupling of constrained intersections of sets 298 Decoupling of constrained products 297 Decoupling of constrained sums 300 Decoupling of Gaussian chaos 183—186 Decoupling of intersections of tangent events 298 299 Decoupling of martingales 34—35 300 320 322 Decoupling of moment generating functions of tangent sequences 300 Decoupling of multilinear fonns in independent random variables 135—136 Decoupling of order statistics 299 Decoupling of products of tangent variables 297 Decoupling of randomly stopped Bessel processes 83 Decoupling of randomly stopped sums 54 62 321 Decoupling of randomly stopped U-statistics 352 356 Decoupling of sampling without replacement 295 Decoupling of sums of nonnegative variables 33—34 Decoupling of sums of tangent variables: 307 308 312 336 347 348 Decoupling of sums of tangent variables: moment generating function 300 Decoupling of sums of tangent variables: tail probabilities 302 308 313 345 Decoupling of U-processes: convex functions 107 Decoupling of U-processes: tail probabilities 125 134 Decoupling of U-statistics and U-processes 97—98 Decoupling of U-statistics: convex functions 99 Decoupling of U-statistics: moment generating functions 301 Decoupling of U-statistics: tail probabilities 125 Decoupling of UMD (Unifonn martingale difference)-martingales 323 Degenerate kernel 137 Degree of degeneracy of a U-statistic 137 Differentially subordinate sequences and inequalities 313—315 Dudley's theorem (on sample continuity of Gaussian processes) 219 Empirical process 237 Envelope of a class of functions 107 224 Exponential inequalities for canonical U-processes 252 Exponential inequalities for canonical U-statistics 167 Exponential inequalities for chaos 116 118 Exponential inequalities for martingales and ratios 369—370 Exponential inequalities for the ratio of a martingale over its conditional variance 369—374 Exponential inequalities for U-statistics 165 167 171 Exponential inequalities Hoeffding's, for U-statistics 165 Exponential inequalities, Bennett's 167 Exponential inequalities, Bennett's for martingales 367 368 Exponential inequalities, Bernstein's 166 Exponential inequalities, Bernstein's for martingales 367 368 Exponential inequalities, maximal, for processes (chaining) 215—221 Exponential inequalities, maximal, for random variables 189 Exponential inequalities, Prokhorov's for martingales 372 First passage times for a the maximum of a group of agents in a market 84 First passage times for Bessel processes 83 84 First passage times for sums of i.i.d. variables 54 55 First passage times for the maximum volume of a group of spheres 86 87 First passage times, comparison between two processes 92 Fubini's inequality for outer expectations 106 Gaussian chaos 117—118 120 122 173—180 Gaussian chaos process 180 220 Gaussian process 173 219 Generalized Minkowski inequality 112 Hermite polynomials 176 Hoeffding's decomposition 137 Hoeffding's inequality for sampling without replacement 295 Hoffmann — Jorgensen inequalities 8—15 47 155 Hoffmann — Jorgensen inequalities for U-statistics and processes 155—160 Hypercontractivity of Gaussian chaos 117—118 Hypercontractivity of multinomial linear forms 131—132 Hypercontractivity of Rademacher chaos 110—116 Hypergeometric distribution 331 332 Hypergeometric distribution, noncentral 331—333 Hypergeometric distribution, noncentral (representation as sum of independent variables) 333 Identifiability (of parameters) 265 Image admissible Suslin classes of functions 138 Integrability in the CLT 180 181 Integrability in the LIL 192 200—205 Integrability in the LLN 161—164 Isonormal Gaussian process 173 k-function 28—32 56 57 Khinchin inequalities 15—20 121—122 Khinchin — Kahane inequalities 15—20 L-function 35—42 57 Law of large numbers for B-valued U-statistics 234 Law of large numbers for decoupled U-processes 235 Law of large numbers for the empirical simplicial median 267 Law of large numbers for U-processes 228—233 Law of large numbers for U-statistics 160—164 Law of large numbers for V-processes 236 Law of the iterated logarithm for B-valued U-statistics, bounded 255 Law of the iterated logarithm for B-valued U-statistics, compact 262 Law of the iterated logarithm for decoupled and/or randomized U-statistics 198 Law of the iterated logarithm for U-processes, bounded 255 Law of the iterated logarithm for U-processes, compact 256 Law of the iterated logarithm for U-statistics, bounded 192—193 Law of the iterated logarithm for U-statistics, compact I 95 Levy's maximal inequalities 2—7 Levy's maximal inequalities for processes with independent increments 81 M-estimator 265 279 Marcinkiewicz inequalities 34 Marcinkiewicz law of large numbers 162 Marcinkiewicz type law of large numbers for U-statistics 235 Maximal inequality for exponential Orlicz norms 189 Measurability 8 15 106 138 Measurable classes of functions 138 Measurable envelope 107 224 Metric entropy 216 Newton's identities 175 Orlicz norms, Orlicz spaces 36 Outer integral, expectation, probability 106 P-Donsker class of functions 237 Packing numbers (of a metric space) 216 Paley — Zygmund argument 119 Polarization fonnula 174 Principle of conditioning, almost sure convergence 327 Principle of conditioning, weak convergence 328 Product limit estimator (Lynden — Bell) 282 Rademacher chaos 110—118 120 122—125 Rademacher chaos processes 220 Rademacher variables, Rademacher sequences 12 16 Random distances 228 231 241 Randomization in the law of the iterated logarithm for U-processes 148 Randomization inequalities for martingales 300 Randomization inequalities for sums 12 139 Randomization inequalities for U-statistics and U-processes: convex functions 140 144 Randomization inequalities for U-statistics and U-processes: tail probabilities 146 148 Rosenthal inequalities 43—46 Rosenthal inequalities for martingales 322 Rosenthal inequalities for martingales (sharp constants) 337 Sample bounded process 209 Sample continuous process 219 Sampling, conditionally independent 295 Sampling, conditionally independent, with replacement 295 Sampling, conditionally independent, without replacement 295 Separable process 218 Simplicial depth function 232 264 Simplicial depth process 232 248 Simplicial median, empirical simplicial median 265 Statistics of directions (example on) 248 Stochastic differentiability 274—275 280 Strassen's law of the iterated logarithm 194 205 Tangent sequences 293 Tetrahedral polynomial 118 Three series theorem 326 Truncated data 280—288 Two- 331 U-process 97 207 U-statistic 97 U-statistics, moments 358 U-statistics, randomization 358 U-statistics, randomly stopped (moments) 351 356 359 V-statistic or von Mises statistic 235 Vapnik — Cervonenkis class of sets 221 Vapnik — Cervonenkis subgraph class of functions 224 Wald's equations for randomly stopped processes 80 Wald's equations for sums of independent random variables 52 54 Wald's equations for U-statistics 351 356 Wald's equations, re-formulation 51 Weak convergence in probability 328 Woodroofe (Nelson — Aalen type) estimator 281 Young function or Young modulus 36 188 Young moduli of exponential type 188—189
Реклама
 |
|
О проекте
|
|
О проекте



 -capacity
-capacity  two tables
two tables 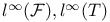
 moments
moments