|
|
 |
| Авторизация |
|
|
 |
| Поиск по указателям |
|
 |
|
 |
|
|
 |
 |
|
 |
|
| Griess R.L.Jr. — Twelve sporadic groups |
|
|
 |
| Предметный указатель |
Hamming weight 25
Happy Family 1 131
Harada, Koichiro 134
Held, Dieter 134
Heptacode [4,2,3] 29 122
Hermitian inner product 26
Hexacode balance 34
Hexacode,  2 30ff 2 30ff
Hexad 80
HI-representation 10
Higman — Sims group 104 116
Higman — Sims group on 100 and 176 points 114
Higman, Donald 116 123
Higman, Graham 134 136
HiS 104 116
HJ 104 123
Holomorph 20
Holt 13
Homological algebra 1
Huppert 20
Indecomposable root system 23
Interpreter, left and right 84
Involutions acting on  109 109
Involvement table 153
Isometric 21
Isotropic 25 92
Isotropic subspaces, formula for the number of 28
j, elliptic modular function 135
Janko, Zvonimir 135 137
Jordan canonical form 85 107
Jordan's Theorem 3
k-fold transitivity 5
Kernel of extension 11
L see "Lattice"
L( ) 55ff ) 55ff
L(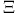 ) 55ff ) 55ff
Labeled set 77
Labeled set, associated 78
Labeling analysis for a frame 102
Labeling map, 6-tuple 39
Labeling map, i-th component 39
Labeling Procedure 44 82ff
Lattice 1 88
Lattice; determinant; volume; automorphism group of a lattice; signature of a lattice; positive definite lattice; negative definite lattice; indefinite lattice; hyperbolic lattice; dual of a lattice; L* 89
Lattice; integral lattice; ambient vector space (for a lattice); nondegenerate, radical 88
Leech lattice 1 86 95ff
Leech triangle 37
Left interpreter 85
Leon, Jeffrey 134
Liber product 132
Lie type, finite groups of 16
Linear error correcting codes 24 25
lines 10
Long Exact Sequence 12
Ly 136
Lyons, Richard 136 137 138
M 95
Maps(A,B) 9
Mathieu groups 1
Maximal subgroups of 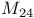 54 54
Maximal subgroups of Aut( ) 127 ) 127
Maximality of 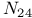 100 100
McKay, John 134 135 136
MCl 104 108
McLaughlin group 104 108
Meierfrankenfeld, Ulrich 133
Minimum weight 25
Modular forms 92
Mon(12,3) 85
Mon(n,F), Mon*(n,F) 26 27
Monomial matrices 9 85
Monomial transformation 26
Monster 1 13
Monster type 133
Monster, Baby 134
Moonshine 134
Moufang loop 133
Multiplication by  42 43 42 43
Multiplier, Multiplikator 15
Natural bilinear form 25
Natural quadratic form 25
Natural transformations 12
Niemeier lattice 93
Niemeier lattice without roots 96
Niemeier lattice, theta function of 96
Nondefective 24
Nondegenerate 21
Nonsingular 24
Nonsplit 11
Normalizer of a subgroup of order 11 106
Norton, Simon 3 132 134
O'Nan, Michael 137
Octad 36
Octad triangle 37
Orbit decomposition 54
Orbits of Aut(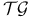 ) on ) on 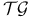 87 87
Orbits of ZS on 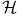 31 31
Order 10
Orthogonal group 21
Overweight 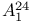 -lattice 98 -lattice 98
p-group 19
p-local subgroup 104
Parameters for a code 25 39
Parameters for rank 3 groups: n, k, l,  , ,  , , 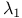 , , 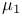 , d, , d,  124 124
Pariahs 2 131 135
Parity 40
Parker, Richard 3 137
Partial holomorph 20
Partition 9
Patterson, N.J. 127
Pentacode, [6,3,4] 29 122
Perfect 1-error correcting code 35
Perfect field 20
Perm(6,4) 30
Perm(n,F) 26
Permutation group 5ff
Permutation module 6
Permutations, useful 49
PGL(2,11) 56 63 66
POINTS 10
Polynomial identity 133
Presentation of symmetric and alternating group 22
Presentation, Chevalley — Steinberg 22
Primitive permutation group 5
Projective involutions 112
Projective plane 10
PSL(2,1) 14 56 61
PSL(2,11) 64 66
PSL(2,23) 56 66
PSL(2,7) labeling 73
PSL(2,9) 18
PSU(3,5) 108 114
PSU(4,3) 108
PSU(6,2) 123
Pullback 132
Pure subspace 69
Quadratic form; bilinear function associated to a quadratic form; defective; degenerate; nondegenerate; nondefective; nonsingular; singular; totally singular 24
Quartet 81
Quartet, standard 81
| Quotient of extension 11
R(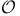 ) 54ff 109ff ) 54ff 109ff
R(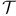 ) 54ff ) 54ff
R(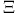 ) 54ff ) 54ff
R(i) 59
Rank 3 116 123ff
Representation group 15
Right interpreter 84
Robinson, Derek 19
Romans, I, II, III 67
Root group 9 68
Root system 23 93ff
Roots 93ff
Ru 136
Rudvalis, Aranas 136
S=VT 30
Scalar labeling 39
Schur multiplier, Multiplikator 15
Schur — Zassenhaus theorem 8
Segev, Y. 133
Self orthogonal 26 76
Semiintegers 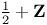 95 95
Semilinear map 68
Semisimple elements in orthogonal groups 117
Septacode, [4,2,3] 29
Sextet 38
Sextet labelings, useful 48
Sextet stabilizer 42
Sextet, unordered 41
Sharp transitivity 5 85
Short exact sequence 11
Shuffle labeling 84
Shult, Ernest 35
Signed point 77
Simplicity of 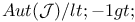 84 84
Simplicity of .223 107
Simplicity of HiS 114
Simplicity of PSL(m,q) 75
Sims, Charles 116 136 137
Simultaneous componentwise downward extension 22
Simultaneous componentwise upward extension 22
SL(2,3) 29
Sl(n, q) 18 19
slope 67
Smith, Peter 134
Smith, Steve 133
SO(n,K) 23
Solomon, Ronald 137
Solvable doubly transitive group on 9 points 87
Sp(2n,q) 18 19
Sp(6,2) 123
Special 6-set 69
Special hexad 80
Spectra of the 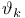 118 118
Spectrum 116
Spin(n,K) 23
Split extension 11
Sporadic simple groups 1 17 131
Square lattice 91
Stabilizer 5
stabilizers 125 126
Standard basis 26 48 84 95
Standard frame in Leech lattice 95 98
Standard Leech lattice 95
Standard quartet 81
Steinberg group 22 23
Steinberg, Robert 15
Steiner system 36
Steiner system  (5,6,12) 80 (5,6,12) 80
SU(4,2) 118
Supp 79
Suz 104 120 124 127ff
Suzuki group 104 120 124 127ff
Suzuki rank 3 sequence 124
Sylow 11-normalizers 63
Sylow 23-normalizer 99
Symbols for sporadic groups 4
Symmetric and alternating group 7
Symmetric and alternating group presentation 22
Symmetric difference 24
Symmetric relation 124
System of imprimitivity 5
Ternary Golay code 2 78 83 102
Ternary Golay code, uniqueness 83
Tetracode, 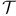 76 76
Tetracode, error correcting properties of 77
Thackray, Jonathan 3 137
Theta function of  , , 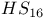 92 92
Theta function of Niemeier lattice 96
Theta function or theta series 92 113
Thickened codes 27
Thickened extended Hamming code 72
Thompson's transfer theorem 8
Thompson, John 8 134 135
Tits, Jacques 132 133
Todd, John 36 54
Totally singular 24
Transitivity 5
Transitivity of 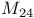 on dodecads 61 on dodecads 61
Transitivity of 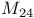 on octads 57 on octads 57
Transitivity on frames 99
Transitivity on triangles of type 233 113
Transitivity on type 2 vectors 98
Transitivity on type 3 vectors 101
Transposition group, {3,4}- 128 133
Transverse 6-set 69
Triangle of type abc 101
Triangular group 9
Trio of octads 54 121
Type n 95
Uniqueness of Golay code 46
Uniqueness of the Monster 133
Uniserial module 53
Universal group of type  23 23
Universe 69
Unordered sextet 41
UP1, UP18, UP19 73
UP1, UP2, ... 49ff
UP9 86
Useful permutations 49ff
Useful sextet labelings 48
Venkov, B.B. 93
Weakly closed 104 112
Weakly self orthogonal 26
Weight distribution 38 80
Well balanced sets, 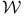 40 40
Weyl groups 91 92
Wilson, Robert 127
Witt index 21 122 131
Witt, Ernst 19 115
x', x'' 102
[12,6,w]-ternary code 80 83
[24,12,8] 27
[24,12,w] 38
[4,2,3] 27 29
[6,3,4] 29
[7,3,4] 27
[8,4,4] 27
[ijkl] 119
{3,4}-transposition group 128 133
|
|
 |
| Реклама |
 |
|
|
 |
|
О проекте
|
|
О проекте



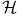

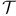 )
) 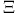 )
) 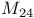
 )
) 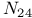

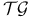 ) on
) on 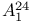 -lattice
-lattice  ,
,  ,
, 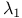 ,
, 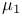 , d,
, d, 
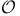 )
) 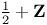
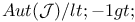
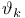
 (5,6,12)
(5,6,12)  ,
,