|
|
 |
| Авторизация |
|
|
 |
| Поиск по указателям |
|
 |
|
 |
|
|
 |
 |
|
 |
|
| Loeve M. — Probability Theory (part 2) |
|
|
 |
| Предметный указатель |
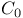 -invariance and continuity criterion 353 -invariance and continuity criterion 353
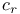 -inequality 157 -inequality 157
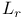 -completeness theorem 163 -completeness theorem 163
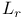 -convergence theorem 164 -convergence theorem 164
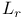 -ergodic criteria 105 -ergodic criteria 105
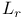 -space(s) 162 -space(s) 162
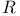 -definition 164 -definition 164
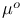 -measurable 88 -measurable 88
 -additive 81 111 -additive 81 111
 -field(s) 59 -field(s) 59
 -field(s), chained 18 -field(s), chained 18
 -field(s), compound 156 235 -field(s), compound 156 235
 -field(s), independent 236 -field(s), independent 236
 -field(s), induced 64 -field(s), induced 64
 -field(s), invariant 79 -field(s), invariant 79
 -field(s), minimal sufficient 13 -field(s), minimal sufficient 13
 -field(s), product 61 62 -field(s), product 61 62
 -field(s), separable 21 -field(s), separable 21
 -field(s), sufficient 11 -field(s), sufficient 11
 -field(s), tail 241 -field(s), tail 241
 -uniform continuity 318 -uniform continuity 318
(sub)Martingale(s) 54 55 194 195
(sub)Martingale(s) central lemma 200
(sub)Martingale(s) closure (sed) 54 200
(sub)Martingale(s) closure theorem 60
(sub)Martingale(s) convergence theorem 59
(sub)Martingale(s) criterion for Brownian motion 244
(sub)Martingale(s) crossings 57 202
(sub)Martingale(s) decomposition 55
(sub)Martingale(s) elementary closure properties 197
(sub)Martingale(s) elementary properties 195
(sub)Martingale(s) extension 206
(sub)Martingale(s) inequalities 57 201
(sub)Martingale(s) preserving transformations 196
(sub)Martingale(s) right closure lemma 197
(sub)Martingale(s) right regularization 206
(sub)Martingale(s) sample limits lemma 202
(sub)Martingale(s) sample limits theorem 203
(sub)Martingale(s) second order 129
(sub)Martingale(s) sequences 62
(sub)Martingale(s) stopping 208
(sub)Martingale(s) suprema theorem 199
(sub)Martingale(s), extended central lemma 208
(sub)Martingale(s), reversed sequences 62 63
(sub)Martingale(s), strong laws of large numbers 66
(sub)Martingale(s), supermartingale 195
(sub)Martingale(s), zero-one laws 64
Abel theorem 400
Absorbing state 314
Addition Property 10
Additive set function 82
Additive set function,  -additive 83 111 -additive 83 111
Additive set function,  -finite 83 111 -finite 83 111
Additive set function, continuity 85
Additive set function, continuity theorem 85
Additive set function, countably 83
Additive set function, decomposition 87
Additive set function, decomposition theorem 88
Additive set function, extension 88
Additive set function, extension theorem 88
Additive set function, finite 83 111
Additive set function, finitely 83
Additive set function, restriction 88
Adherence 66
Adherent point 66
Adjoint space 80
Adjoint,  -endomorphism 334 -endomorphism 334
Alexandrov 190 409
Allard 44
Almost everywhere 112
Almost everywhere, Borel random function 169
Almost everywhere, convergence 114
Almost everywhere, mutual convergence 114
Almost sure(ly) 148
Almost sure(ly), continuous random function 167
Almost sure(ly), convergence 152 248 260
Almost sure(ly), equal random functions 165
Almost sure(ly), ergodic criterion 101
Almost sure(ly), ergodic theorem 100
Almost sure(ly), mutual convergence 153
Almost sure(ly), separability 173
Almost sure(ly), stability 244 249 274 260 124 52
Almost sure(ly), stability criterion 264
Andersen 368 379 404 411
Andersen and Jessen 92 408
Andersen equivalence 377 391
Andersen equivalence lemma 378
Andre, Desire 47 260
Araujo and Le Cam 287
Arcsine law 379 404 278
Arzela — Ascoli theorem 265
Asymptotic behaviour theorem 399
Asymptotic central problem 37
Asymptotic independence 42
Asymptotic passage theorem 36
Asymptotic uniform negligibility 302 47
atom 100 15 22
Attraction, domain of 360
Attraction, partial 403
Attraction, stability and criteria 364
Attraction, standard 402
Austin, Blumenthal and Chacon 387
Axioms of the countable case 16
Axioms of the finite case 8
Bachelier 212 236 386
Backward equation 311
Baire, category theorem 75
Baire, functions 109
Banach 407
Banach space 81
Banach, subspace, invariant 334
Base of a cylinder 62
Base of a linear subspace 126
Base, countable 72
Bawly 302 410
Baxter 369 390 411
Bayes theorem 24
bernoulli 407
Bernoulli case 12 244 280
Bernoulli, extended 26
Bernoulli, law of large numbers 14 244 282 11
Bernstein 407 289
Berry 294 410
Bienayme 408
Bienayme equality 12 246
Billingsley 196 409 264 382 386
Birkhoff 176 385
Birkhoff theorem 76 77
Blackwell 369 411 384
Blumenthal 361 383 386
Blumenthal, zero-one law 248
Bochner 408 409
Bochner theorem 220
Bogoliubov 110
Boltzmann statistics 42—43
Bolzano — Weirstrass property 70
Borel — Cantelli lemma 240
Borel(ian) 107 407 410
Borel(ian), cylinder 93
Borel(ian), field 93 104
Borel(ian), function 111 156
Borel(ian), line 93 107
Borel(ian), progressively— 192
Borel(ian), random function 168 169
Borel(ian), semi-groups 376
Borel(ian), sets 93 104
Borel(ian), space 93 107
Borel(ian), strong law of large numbers 18 19 26 244 11
Borel(ian), zero-one criterion 240
Bose — Einstein statistics 43—44
| Bounded functional 80
Bounded Liapounov theorem 231 282
Bounded q-pair 329
Bounded set 74
Bounded totally 75
Bounded variances case 303
Bounded variances limit theorem 305
Bourbaki 407
Bray 409
Breiman 409 264 384
Breiman lemma 273
Brown(ian) 235
Brown(ian) asymptotic LIT 249
Brown(ian) Brown(ian) Laws of the iterated logarithm 249
Brown(ian) convergence criterion 269
Brown(ian) covariance criterion 238
Brown(ian) diffusion coefficient 237
Brown(ian) drift 237
Brown(ian) embedding lemma 272
Brown(ian) embedding theorem 273
Brown(ian) extrema 239
Brown(ian) extrema equalities 261
Brown(ian) extrema inequalities 243
Brown(ian) extrema laws 279
Brown(ian) first exit times 255
Brown(ian) hitting times 256
Brown(ian) invariance theorem 246
Brown(ian) level sets structure 259
Brown(ian) Lipschitz p-property 247
Brown(ian) local LIT 249
Brown(ian) martingales criterion for 244
Brown(ian) martingales property 239
Brown(ian) motion definition 244
Brown(ian) passage times 284
Brown(ian) process 223
Brown(ian) quadratic variation 253
Brown(ian) reflection principle 260
Brown(ian) sample continuity 243
Brown(ian) sample continuity modulus 247
Brown(ian) second order property 281
Brown(ian) times 254
Brown(ian) times origin invariance 258
Brown(ian) Wald relations 257
Brown(ian), distributed 238
Brown(ian), geometric 281
Brown(ian), reflected 281
Brunk 271 410
C-extension theorem 343
C-invariance and continuity criterion 352
Calculus, second order 135 137 185
Cantelli 20 240 409 410
Cantor theorem 74
Caratheodory extension theorem 88
Category theorem 75
Category, first 75
Category, second 75
Cauchy mutual convergence criterion 74 104 114
Centered sample lemma 218
Centering 244 81
Centering at expectations 244 51
Centering at medians 256 52
Centering function(s) 350 216
Centering lemma 216
Centering, unconditional 240
Central asymptotic problem 37
Central convergence criterion 323 326
Central inequalities 316
Central limit problem 302
Central limit theorem 321 322
Central statistical theorem 20
Chacon 384
Chain 29 31
Chain constant 29 32
Chain, elementary 29 33
Chain, quasi-strongly compact 115
Chain, second order 130
Chain, stationary 39 32
Chained  -fields 18 -fields 18
Chained classes 28
Chained events 28
Chained random variables 29 31
Change of variable lemma 190
Characteristic function(s) 198
Characteristic function(s) and dichotomy 386
Characteristic function(s), composition theorem 226
Characteristic function(s), conditional 26
Characteristic function(s), continuity theorem 204 224
Characteristic function(s), convergence criterion 204
Characteristic function(s), extension theorem 224
Characteristic function(s), general properties 207
Characteristic function(s), infinitely decomposable 306
Characteristic function(s), integral 202
Characteristic function(s), inversion formula 199
Characteristic function(s), self-decomposable 334
Characteristic function(s), stable 338 363
Characteristic function(s), triangular 386
Characteristic function(s), uniform convergence theorem 204
Chung 407 409 410
Chung and Fuchs 368 383 411
Chung and Ornstein 383 411
Class of sets 55
Class, closed under 59
Class, lower 272
Class, monotone 60
Class, upper 272
Classical degenerate convergence criterion 290
Classical limit problem 286
Classical normal convergence criterion 292
Closed class of states 36
Closed linear subspace 126
Closed model 22
Closed on the left (right) (sub)martingales 196
Closed set 66
Closed, strongly 107
Closed, under 59
Closed, weakly 107
Closure theorem(s), covariances 134
Closure theorem(s), infinitely decomposable laws 309
Closure theorem(s), submartingales 60
Cogburn and Tucker quadratic variation 283
Combinatorial lemma 378
Combinatorial method 47
Compact locally 71
Compact set 69
Compact space 69
Compact, strongly (quasi-) 107 110 115
Compact, weakly (quasi-) 107 110 115
Compactification 71
Compactness theorem for distribution functions 181
Compactness theorem for metric spaces 76
Compactness theorem for separated spaces 70
Compactness, properties 70
Compactness, relative 195
Compactness, relative—criterion 195
Comparison theorem for, convergences 117
Comparison theorem for, laws of sums 41
Comparison theorem for, lemma 303 320
Complement 4 56
Complete Complete convergence criterion 204
Complete convergence 180
Complete equivalence 39
Complete equivalence criterion 40
Complete measure 91
Complete metric space 74
Completeness theorem, 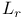 - 163 - 163
Completion of  -fields 91 -fields 91
Completion of metric spaces 77
Complex random variable(s) 154 126
composition 204 283
Composition and decomposition theorem 283
|
|
 |
| Реклама |
 |
|
|
 |
|
О проекте
|
|
О проекте



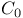 -invariance and continuity criterion
-invariance and continuity criterion 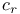 -inequality
-inequality 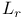 -completeness theorem
-completeness theorem 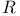 -definition
-definition 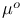 -measurable
-measurable  -additive
-additive  -endomorphism
-endomorphism