|
|
 |
| Авторизация |
|
|
 |
| Поиск по указателям |
|
 |
|
 |
|
|
 |
 |
|
 |
|
| Kuttler K.L. — Modern Analysis |
|
|
 |
| Предметный указатель |
 functions 68 functions 68
 sets 123 sets 123
 34 34
 sets 123 sets 123
 140 140
 , sequential compactness 244 , sequential compactness 244
 , compactness in 367 , compactness in 367
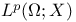 485 485
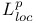 359 359
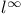 215 215
 net 17 net 17
 locally finite basis 541 locally finite basis 541
Alexander subbasis theorem 98
Alexandrov’s theorem 520
Algebra of sets 123
Analytic function 546
Apriori estimates 110
Area formula 398
Ascoli — Arzela theorem 23
Axiom of Choice 1—2 169
Axiom of extension 1
axiom of specification 1
Axiom of unions 1
Banach space 205
Banach space, interpolation 469
Banach — Alaoglu theorem 100
Banach — Steinhaus theorem 36
Basic open set 5
Basis 5
Besicovitch covering theorem 263
Bessel potentials 472
Bochner integrable 482
Borel measurable 132 169
Borel measure 154
Borel regular 381
Borel sets 123
Borel — Cantelli lemma 133
Bounded continuous linear functions 35
Brouwer fixed point theorem 557
Calderon — Zygmund decomposition 438
Cantor diagonal process 31
Cantor function 169
Cantor set 169
Caratheodory 149
Caratheodory’s criterion 378
Caratheodory’s procedure 150
Cauchy Schwarz inequality 49
Cauchy sequence 7
Ceasaro mean 61
Central limit theorem 338
Chain rule 64
Change of variables 177 433
Change of variables 1—1 transformation 257
Change of variables general case 259
Characteristic function 135 305
Chi square 348
Clarkson’s inequality 216
Closed graph theorem 39
Closed set 6
Closure of a set 7
Coarea formula 427
Coercive 527
Combinations of measurable functions 130
Compact set 8
Complete measure space 150
Complete metric space 7
Completely separable 7
Completion of a measure space 170
Completion of product measures 196
Conjugate function 509
Continuous function 8
Convergence in measure 133
Convex function 503
Convex functions 214
Convex sets 91
Convolution 212 297
Covariance 305
derivatives 64
Differentiability monotone functions 261
Differential equations 552
Differential equations, global existence 109 121
Differential equations, Peano existence theorem 85
Differential inclusion 528
Dirichlet kernel 47
distribution 355
Distribution function 200 259 436
Divergence theorem 411
Dominated Convergence Theorem 143 487
Dual space 43
Duality maps 47
Eberlein — Smulian theorem 104
Egor off theorem 131
Elementary sets 189
Equality of mixed partial derivatives 70
Equivalence of norms 55
Expected value 304
Fatou’s Lemma 142
Fejer kernal 61
Fisher Neyman criterion 352
Fourier series 46
Fourier transform 295
Fourier transform  299 299
Fourier transforms 283
Fubini’s Theorem 176 192 198
Fundamental theorem of calculus, general Radon measures 271
Fundamental theorem of calculus, Lebesgue measure 249
Gamma function 214 376
Gauge function 40
Graves theorem 82
H lder’s inequality 203 lder’s inequality 203
Hahn Banach theorem 41
Hardy Littlewood maximal function 247
Hardy’s inequality 214
Hausdorff and Lebesgue measure 384
Hausdorff dimension 384
Hausdorff maximal principle 40
Hausdorff maximal theorem 529
Hausdorff measures 378
Hausdorff space 6
Heine — Borel theorem 20
Helly’s lemma 56
Helly’s selection theorem 351
Higher order derivatives 68
Hilbert space 49
Hormander condition 440
Implicit function theorem 77
Inner product space 49
Inner regular measure 154
Inverse function theorem 71 84
Isodiametric inequality 371 375
Isometric 494
Iterated integrals 189
James map 44
Jensens inequality 214
Laplace transform 187
Laplace transforms 244
Lebesgue decomposition 241
Lebesgue integral 136 140
Lebesgue measure 171
Lebesgue measure, translation invariance 173
Lebesgue number 251
Lebesgue set 250
Lebesgue Stieltjes measure 168
lim inf 129
lim sup 129
Limit point 6
Lipschitz maps, extension 387
| Locally compact 8
Locally convex topological vector space 87
Locally finite 535
Marcinkiewicz interpolation 437
Maximal function measurability 259
Maximal function strong estimates 260
Maximal function, general Radon measures 268
McShane’s lemma 233
Mean 305
Measurable 149
Measurable function 127
Measurable rectangle 189
Measurable representative 490
Measurable sets 126 149
Measure space 126
Metric space 7
Metrizable space 541
Mihlin’s theorem 452
Milman’s theorem 58
Minkowski functional 47 56 92
Minkowski’s inequality 206
Moment generating function 305
Monotone class 124
Monotone Convergence Theorem 136
Morrey’s inequality 361
Multi-index 283
Nagata — Smirnov metrization theorem 544
Nonmeasurable set 169
Normal topological space 6
Nowhere differentiable functions 46
One point compactification 13
Open cover 8
Open mapping theorem 37
Open sets 5
Orthonormal set 60
Outer measure 133 149
Outer regular measure 154
Paracompact space 535
Parallelogram identity 60
Parseval’s Inequality 60
Partial order 40
Partially ordered set 529
Partition of unity 155 539 541
Pettis theorem 478
Plancherel theorem 289
Point of density 259
Pointwise limits of measurable functions 128
polar 509
Polar coordinates 183
Polar decomposition 224
Positive linear functionals 156
Power set 5
Precompact 30
Product measure 191
Product measures and regularity 193
Product rule 361
Product topology 8
Projection in Hilbert space 51
Proper function 503
Pseudomonotone operator 113
Quotient space 469
Rademacher’s Theorem 363
Radon measure 156
Radon Nikodym property 491
Radon Nikodym Theorem,  finite measures 220 finite measures 220
Radon Nikodym Theorem, finite measures 219
Random variable, chi square 348
Random vector, characteristic function 305
Random vector, distribution function 304
Random vector, independent 309
Random vector, moment generating function 306
Random vector, normal 335
Random vectors 303
Rao — Blackwell theorem 352
Refinement of a cover 535
Reflexive Banach space 45
Reflexive space, closed subspace of 300
Regular measure 154
Regular topological space 6
Riemann integrable 168
Riemann Stieltjes integral 168
Riesz representation theorem 496
Riesz representation theorem  , ,  finite case 230 finite case 230
Riesz representation theorem  , finite measures 226 , finite measures 226
Riesz representation theorem  , non , non  finite measure space 237 finite measure space 237
Riesz representation theorem  , positive linear functionals 156 , positive linear functionals 156
Riesz representation theorem for  , finite measures 229 , finite measures 229
Riesz Representation theorem,  239 239
Riesz representation theorem, 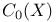 240 240
Riesz representation theorem, Hilbert space 52
Riesz representation theorem, locally compact Hausdorff space 164
Sard’s lemma 256
Schaefer fixed point theorem 121
Schauder fixed point theorem 108
Schroder Bernstein theorem 2
Schwartz class 283
Seminorms 87
Separable space 7
Separation theorem 47
Sequential weak* compactness 103
Shannon sampling theorem 301
Sigma algebra 123
Sigma compact topological space 155
Sigma finite 189
Simple function 135 475
Sobolev Space, embedding theorem 300 368
Sobolev Space, equivalent norms 300
Sobolev Space, extension map 416
Sobolev Space, reflexive space 367
Sobolev Space, trace map 415
Steiner symetrization 373
Stone’s theorem 537
Strict convexity 48
Strongly measurable 475
Subbasis 9
Sufficient statistic 314
Support of a function 154
Surface measure 402
Taylor’s Formula 545
Tempered distributions 293
Tietze extention theorem 31
Topological space 5
Topological vector space dual 89
Total variation 222
Totally bounded set 17
Totally ordered set 529
Triangle inequality 142
Tychonoff fixed point theorem 107
Tychonoff theorem 100
Uniform boundedness theorem 36
Uniform convexity 48 58
Uniformly convex 216
Uniformly integrable 145
Urysohn’s lemma 9
Variational inequality 51
Vector measures 222 490
Vitali convergence theorem 215
Vitali covering theorem 246
Vitali coverings 260
Weak * convergence, Young’s measures 277
Weak convergence 48
Weak derivative 356
Weak topology 98
Weak* measurable 480
Weak* topology 98
Weakly measurable 475
Well-ordered sets 531
Young’s inequality 242
|
|
 |
| Реклама |
 |
|
|
 |
|
О проекте
|
|
О проекте



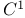 functions
functions 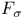 sets
sets 
 sets
sets 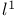
 , sequential compactness
, sequential compactness  , compactness in
, compactness in 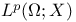
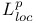
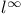
 net
net  locally finite basis
locally finite basis  lder’s inequality
lder’s inequality