Авторизация
Поиск по указателям
Behnke H., Bachmann F., Fladt K. — Fundamentals of mathematics. Volume III. Analysis
Обсудите книгу на научном форуме Нашли опечатку?
Название: Fundamentals of mathematics. Volume III. AnalysisАвторы: Behnke H., Bachmann F., Fladt K.Аннотация: Fundamentals of Mathematics represents a new kind of mathematical publication. While excellent technical treatises have been written about specialized fields, they provide little help for the nonspecialist; and other books, some of them semipopular in nature, give an overview of mathematics while omitting some necessary details. Fundamentals of Mathematics strikes a unique balance, presenting an irreproachable treatment of specialized fields and at the same time providing a very clear view of their interrelations, a feature of great value to students, instructors, and those who use mathematics in applied and scientific endeavors. Moreover, as noted in a review of the German edition in Mathematical Reviews , the work is "designed to acquaint [the student] with modern viewpoints and developments. The articles are well illustrated and supplied with references to the literature, both current and ‘classical’".
Язык: Рубрика: Математика /Статус предметного указателя: Готов указатель с номерами страниц ed2k: ed2k stats Год издания: 1984Количество страниц: 557Добавлена в каталог: 01.12.2013Операции: Положить на полку |
Скопировать ссылку для форума | Скопировать ID
Предметный указатель
Local density 453 Local parameter 231 234 Local properties 453 Local theory 439 Local uniformizing parameter 244 Local, meaning of 439 Locally compact topological space 514 Locally countable 453 Logarithm function 22 40 Logarithms, natural 38 Looman — Menchoff theorem 208 Lower limit 8 Luzin, N. 466 M-neighborhood 449 MacLane 271 Majorant (minorant) functions 328 Majorant series 441 Majorizing sequence 8 Manifolds, characteristic 309 311 321 322 337 344 Manifolds, complex 272 Manifolds, differentiable 184—186 513 Manifolds, discriminant 282 Manifolds, orientable 140 185 Manifolds, Riemannian 124—125 184—186 Manifolds, two-dimensional 231 245n Mapping 23 26 41 42 Mapping, angle-preserving 210 Mapping, biholomorphic 258 Mapping, complete 424 Mapping, conformal 210 325 Mapping, continuous 24 455 Mapping, contractive 423 424 Mapping, defined by holomorphic functions 209 Mapping, equiform 194 Mapping, extension of 449 Mapping, fixed point of 423 Mapping, function as 215 Mapping, homogeneous 201 Mapping, homogeneous linear 45 Mapping, linear 516 Mapping, locally univalent 209 Mapping, open 455 Mapping, projection 239 Mapping, scale-preserving 209 Mapping, schlicht 209 Mapping, segment-preserving 209 Mapping, sense-preserving conformal 211 Mapping, sense-reversing conformal 211 Marginal distribution function 97 Marginal probability distribution 97 Mathematical logic 509 Matrix, left reciprocal 406 Maximum principle, for functions of a complex variable 226 Maximum, of two functions 54n Maximum-minimum principle 327 328 Maxwell equations 307 Maxwell, J.C. 89 Mean density 497 Mean of k 102 Mean-value theorem 30 Measurability, of functions 66 Measurable function 93 Measurable set 63 Measurable space 93 Measure 53 67 Measure 83 Measure and integration, theory of 77 Measure, theory of 77 Mechanical system 416 Medium, of function 74 mellin 501 Membrane, vibrations of 307 Meromorphic continuation 241 Meromorphic differential 234 Meromorphic function in complex plane 226—229 Meromorphic function, algebraic equation for 248 Meromorphic function, algebraic function field of 248 Meromorphy 228 Metric spaces 11—12 423 513 MIN 486 minimax 411 Minimum, of two functions 54n Minorant (majorant) functions 328 Minorizing sequence 8 Mirror image 193 Mitrovic, D. 494 Mixed structure 522 Model 508 Modification, concept of 275 Modification, meromorphic 275 Modification, proper continuous 275 Modification, theory of vi 253 Module 519 Module of sets 452 Modulus problem 250n Moebius strip 262 Molecule 418 moment 102 Moment, "central" 102 Moment, first 102 Momentum coordinates 416 Monoid 524 Monotone increasing (decreasing) 7 Monotone limits 58 Monotone sequences 7—8 Monotone sequences, fundamental theorem on 7 Montgomery 517 Moore — Smith convergence 526 Moore — Smith sequences v 2 12—13 14 15 Motion 42 Motion of electron 417 Motion, hyperbolic 194 Mountain 43 mu*-equivalence 70 mu-integral 71 mu-upper integral 69 Multidimensional normal distribution 97 Multiple integrals vi Multiple integrals, laws for 125 Multiplication, alternating 151 153 Multiplication, continuity of 7 Multiplication, exterior 151 155 Multiplicity, of zero 195 n-dimensional space, functions in 42—52 Nabla operator 180 Natural number 524 Neighborhood 3 20 429 Neighborhood and compactification 264—266 Neighborhood filter 14 Neighborhood set 198 Neighborhood, concept of 196 Neighborhood, coordinate 184 Neighborhood, deleted 225 Neighborhood, elementary 196 Neighborhood, system of 263 Neumann series 428 Noether, Emmy 510 512 Non-Borel set 458 Non-Euclidean motion 194 Nonanalytic segment, of surface 325 Nonhomogeneous differential equation 286 Nonlinear differential equation 518 Nonorientability 270 Norm 69 190 396 Normal form 323 Normal form of differential form 156 Normalization 398 Normed algebra 516 Normed space 69n North pole 263 Novikov independence theorem 471 475 Novikov separation theorem 472 Number circle 498 Number sphere 262 Number theory 499 500 Number triples, equivalence class of 259 Number triples, singular equivalence class of 260 Number, cardinal 514 Number, complex 188—191 Number, hypertranscendental 493 Number, natural 524 Number, revolution 429 432 Number, transcendental 491 Numerical sequence, limit of 197 Open kernel 64n Open set 18 197 Operator 392 Operator equations 423 434—437 Operator in a domain 415 Operator, adjoint 403 Operator, completely continuous 341 342 413 Operator, domain of 524 Operator, energy 417 Operator, groups with 511 Operator, integral representation of 415 Operator, linear 400 Operator, nabla 180 Operator, self-adjoint 408 413—418 Operator, solution 346 Operator, star 159 Operator, symmetric 341 Operator, unbounded 415 Ordered pair 17 Ordered set 521 Ordinary differential equations vi Ordinary point 239 Orientability 268 Orientable 185 268 Orientable manifold 140 185 Orientable surface-segment 137 Orientation 126 Orientation, induced 132 140 Orientation, opposite 126 Oriented boundary 126 Oriented Riemann surface 246 Oriented surface-segment 134 Orthogonality 398 Orthonormal system 398 412 442 Orthonormal system, complete 398 Orthonormality 168 186 Oscillation, forced 302 Oscillation, free 302 Oscillation, theorem of 298 Osgood space 273 Outer composition 524 p-adic numbers 519 Pappus and Pascal theorem 254 255 270 Parabolic curves 325 Parallel lines 252 Parallel lines, equivalence class of 259 PARAMETER 126 Parameter planes 244 Parameter, global uniformizing 250 Parameter, local uniformizing 244 271 Parameter, variation of 289 Parametric representation, of arc 126 Parseval equation 404 Partial derivative 43 Partial derivative of distribution 85 Partially ordered 55n Partition 54 Partition of integral 13 Pascal and Pappus theorem 254 255 270 Paths 234 Paths, bounding system of 217 Paths, simple 219 Paths, simple closed 220 PCA-set 471 PCPCA-set 471 Peano theorem 290 Peano — Jordan content 62 Peano, G. 508 Peano, G., axioms of 518 Peano, G., convergence theorem of 278 Periodic solution 302 Perpendicular 193 Perron, O. 326 328 336 338 Perturbation 296 312 Perturbed differential equation 302 Pfaffian form 145 156 160 168 Pfaffian form, orthonormal basis of 170 186 Phase plane 303 Phi-function 358 PI 351n 491 Picard — Lindeloef method of iteration 279 Picard — Lindelof method 279 Piecewise smooth curves 130 Piecewise smooth surfaces 130 Planck quantum of action 417 Plane triadic set 477 Plane, closing of 266—271 Plane, Euclidean 176 192 259 262—263 Plane, infinitely distant 273 Plane, nonorientable 270 Plane, parameter 244 Plane, projective 259—262 Poincare lemma, first 160 164 Poincare lemma, first, second 161 164 176 Point 3 Point at infinity 252 259 260 Point of accumulation 196 Point of continuity 461 Point spectrum 413 Point, branch 210 239 Point, continuity at 454 Point, double 130 Point, end 126 Point, hyperinfinitely distant 274 Point, ideal 227 Point, initial 126 Point, isolated 198 Point, limit 11 196 198 Point, neighborhoods of 230 Point, open set of 197 Point, ordinary 239 Point, oriented 126 Point, singular 282 Point, usefulness of 253—259 Poisson distribution 96 122 Poisson, S.D. 89 Pole 235 Pole of a differential 235 Position coordinates 416 potential energy 416 Potential equation 306 314 Potential-theoretic method, for elementary functions 249 Power 446 Power series 34—35 215 229 Power series and Cauchy integrals 222 Power series, derivatives of 216 Preservation of domains, theorem on 226 Prime ideal 517 Prime number theorem 482 Prime number theorem, consequences of 484—485 Prime number theorem, elementary proofs of 489 Prime number theorem, second 487 488 Primes, Bertrand — Cebysev theorem 483 Primes, Bohr — Landau theorem 486 Primes, Cudakov theorem 485 Primes, Dirichlet theorem 488
Реклама
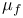, density of 
|
|
О проекте