|
|
 |
| јвторизаци€ |
|
|
 |
| ѕоиск по указател€м |
|
 |
|
 |
|
|
 |
 |
|
 |
|
| Taylor J.R. Ч An Introduction to Error Analysis: The Study of Uncertainties in Physical Measurements |
|
|
 |
| ѕредметный указатель |
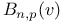 , binomial distribution 230 (see also Binomial distribution) , binomial distribution 230 (see also Binomial distribution)
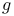 , measurement with pendulum 68Ч69 88 , measurement with pendulum 68Ч69 88
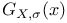 , Gauss function 133 (see also Normal distribution) , Gauss function 133 (see also Normal distribution)
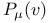 , Poisson distribution 246 (see also Poisson distribution) , Poisson distribution 246 (see also Poisson distribution)
 , correlation coefficient 216Ч217 (see also Correlation coefficient) , correlation coefficient 216Ч217 (see also Correlation coefficient)
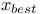 estimate for estimate for  14 14
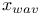 , weighted average 175 , weighted average 175
 , uncertainty in , uncertainty in  14 (see also Uncertainty and Error 14 (see also Uncertainty and Error
 , width of Gauss distribution 131 (see also Normal distribution) , width of Gauss distribution 131 (see also Normal distribution)
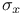 , covariance of , covariance of  and and 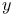 212 (see also Covariance) 212 (see also Covariance)
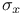 , standard deviation 99Ч101 (see also Standard deviation) , standard deviation 99Ч101 (see also Standard deviation)
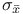 , standard deviation of mean 102 (see also Standard deviation of mean) , standard deviation of mean 102 (see also Standard deviation of mean)
Absolute uncertainty 29
Absolute zero of temperature 190
Absorption coefficient and photon energy 84
Acceleration of cart on slope 71Ч73 89
Accepted value 18
Accepted value, compared with measured value 18Ч20
Addition in quadrature 58
Addition in quadrature, can give smaller answer 62
Addition in quadrature, for independent random errors 57Ч62
Addition in quadrature, justification of 141Ч146
Adjusted chi squared 283 (see also Chi squared)
ArchimedesТ problem 5Ч6
Area of a rectangle 104Ч105 115
Average see Mean and Weighted average
Average deviation 99
Background events, subtraction of 254Ч255
Bar histogram 124
Bell-shaped curve 129Ч130 (see also Normal distribution)
Bending of light by sun 7
Best estimate, 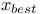 14 14
bin 124
Bin histogram 125
Bin, choice of 125Ч126 262 266Ч268 272
Binomial coefficient 229
Binomial distribution 228Ч235
Binomial distribution, compared with Gaussian 232Ч235
Binomial distribution, definition 230
Binomial distribution, mean for 231 242
Binomial distribution, standard deviation of 232
Binomial distribution, symmetry when 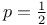 232 232
Binomial expansion 230
Binomial series 52 80
Bubble chamber 223
Cart on slope 71Ч73 89
ChauvenetТs criterion 166Ч169
ChauvenetТs criterion, to reject several data 169
Chi squared, 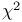 266 268 266 268
Chi squared, adjusted 283
Chi squared, as indicator of agreement 264
Chi squared, per degree of freedom 271
Chi squared, probabilities for 271Ч273
Chi squared, reduced, 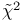 271 271
Chi squared, table of probabilities for 292Ч293
Chi-squared test 261Ч278
Chi-squared test, for dice experiment 267Ч268 275
Chi-squared test, for Gauss distribution 261Ч265 274Ч275
Chi-squared test, for Poisson distribution 276Ч277
Coefficient of correlation see Correlation coefficient
Coefficient of determination 217 (see also Correlation coefficient)
Coefficient of expansion 59
Comparison, of measured and accepted values 18Ч20 149Ч151
Comparison, of two measured numbers 20Ч23 149Ч151
Compensating errors 74
Confidence level for uncertainty 149 (see also Significance level)
Consistent measurements 173
Constraints 269Ч270
Correlation coefficient 216Ч217
Correlation coefficient, probabilities for 219Ч220
Correlation coefficient, table of probabilities for 290Ч291
correlation, negative 218
Correlation, of I.Q. 225
Correlation, significant 220
Cosmic rays 253
Counting experiments, uncertainty in 48Ч49 249
Covariance 212
Covariance, and correlation coefficient 216Ч217
Covariance, in error propagation 211Ч215
Critical angle 63
Decay, radioactive 245
Definition, problems of 4 46
Degrees of freedom 188 269Ч271
Density of gold 5
Determination, coefficient of 217 (see also Correlation coefficient
Deviation 98
Deviation, average 99
Deviation, mean of is zero 111 (see also Standard deviation
Dice experiments 228
Dice experiments, and chi-squared test 267Ч268 275
Difference of measured numbers 22Ч24 41Ч42 49Ч50 60
Digital displays 47
Discrepancy 16Ч18
Discrepancy, significant and insignificant 17Ч18 150Ч151
Discrete distributions 232
distribution 123
Distribution, binomial see Binomial distribution
Distribution, discrete 232
Distribution, exponential 155 158
Distribution, Gauss see Normal distribution
Distribution, limiting see Limiting distribution
Distribution, Lorentzian 266
Distribution, normal see Normal distribution
Distribution, parent 126
Distribution, Poisson see Poisson distribution
Distribution, universe 126 (see also Limiting distribution)
Dividing measured numbers 51Ч53 61
Door, measurement of 3Ч4
Erf(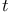 ) see Normal error integral ) see Normal error integral
Error 3 14
Error bar 5 25Ч27
Error function see Normal error integral
Error of the mean 102 (see also Standard deviation of mean)
Error propagation 45Ч91 209Ч215
Error propagation, covariance in 211Ч215
Error propagation, for function of one variable 63Ч65
Error propagation, for function of several variables 74Ч77
Error propagation, for independent errors 57Ч62
Error propagation, for powers 55Ч56 66
Error propagation, for products and quotients 51Ч54 61
Error propagation, for sums and differences 49Ч50 60
Error propagation, general formula 73Ч77
Error propagation, proof of general formula 146
Error propagation, step-by-step 66Ч68
Error propagation, upper bound for 214Ч215
Error propagation, with graphs 63 84
Error, compensating 74
Error, in target practice 95Ч96
Error, probable 137
error, random 94Ч97
Error, systematic 11 94Ч97 106Ч109
Error, true 18 (see also Uncertainty)
Exponential distribution 155 158
Extrapolation 192
Factorial function, 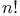 229 229
Fractional uncertainty 28Ч31
Fractional uncertainty, and significant figures 30Ч31
Fractional uncertainty, in products 31Ч34 (see also Uncertainty)
Full width at half maximum 156
Function of one variable, uncertainty in 65
Function of several variables, uncertainty in 75
FWHM 156
Gauss distribution see Normal distribution
Gauss function 133 (see also Normal distribution)
Gaussian approximation, to binomial distribution 232Ч235
Gaussian approximation, to Poisson distribution 250Ч251
General relativity, test of 7
Gold, density of 5
graphs 24Ч28
Graphs, and error propagation 63 84
| Graphs, error bars on 25Ч27 39
Graphs, slope of 25 40
Graphs, straight-line 24Ч27 181Ч182
Half width at half maximum 156
Highly significant test (1%) 237 272
histogram 124
Histogram, bar 124
Histogram, bin 125
HWHM 156
Hypotheses 237 238
Hypotheses, null 237
Hypothesis testing 236Ч240
Inconsistent measurements 173
Independent errors, propagation of 57Ч62
Interpolation 9
Least squares 174 (see also Least-squares fit to a line and Least-squares fit to curves)
Least-squares fit to a line 182Ч192
Least-squares fit to a line, estimates for 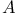 and and 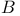 184 184
Least-squares fit to a line, line through origin 200 204
Least-squares fit to a line, uncertainty 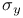 186Ч188 186Ч188
Least-squares fit to a line, uncertainty in 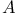 and and 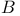 188 188
Least-squares fit to a line, weighted 201 204
Least-squares fit to a line, when both  and and 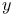 are uncertain 188Ч190 are uncertain 188Ч190
Least-squares fit to curves 193Ч196
Least-squares fit to curves, exponential 194Ч196
Least-squares fit to curves, polynomial 193Ч194
Light, bending by sun 7
Limiting distribution 126Ч129 227
Limiting distribution, as probability 128
Limiting distribution, mean of 129
Limiting distribution, normalization of 128
Limiting distribution, standard deviation of 129 (see also Distribution)
Line of regression 184
Linear correlation see Correlation coefficient
Linear graphs 24Ч27 181Ч182
Linear graphs, slope of 25 40
Linear regression 182 (see also Least-squares fit to a line)
Linearization 194
Lorenzian distribution 266
Margin of error 14 (see also Uncertainty)
Maximum likelihood see Principle of maximum likelihood
Mean, as best estimate 97Ч98 137Ч139
Mean, of binomial distribution 231 242
Mean, of limiting distribution 129
Mean, of normal distribution 134
Mean, of Poisson distribution 247
Mean, standard deviation of see Standard deviation of mean
Method of least squares 174 (see also Least-squares fit to a line and Least Ч Squares fit to curves)
MillikanТs measurement of e 109 116
Multiple regression 196Ч197
Multiplying measured numbers 31Ч34 53 61
negative correlation 218
Nonparametric tests 238
Normal density function 131
Normal distribution 129Ч135
Normal distribution, as limit of binomial 232Ч236
Normal distribution, as limit of Poisson distribution 250Ч251
Normal distribution, chi-squared test for 261Ч265 274Ч275
Normal distribution, compared with binomial 232Ч235
Normal distribution, compared with Poisson 250
Normal distribution, definition 133
Normal distribution, mean of 134
Normal distribution, normalization of 132
Normal distribution, points of inflection of 157
Normal distribution, proof of for random errors 235Ч236
Normal distribution, standard deviation of 134
Normal distribution, width parameter of 131
Normal equations 184 194 197
Normal error function 131
Normal error integral 136Ч137
Normal error integral, tables of 286Ч289
Normalization condition 124 128
Normalization condition, for normal distribution 132
Normalization condition, for Poisson distribution 257
Normally distributed measurements 133
Null hypothesis 237
One-tailed probability 239
Opinion poll 238Ч239
Parallax 97
Parent population 126 (see also Limiting distribution)
Partial differentiation 74 90
Partial uncertainty 76
Pendulum 68Ч69 88
Percent uncertainty 29 (see also Fractional uncertainty)
photoelectric effect 225
Points of inflection of Gauss function 157
Poisson distribution 245Ч260
Poisson distribution, approximate symmetry for  large 250 large 250
Poisson distribution, as limit of binomial 246
Poisson distribution, chi-squared test for 276Ч277
Poisson distribution, compared with Gaussian 250Ч251
Poisson distribution, definition 246
Poisson distribution, for cosmic rays 253
Poisson distribution, mean of 247
Poisson distribution, normalization of 257
Poisson distribution, standard deviation of 249
Polynomial regression 194
Population standard deviation 100
PRECISION 28 (see also Fractional uncertainty)
Principle of maximum likelihood 139
Principle of maximum likelihood, applied to Poisson distribution 257 258
Principle of maximum likelihood, in least-squares fits 182 202
Principle of maximum likelihood, in weighted averaging 174
probability distribution 227 (see also Limiting distribution)
Probability, one- and two-tailed 239
Probable error 137
Problems of definition 4 46
Product of measured numbers 31Ч34 42Ч43 53 61
Propagation of errors see Error propagation
Quadratic sum 58 (see also Addition in quadrature)
Quotient of measured numbers 51Ч53 61
Radioactive decay 245
Random component of error 106
Random errors 94Ч97
Random errors, can become systematic 117
Reduced chi squared, 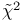 271 (see also Chi squared) 271 (see also Chi squared)
Refractive index, from critical angle 63
Refractive index, using SnellТs law 69Ч70 89
Regression, line of 184
Regression, linear 182
Regression, multiple 196Ч197
Regression, polynomial 194 (see also Least-squares fit to a line and Least-squares fit to curves)
Rejection of data 165Ч169
Relative uncertainty 28 (see also Fractional uncertainty)
Relativity, test of 7
Residual 98 (see also Deviation)
RMS deviation 99
Sample standard deviation 100
Sample standard deviation as best estimate for width  296 296
Scatter plot 216
Schwarz inequality 214 224
SD see Standard deviation
SDOM (see also Standard deviation of mean)
Sigma notation,  98 98
Significance level 238 272
Significant correlation 220
Significant discrepancy 151
Significant figures 14Ч16 30
Significant figures, and fractional uncertainty 30Ч31
Significant figures, in products 42Ч43
Significant figures, need to keep extra 16
Significant test (5%) 237 272
simple pendulum 68Ч69 88
Ski wax, tests of 236Ч238
SnellТs law 69Ч70 89
spreadsheets 112 179 202
Spring constant,  101Ч103 105Ч106 200 101Ч103 105Ч106 200
Square root, uncertainty in 66
Square-root rule 48 249
Standard deviation 98Ч101
|
|
 |
| –еклама |
 |
|
|
 |
|
ќ проекте
|
|
ќ проекте



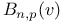 , binomial distribution
, binomial distribution 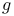 , measurement with pendulum
, measurement with pendulum 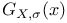 , Gauss function
, Gauss function 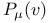 , Poisson distribution
, Poisson distribution  , correlation coefficient
, correlation coefficient 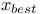 estimate for
estimate for 
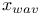 , weighted average
, weighted average  , uncertainty in
, uncertainty in  , width of Gauss distribution
, width of Gauss distribution 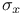 , covariance of
, covariance of 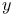
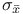 , standard deviation of mean
, standard deviation of mean 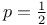
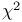
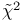
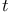 )
) 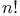
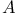 and
and 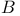
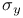
 large
large 
