|
|
 |
| Авторизация |
|
|
 |
| Поиск по указателям |
|
 |
|
 |
|
|
 |
 |
|
 |
|
| Dunford N., Schwartz J.T. — Linear operators. Part III. Spectral operators |
|
|
 |
| Предметный указатель |
Spectrum, of a spectral operator XV.8 (1954)
Spectrum, of a spectral operator, condition to be in point spectrum XV.15.13 (2076)
Spectrum, of a spectral operator, continuous spectrum, definition of XV.8.1 (1955)
Spectrum, of a spectral operator, examples of XV.15.37 XV.15.38 XV.15.39
Spectrum, of a spectral operator, point spectrum, definition of XV.8.1 (1955)
Spectrum, of a spectral operator, residual spectrum, definition of XV.8.1 (1955)
Spectrum, of a vector, definition of XV.2.6 (1931)
Spectrum, of a vector, examples of XV.15.39 XV.15.40
Spectrum, of a vector, properties of XV.3.3 XV.3.4 XV.3.6 XV.3.7
Spitzer, F. 2103
Srinivasan, T. P. 2129
Stampfli, J. G. 2089 2090 2105 2106 2107.2128.2176.2511
Stankevi , I. V. 2374 2511 , I. V. 2374 2511
Ste enko, V. Ja. 2131 2132 enko, V. Ja. 2131 2132
Steckloff, W. 2371
Stesichorus 2055
Stone, M. 2225
Stone’s theorem on extremally disconnected spaces XVII.5.16 (2225)
Strassmaier, J. N. 2059
Straus, A. V. 2374
Sturm, J. C. F. vi xii
Subscalar operators XV.16 (2095-2096)
Suciu, I. 2117 2129
Support, of a spectral measure XV.9 (1965)
Suzuki, N. 2090 2122 2128
Sz.-Nagy, B. 2098 2122 2128 2129 2176 2226 2510
T g g , S. 2133 , S. 2133
Tamarkin, J. D. xii 2371 2372
Taylor, A. E. 2083 2090
Taylor, B. vi
Tempered distribution in 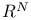 , complex conjugate of XV.12.14 (2032) , complex conjugate of XV.12.14 (2032)
Tempered distribution in 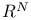 , definition of XV.12.9 (2028) , definition of XV.12.9 (2028)
Tempered distribution in 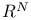 , derivations of XV.12 (2031-2032) , derivations of XV.12 (2031-2032)
Tempered distribution in 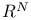 , determined by a function XV.12.10 (2029) XV.12.11 XV.12.12 XV.12.13 , determined by a function XV.12.10 (2029) XV.12.11 XV.12.12 XV.12.13
Tempered distribution in 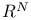 , Fourier transform and other properties of XV.12 (2029) , Fourier transform and other properties of XV.12 (2029)
Thoe, D. 2511
Thompson, A. C. 2131
Thorp, E. 0. 2132
Tillmann, H. G. 2090 2117 2122 2128 2175
Titchmarsh, E. C. 2372
Tomiuk, B. J. 2131
Trampus, A. 2090
Turner, R. E. L. 2090 2371 2374 2376 2448 2511
Type 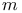 , conditions for XV.5.4 (1944) XV.6.7 XV.6.7 XV.7.7 XV.10.13 XV.16 XVI.5.18 , conditions for XV.5.4 (1944) XV.6.7 XV.6.7 XV.7.7 XV.10.13 XV.16 XVI.5.18
Type 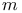 , definition of XV.5.3 (1943) , definition of XV.5.3 (1943)
Type 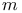 , examples of XV.10.13 (1982) XV.11.21 XV.11 XV.12 (15) (2017) XV.12 (2020) XV.12 (2026) XV.15.57 XV.15.58 , examples of XV.10.13 (1982) XV.11.21 XV.11 XV.12 (15) (2017) XV.12 (2020) XV.12 (2026) XV.15.57 XV.15.58
Type 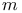 , point spectrum of XV.8.3 (1956) , point spectrum of XV.8.3 (1956)
Type 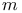 , residual spectrum of XV.8.3 (1956) , residual spectrum of XV.8.3 (1956)
Tzafriri, L. 2089 2090 2100 2225 2226 2511
Unbounded operators, matrices of measurable functions as XV.12.2 (2013)
Unbounded operators, special case, definition of XV.12 (2011) XV.12.1
Unbounded scalar type operators, convergent sequences of XVIII.2.18 (2244)
Unbounded scalar type operators, definition of XVIII.2.12 (2241)
Unbounded scalar type operators, measurable functions of XVIII.2.10-11 (2238) XVIII.2.17
Unbounded scalar type operators, resolution of the identity for XVIII.2.12-14 (2241-2242)
Unbounded spectral operators, analytic functions of XVIII.2.8-9 (2233) XVIII.2.16 XVIII.2.21 XVIII.2.24 XVIII.2.26 XVIII.2.29
Unbounded spectral operators, associated with matrices of measurable functions XV.12.2 (2013)
| Unbounded spectral operators, characterization of 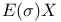 for compact for compact  XVIII.2.3 (2229) XVIII.2.3 (2229)
Unbounded spectral operators, commutivity properties XVIII.2.4 (2229)
Unbounded spectral operators, condition for XVIII.2.28 (2252) XVIII.2.32 XVIII.2.33 XVIII.2.34
Unbounded spectral operators, definition of XVIII.2.1 (2228)
Unbounded spectral operators, definition of, scalar part XVIII.2.27 (2251)
Unbounded spectral operators, examples of XV.12 (2010) (2016-2017) (2020) (2023-2024) XV.12.18
Unbounded spectral operators, polynomial function of XVIII.2.22 (2249)
Unbounded spectral operators, resolution of the identity for XVIII.2.1 (2228) XVIII.2.5 XVIII.2.23
Unbounded spectral operators, restriction of XVIII.2.2 (2228) XVIII.2.19-20
Unbounded spectral operators, special case, case  XV.12.6 (2015) XV.12.6 (2015)
Unbounded spectral operators, special case, condition for XV.12.4 (2014)
Unbounded spectral operators, special case, definition of XV.12.3 (2013)
Unbounded spectral operators, special case, definition of scalar type XV.12.3 (2013)
Unbounded spectral operators, special case, properties of scalar type XV.12.5 (2015)
Unbounded spectral operators, special case, resolution of the identity for XV.12.4 (2014)
Unbounded spectral operators, special case, spectrum and resolvent set of XV.12.7 (2018)
Unbounded spectral operators, spectrum of XVIII.2.25 (2251)
Uniform asymptotic expansion, definition of XIX.3.4 (2309)
Uniform asymptotic representation, definition of XIX.3.4 (2310)
Val ry, Paul 2062 ry, Paul 2062
Valicki , Yu. N. 2131 , Yu. N. 2131
Vasilescu, F.-H. 2090 2092 2095 2113 2117 2120
Vidav, I. 2105
Vizitei, V. N. 2374 2511
Volk, V. Ja. 2129
Volterra, V. vi
von Neumann’s theorem on the algebra generated by a self adjoint operator XVII.3.22 (2215)
Waelbroeck, L. 2090
Walsh, B. J. 2090 2107 2108 2109 2110 2219 2225 2226
Wave operator, perturbation of a self adjoint operator by a symmetric operator XX.4.9 (2460)
Wave operator, stationary approach to the definition of XX.6 (2504)
Wave operators, theorem of invariance of XX.6 (2503)
Weakly compact spectral operators XV.7.4 (1951)
Wecken, F. J. 2226
Weidmann, J. 2132 2374
Wermer, J. 2090 2098 2121 2129 2509 2510
West, T. T. 2128 2131 2132
Weyl, H. vi xiv 2490
Whitley, R. J. 2128 2132
Wiener — Hopf equation in 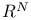 , solution of XV.14.12 (2074) , solution of XV.14.12 (2074)
Wiener — L vy — Hopf theorem XV.14 (2063) vy — Hopf theorem XV.14 (2063)
Wiener, N. vi x 2067 2068 2102 2103
Wiener’s theorem on inverses in 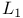 , group algebras XV.14.3 (2067) XV.14.6 , group algebras XV.14.3 (2067) XV.14.6
Wilder, C. E. 2371
Williams, J. P. 2128
Williamson, J. H. 2132
Wolf, F. 2077 2090 2113 2117 2175 2225 2509
Wolf’s operational calculus XV.15.23-31 (2077-2079)
Wright, Frank Lloyd 2062
Yen, T. 2132
Yood, B. 2133
Yoshino, T. 2128 2129
Yosida, K. vi 2107 2511
Zaanen, A. C. 2132 2511
Zabre ko, P. P. 2132 ko, P. P. 2132
Zeno 2056
Zinnes, I.I. 2497 2511
Zygmund, A. 2223
|
|
 |
| Реклама |
 |
|
|
 |
|
О проекте
|
|
О проекте



 , I. V.
, I. V.  g
g , complex conjugate of
, complex conjugate of  , conditions for
, conditions for  for compact
for compact 

 ry, Paul
ry, Paul  , Yu. N.
, Yu. N.  , group algebras
, group algebras