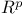|
|
 |
| Авторизация |
|
|
 |
| Поиск по указателям |
|
 |
|
 |
|
|
 |
 |
|
 |
|
| Bartle R.G. — The Elements of Real Analysis |
|
|
 |
| Предметный указатель |
Limit of a function 195—205
Limit of a sequence 100
Limit of a series 376
Limit, deleted 196
Limit, inferior 133
Limit, non-deleted 196
Limit, right-hand 203
Limit, superior 133
Limit, upper 200
Linear function 154
Linear functional 289
Linear space 110
Lipschitz condition 169
Lipschitz, R. 169
Local solvability theorem 255
Locally one-one mapping theorem 251
Logarithm 56 174 221 314
Lower bound 47
Lower integral 298—299
machine 15
Maclaurin, C. 393
Mapping 13
Matrix 156
Maximum value theorem 164
Mean convergence 296
Mean square convergence 297
Mean value theorem for derivatives in 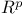 239—240 239—240
Mean value theorem for derivatives in R 209
Mean value theorem for integrals in 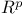 327 327
Mean value theorem for integrals in R 301—304
Member of a set 1
Mertens, F. 385
Metric 67
Metric space 83
Minkowski's inequality 69 273
Minkowski, H. 69
Monkey viii
Monotone convergence theorem for functions 289
Monotone convergence theorem for infinite integrals 360
Monotone convergence theorem for sequences 111
Multiplication, of power series 413
Multiplier, Lagrange 266
Nearest point theorem 89
Neighborhood 73
Nested intervals in a Cartesian space 75
Nested intervals in an Archimedean field 42
Neuman, A.E. 327
Norm of a function 127 296
Norm of a partition 294
Norm of a vector 61—62
Normed linear space 110
Numbers, complex 4
Numbers, natural 3
Numbers, rational 4
Numbers, real 4
O, o 136 (see also 14)
Open mapping theorem 256
Open set 70
Operation, binary 28
Ordered fields 34—45
Ordered pair 9
orders of magnitude 136
Origin in a Cartesian space 59
Orthogonal vectors 66
Pair, ordered 9
Parallelogram identity 64
Parallelpiped 74
Partial derivative 226 261
Partial integral 343
Partial product 404
Partial sum 376
Partition 275 320
Peano curve 319
Perpendicular vectors 66
Piecewise continuous function 311
Point, accumulation 73
Point, boundary 83 324
Point, cluster 73
Point, critical 262
Point, interior 73
Point, saddle 263
Polygonal curve 80
Polynominal, Bernstein 180
Positive class 34
Power series 408 ff.
Power, of a real number 55—56
Preservation of compactness 162
Preservation of connectedness 163
Product of a real number and a vector 59
Product of functions 153
Product of sequences 100
Product, Cauchy 384
Product, dot 61
Product, infinite 403—405
Product, inner 61
Property 3
Quotient of functions 153
Quotient of sequences 100
Raabe's test 391
Raabe, J.L. 391
Radius of convergence 409
Range of a function 13
Ratio Test 390
Rational elements of a field 33
Rational numbers 4 29 34
Rational powers of a real number 55
Real numbers 27—57 especially
Real part of a complex number 94
Rearrangements of series 381
rectangle 74
Remainder in Taylor's theorem, Cauchy's form 212
Remainder in Taylor's theorem, integral form 309
Remainder in Taylor's theorem, Lagrange's form 212
Riemann integral of a function in  316—341 316—341
Riemann integral of a function in R 277 ff.
Riemann — Stieltjes integral 275—300
Riemann, B. 274
Riesz, F. 290
Rolle's theorem 388
Root test 388
Rosenberg, A. 46 64
Rota, G.-C. 446
Saddle point 263
| Scalar product 61
Schoenberg, I.J. 319
Schwarz, H.A. 61
Second mean value theorem 304
Semi-continuity 202
Sequence and sequences 98—145
Sequence and sequences in a Cartesian space 98
Sequence and sequences in a metric space 110
Sequence and sequences of arithmetic means 137
Sequence and sequences of functions 121—131
Sequence and sequences product 100
Sequence and sequences quotient 100
Sequence and sequences, Cauchy 115
Sequence and sequences, convergent 100
Sequence and sequences, difference of 99
Sequence and sequences, divergent 100
Sequence and sequences, double 139
Sequence and sequences, iterated 140
Sequence and sequences, limit of 100
Sequence and sequences, monotone 111
Series 375—421
Series of functions 405 421
Series, absolutely convergent 378
Series, alternating 399
Series, conditionally convergent 378
Series, double 381
Series, geometric 378
Series, harmonic 378
Series, hypergeometric 403
Series, p-series 379
Series, power 408 ff.
Series, rearrangements of 380
Set or sets 1—11
Set or sets, accumulation point of 73
Set or sets, boundary point of 83
Set or sets, bounded 71
Set or sets, Cantor 52
Set or sets, Cartesian product of 9
Set or sets, closed 71
Set or sets, closure of 82
Set or sets, cluster point of 73
Set or sets, compact 84
Set or sets, complement of 7
Set or sets, connected 77
Set or sets, countable 23
Set or sets, denumerable 23
Set or sets, disconnected 77
Set or sets, disjoint 5
Set or sets, empty 5
Set or sets, enumerable 23
Set or sets, equality of 3
Set or sets, finite 23
Set or sets, infinite 23
Set or sets, interior point of 73
Set or sets, intersection of 4
Set or sets, non-intersecting 5
Set or sets, open 70
Set or sets, relative complement of 7
Set or sets, symmetric difference of 10
Set or sets, union of 4
Set or sets, void 5
Shuffled sequence 119
Side condition 266
Space, linear 110
Space, metric 83
Space, normed linear 110
Space, topological 83
Space, vector 110
Space-filling curve 319
Sphere in a Cartesian space 64
Stieltjes, T.J. 274
Stirling's formula 300
Stirling, J. 300
Stone — Weierstrass theorem 185
Stone, M.H. 184
Subsequence 104
Subset 2
Sum of two functions 153
Sum of two sequences 99
Sum of two vectors 59
Sum, partial 376
Sum, Riemann 276
Sum, Riemann — Stieltjes 275
Summability of sequences, Abel 416
Summability of sequences, Cesaro 137
Supremum, definition 47
Supremum, iterated 54
Supremum, Principle 49
Tauber's theorem 417
Tauber, A. 416
Taylor's theorem 211 245 309 338
Taylor, B. 211
Tests for convergence of series 387—405
Tietze extension theorem 187
Tietze, H. 187
topology 83
Transformation 15
Transformation of integrals 331—336
Triangle inequality 38 64
Trichotomy Property 34
Trigonometric functions 222 315 420
Trigonometric polynomial 194
Uniform continuity 166 ff.
Uniform convergence of a sequence of functions 126—131
Uniform convergence of a sequence of sequences 142
Uniform convergence of a series of functions 405—407
Uniform convergence of an infinite integral 352
Union of sets 4 7
Uniqueness theorem for power series 412
Upper bound 47
Upper integral 298
Vector in a Cartesian space 59
Vector space 60 110
Wallis product 299
Wallis, J. 299
Weierstrass approximation theorem 183 ff.
Weierstrass M-test for infinite integrals 352
Weierstrass M-test for series 407
Weierstrass, K. 75
Well-ordering property 23
Zero element of a field 28
Zero vector in a Cartesian space 59
Zero, characteristic 32
Zero, content 317
|
|
 |
| Реклама |
 |
|
|
 |
|
О проекте
|
|
О проекте