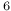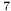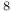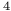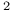|
|
 |
| Авторизация |
|
|
 |
| Поиск по указателям |
|
 |
|
 |
|
|
 |
 |
|
 |
|
| Hausner M., Schwartz J.T. — Lie groups, Lie algebras |
|
|
 |
| Предметный указатель |
A(n) 58—59
Ad  66 66
Adjoint of an operator 6
Adjoint representation 94
Analytic functions of an operator 10—11
Automorphisms, Canonical factorization 181
Automorphisms, Diagonalization 198
Automorphisms, Jordan canonical form 194
Baker — Hausdorff theorem see Campbell — Baker — Hausdorff theorem
Bilinear form 1
Campbell — Baker — Hausdorff theorem 68
Cartan criterion 98—99
Cartan subalgebra 95
Cartan subalgebra isomorphisms 138
Casimir operator 144
Chromosome 120
Classical algebras, models 123—125
Classical algebras, real forms 184—201
Classical groups 81—85
Closed subgroups 77
Commutative algebra 77
Compact groups 220—226
Complexification 87
Component of identity 38
Conjugations, definition 173
Conjugations, equivalence 176
Conjugations, in Algebras of type A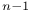 184—187 184—187
Conjugations, in Algebras of type B , C , C , D , D 188— 193 188— 193
Conjugations, in E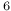 207—213 207—213
Conjugations, in E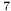 205—207 205—207
Conjugations, in E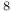 202—205 202—205
Conjugations, in F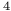 213—214 213—214
Conjugations, in G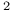 201—202 201—202
Conjugations, positive 176
Connected group 37
Coordinates, definition 20
Coordinates, for functions 21
Coordinates, for tangent vectors 25
Covering group 42
Covering space 14—19
Derivative of a curve 29—30
Diagonalization 4
Differential equations 32
Dynkin diagram 120—121
Eigenspace 5
Eigenvalue, analytic dependence 10
Eigenvalue, definition 5
Eigenvector 5
Exceptional algebras 122
Exceptional algebras, models 126—136
Exceptional algebras, real forms 201—220
Exponential function 60
Factor algebra see Quotient algebra
Factor group see Quotient group
Fundamental group, definition 14
Fundamental group, of a covering space 16
Fundamental group, of a Lie group 42
Germ 44
Gl(n) 58—59
Hermitian form 1
Hermitian operator 7
Homeomorphism 22
Homomorphism, of fundamental groups 14
Homomorphism, of Lie algebras 55
Homomorphism, of Lie algebras, differentiability 64
Homomorphism, of Lie algebras, induced by group homomorphism 56
Homomorphism, of Lie groups 38
Homomorphism, of Lie groups, defined by local homomorphism 39
Homomorphism, of Lie groups, induced by algebra homomorphism 70
Homotopy 13
Ideal, definitions 73 88
Ideal, induced by invariant subgroups 74
Implicit function theorem 12 28—29
Inner automorphism 138
Integrability conditions 56
Invariant measure 19
Invariant subgroup 73
| Invariant subspace 142
Irreducible invariant subspace 143
Isomorphism, of Lie algebras 55
Isomorphism, of Lie groups 38
Isomorphism, of Lie groups, induced by algebra isomorphism 71
Isomorphism, of Lie groups, induced by local isomorphism 41
Isomorphism, of Lie groups, induced by mapping of roots 108
Jacobian 27
Lie algebra 55 86
Lie bracket, definition 50
Lie bracket, induced in a subalgebra 72
Lie group 37
Local homomorphism 38
Local isomorphism, definition 38
Local isomorphism, induced by algebra isomorphism 71
Local isomorphism, inducing global isomorphism 41
Local subgroup 44
Logarithmic coordinate system 64
Manifold 20
Natural bilinear form 94
Neighbors 114
Nilpotent algebra 88 92
Non-singular bilinear form 2
Non-singular subspace 3
O(n) (orthogonal group) 83
Positive definite form 4
Positive definite operator 8
Product manifold 32
Quotient algebra, definition 74
Quotient algebra, determined by quotient group 75
Quotient group, definition 43 75
Quotient group, determining quotient algebra 75
Radical 160
Radical splitting theorem 160—163
Regular map 34
Representations, definition 87
Representations, equivalence 146
Root space 94
Roots, definition 94
Roots, positive 106
Roots, simple set 105
Semi-direct product of groups 165
Semi-direct sum of algebras 159 163
Semi-simple algebra, characterized as a direct sum of simple algebras 99
Semi-simple algebra, characterized by natural bilinear form 94
Semi-simple algebra, characterized by root structure 122
Semi-simple algebra, definition 93
Signature 5
Simple algebra, characterized by root structure 115
Simple algebra, definition 93
Simple algebra, models 123—136
Simple algebra, real forms 215
Simply connected space 13 16
Singular bilinear form 2
Skew-symmetric bilinear form 2
SL(n) (Special linear group) 81
Solvable algebra 88
Sp(n) (Symplectic group) 84
Special linear group see SL(n)
Spectrum 5
Subalgebra 72
Subgroup, definition 44
Subgroup, induced by subalgebra 72
Submanifold 34
Symmetric bilinear form 1
Symplectic group see Sp(n)
Tangent vector, coordinates 25
Tangent vector, definition 23
Tangent vector, on submanifold 35
Topological group 19
Total commutativity 196
Unitary space 6
Vector field 31
Weight space 87 90
Weight vector 87
Weights 87 88 146
Weyl group 140
Weyl reflection 140
|
|
 |
| Реклама |
 |
|
|
 |
|
О проекте
|
|
О проекте




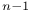
 , C
, C