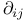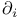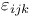|
|
 |
| Авторизация |
|
|
 |
| Поиск по указателям |
|
 |
|
 |
|
|
 |
 |
|
 |
|
| Davis H. F., Snider A. D. — Introduction to Vector Analysis |
|
|
 |
| Предметный указатель |
 102 102
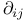 46 46
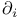 111 111
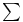 45 45
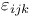 45 45
Acceleration 2 63—73
Acceleration, angular 63
Acceleration, centripetal 63
Acceleration, Coriolis 75
Acceleration, normal to a curve 63
Acceleration, tangential to a curve 63
Acceleration, tensor form 257
Addition see Sum
Affinor 229
Angle between planes 27
Angle between vectors 16—17
Angle, direction 10
Angle, positive sense 29
ARC 59 (see also Curve)
Arc length 59 60
Arc length, in orthogonal curvilinear coordinates 191
Area cosine principle 145
Basis 219 (see also Components Coordinates)
Basis, dual 221
Basis, normal 241
Basis, reciprocal 221
Basis, tangent 241
Binormal 68
Boundary, oriented 139 206
Bounded domain 158
Cartesian coordinates 7 187 256
Center of mass 14
Chain rule 80 287
Christoffel symbols 250
circular motion 63
Circulation 117
Cofactor 215
components 7 8
Components, contravariant 222
Components, covariant 222
Components, mixed 264
Components, physical 242
Components, stress 226
Components, tensor 227 262
Continuity equation 86
Contraction of a tensor 253
coordinates see also Components
Coordinates, affine 259
Coordinates, Cartesian 7 187 256
Coordinates, Cartesian, non-uniqueness 256
Coordinates, change of 13 260—276
Coordinates, orthogonal 189—193
Coordinates, polar 188
Coordinates, right-handed 32 207
Coordinates, spherical 188
Cosine law 24
Cosine principle 145
Cosines, direction 10
Cross product 32 (see also Vector product)
Curl 97—99
Curl, and swirl 97
Curl, as differential of a one-form 202
Curl, formula 97
Curl, formula, in cylindrical coordinates 195
Curl, formula, in general coordinates 255
Curl, formula, in orthogonal coordinates 194
Curl, formula, in spherical coordinates 195
Curl, invariance 255
Curl, physical significance 97
Curl, tensor 255
Curvature 63 67—68
Curve, arc length 59—60 191
Curve, characteristic 86
Curve, closed 54
Curve, curvature 63 67—68
Curve, oriented 55
Curve, parametric equations 53
Curve, regular 55
Curve, smooth 54
Curve, space 52
Curve, tangent to 57 61
Degenerate line segment 1 2
Del notation 102
Delta, Kronecker 46
Density, as a differential form 199
Density, flux 89 149
Density, mass 3
Density, scalar 242 267
Derivative, as an operator 104
Derivative, chain rule 80 287
Derivative, directional 79
Derivative, intrinsic 258
Derivative, of a determinant 253
Derivative, of a vector field 251 257 264
Derivative, of a vector function 50 258 260
Derivative, partial 79
Determinant see also Jacobian
Determinant, and matrices 214
Determinant, cofactor 215
Determinant, differentiation rule 253
Determinant, formula 215
Determinant, symbolic 97
Differential see also Derivative
Differential form 114 198
Differential, of a differential form 201
Differential, total 201
Displacement 11 54
Distance 3
Distance, between a point and a plane 27
Distance, between planes 27—28
Divergence 88—94
Divergence theorem 165 171
Divergence theorem, and differential forms 209
Divergence theorem, and Gauss’s law 165
Divergence theorem, heuristic proof 165
Divergence theorem, proof 171
Divergence, as differential of a two-form 203
Divergence, dependence on coordinates 254
Divergence, in cylindrical coordinates 195
Divergence, in general coordinates 254
Divergence, in orthogonal coordinates 193 243
Divergence, in spherical coordinates 195
Divergence, invariance 256
Division, points of 22
Domain see also Region
Domain, bounded 158
Domain, simply-connected 121
Domain, star-shaped 122
Dot product 22 (see also Scalar product)
Duality 221 240
Dummy 231
Dyadic 280
Einstein convention 253
electric field 86
Equipotential 79
Equivalence of line segments 1 14
Factor, scale 190
Field, as a differential form 198
Field, conservative 123—135
Field, differentiable 123
Field, irrotational 131
Field, matrix 261
Field, scalar 78 (see also Potential)
Field, solenoidal 137
Field, tensor 240 263
Field, vector 85
Field, velocity 86
Flow line 86
Flow, fluid 86 92
Flow, heat 148
| Fluids 86 92
Flux 89 149
force 2 3 12
Force, central 75
Force, centrifugal 63
Force, centripetal 63
Force, lines of 86
FORM 114 198
Frenet formulas 70
Functions see also Field
Functions, and operators 100—104
Functions, harmonic 104
Functions, linear 233
Functions, scalar-valued 78
Functions, vector-valued 50
Gauss’s law 149 153
Geodesic equations 257
Gradient 80
Gradient, as an operator 100—104
Gradient, as differential of a zero-form 202
Gradient, in cylindrical coordinates 195
Gradient, in general coordinates 254 261
Gradient, in orthogonal coordinates 193 254
Gradient, in spherical coordinates 195
Green’s Theorem 180—185
Harmonic 104
Heat 148
Identities, algebraic 42
Identities, analytic (in del notation) 107—108
Identity matrix 212
Independence, linear 42 237
Index, dummy 231
Index, free 231
Inner product 22 (see also Scalar product)
Integrals see Line integrals Surface etc.
Invariance 249 255
Inverse of a matrix 216
Isobar 79
Isotherm 79
Jacobian 241 266
Jacobian, volume integrals 270
Kepler’s second law 75
Kernel letter 265
Kramer’s rule 42
Kronecker delta 46
Laplace’s equation 104
Laplacian 103—105
Laplacian, and differential forms 203
Laplacian, formula 104
Laplacian, formula, dependence on coordinates 256
Laplacian, formula, in cylindrical coordinates 195
Laplacian, formula, in general coordinates 255
Laplacian, formula, in orthogonal coordinates 193 243
Laplacian, formula, in spherical coordinates 195
Laplacian, physical interpretation 104
Line integrals 114
Line integrals, dependence on path 115 124
Line, equations 19—21
Line, flow 86
Line, stream 86
Line, vector parallel 21
Lines of force 86
Magnitude of a vector 2 5 10
Mass 3
Matrix 209 (see also Determinant)
Matrix, column 210
Matrix, identity 212
Matrix, inverse 216
Matrix, row 210
Matrix, trace of 253
Matrix, transpose of 214
Matrix, zero 212
Maximum principle 22
Mechanics see Force Velocity etc.
Metric see Tensor metric
Metric concepts 223 245
Mobius strip 140
Momentum, angular 279
Multiple, scalar 6
Multiplication see Product Scalar Vector
nabla 102
Neighborhood 119
Newton’s Second Law 75 249 257
Nonorientable surface 140
Normal, to a curve 67
Normal, to a plane 27
Normal, to a surface 81 139 142
One-form 198
Operators 100—104
Orientation 29 206
Orientation, of arcs 55
Orientation, of boundaries 139 206
Orientation, of planes 29—30
Orientation, of points 207
Orientation, of regions 207
Orientation, of surfaces 139
Orthogonal curvilinear coordinates 187—195 (see also Coordinates)
Osculating circle 64
Parallelepiped, volume 38
Parallelogram law 4
Parallelogram, area 32
Parallelogram, geometry of 15—16 18
Parametrization 19 53
Permutation symbol 215
Physical components 242
Planes see also Distance
Planes, angle between 27
Planes, equations 25—28
Planes, vectors perpendicular to 27
Point of division 22
Point, boundary 120
Point, interior 120
Polar coordinates 73 188
Position vector 11 19 65
Potential 123
Potential, vector 137
Pressure 79
Pressure and stress 226
Principal normal 67
PRODUCT see also Scalar Product Vector
Product, cross 31—37
Product, dot 22
Product, inner 22
Product, of a vector by a scalar 6 9
Product, of differential forms 199
Product, of matrices 215
Product, wedge 199
Pseudoscalar 268
Pseudovector 245 268
Pythagorean Theorem 8
Quaternions 276—279
Radius of curvature 64
Rank of a tensor 232
Reciprocal basis 221
Reflection 245 281
Region, boundary point 120
Region, bounded 158
Region, connected 120
Region, interior point 120
Region, open 120
Region, oriented 207
Region, simply-connected 121
Resultant 12
Right-hand rule 31 32
Rotation 12
scalar 3
Scalar density 266
Scalar field 78—86
Scalar field, as a differential form 198
Scalar multiple 6
|
|
 |
| Реклама |
 |
|
|
 |
|
О проекте
|
|
О проекте