Авторизация
Поиск по указателям
Vrabie I.I. — Compactness methods for nonlinear evolutions
Обсудите книгу на научном форуме Нашли опечатку?
Название: Compactness methods for nonlinear evolutionsАвтор: Vrabie I.I. Аннотация: This monograph provides a self-contained and comprehensive account of the most significant existence results obtained over the
Язык: Рубрика: Математика /Серия: Сделано в холле Статус предметного указателя: Готов указатель с номерами страниц ed2k: ed2k stats Год издания: 1987Количество страниц: 344Добавлена в каталог: 28.04.2013Операции: Положить на полку |
Скопировать ссылку для форума | Скопировать ID
Предметный указатель
6 Adams 12 298 309 Aizicovici 306 309 Alaoglu 7 Ames 37 309 Arino 6 7 8 301 305 309 Aris 146 309 Arrhenius 146 Arzela 1 10 57 62 63 64 83 86 89 168 Ascoli 1 10 57 62 63 64 83 86 89 168 Attouch 142 143 301 309 Aubin 301 310 Badii 300 305 310 Ball 39 40 298 310 Banach 16 44 Banach space, uniformly convex 18 Baras 64 299 300 310 Barbu 13 17 18 21 26 27 28 29 30 35 38 41 44 58 113 160 174 186 296 298 299 301 310 Bellman 3 134 194 237 238 271 279 282 Benilan 25 34 35 37 41 298 299 311 Bensoussan 308 311 Berens 256 312 Biroli 306 311 Boltzmann 24 Bounded domain with smooth boundary 12 Bourbaki 7 Brezis, D. 256 306 311 Brezis, H. 22 23 41 42 45 53 54 57 186 293 298 299 311 Browder 39 144 180 299 312 Buniakowsky 2 226 Butzer 256 312 Carath 134 135 136 141 142 143 152 161 178 179 180 181 182 186 191 305 Castaing 301 312 Cauchy 2 90 118 181 201 226 234 236 237 249 253 Cellina 301 310 Cioranescu 18 298 312 Clarkson 18 Crandall 30 34 38 298 299 306 311 312 313 Damlamian 142 143 301 309 Deimling 16 298 313 Diaz 300 301 305 310 313 Diestel 8 11 298 313 Dirichlet 23 105 256 Discretization ( 33 Dunford 1 11 321 Eberlein 6 Edwards 6 8 10 11 298 313 Effective domain 26 Egoroff 291 Evans 34 298 312 Fatou 28 77 109 114 Fermat 22 Fitzgibbon 307 313 FOURIER 51 Fujita 263 306 313 Function, 199 Function, A-demiclosed 272 Function, A-dominated 272 Function, compact type, of 57 Function, conjugate 28 Function, convex 25 Function, indicator 27 Function, Lipschitz on bounded subsets 43 Function, lower semicontinuous (l. s. c.) 13 Function, proper 25 Function, upper semicontinuous (u. s. c.) 13 Gautier 6 7 8 301 305 309 Generalized directional derivative 43 Generalized gradient 44 Goursat 308 311 Green 22 23 97 249 251 Gripenberg 306 313 Gronwall 3 Gutman 12 79 82 300 301 314 H 2 11 210 211 239 Hahn 16 44 Hale 307 314 Hardy 3 220 224 226 298 314 Hassan 300 310 Hazan 308 315 Hille 5 7 8 298 299 301 314 Hirano 300 314 Inequality, Bellman 3 Inequality, Buniakowsky — Schwarz 2 Inequality, Cauchy with e 2 Inequality, Gronwall 3 Inequality, H 2 Inequality, Hardy 3 Inequality, Minkowski 2 Inequality, Tartar 2 Intermediate interpolation class 214 Kakutani 7 Kato 18 298 306 313 314 Kobayashi 34 298 314 Kolmogorov 1 11 Kondrashov 1 12 Konishi 57 299 314 Krein 308 315 Kuratowski 116 301 315 Ky Fan 308 Ladyzhenskaja 306 315 Lakshmikantham 14 15 34 35 2 98 315 Lasota 144 301 315 Lax 299 Lebesgue 77 93 179 286 Leeia 14 15 34 35 298 315 Lewis 146 Liggett 30 38 298 312 Lions 308 311 Littlewood 3 298 314 Local existence property 125 Londen 306 313 Luzin 291 Mapping, bounded 121 Mapping, compact 163 Mapping, continuous 118 Mapping, duality 16 Mapping, k-radial truncate of a 153 Mapping, local integrability property (having) 127 Mapping, measurable 116 Mapping, p — Carath 134 Mapping, positively sublinear 130 Mapping, superposition 148 Mapping, weakly continuous 118 Mapping, weakly p — Carath 134 Martin 298 302 305 315 Mazur 5 8 302 Maz’ya 12 315 Milman 18 Minkowski 2 94 281 Minty 26 Mitidieri 300 306 307 315 Moreau 26 Morimoto 263 313 Nagumo 305 Navier 195 256 306 Neumann 24 256 Nohel 306 313 Operator, accretive 19 Operator, compact 49 Operator, m-accretive 21 Operator, resolvent 20 Operator, superposition on 91 Operator, superposition, or natural realization 25 Operator, well defined on 91 Operator, well defined on 91 Operator, Yosida approximation 20 Opial 144 301 315 322 Otani 305 306 316 P 3 298 314 Pavel 15 17 20 298 299 301 305 316 Pazy 39 43 53 55 85 144 180 256 298 299 300 305 306 311 316 Penot 6 7 8 301 305 309 Pettis 1 7 11 301 Phillips 5 7 8 298 299 301 314 Pierre 302 317 Plant 49 317 Popescu 300 317 Prater 146 Precupanu 13 29 58 298 310 Rellich 1 12 Riesz 1 11 Ryll — Nardzewski 116 301 315 Sato 15 16 317 Schauder 8 268 287 308 Schechter 302 317 Schiaffino 305 317 Schwarz 2 226 Selection 116 Semi-inner product, lower 14 Semi-inner product, normalized lower 14 Semi-inner product, normalized upper 14 Semi-inner product, upper 14 Semigroup, compact 50 Semigroup, equicontinuous 52 Semigroup, generated by -A 38 Semigroup, locally weakly equicontinuous 84 Semigroup, nonexpansive mappings, of 37 Semigroup, weakly equicontinuous 52 Set, compact 5 Set, equicontinuous 9 Set, p-equiintegrable 11 Set, precompact 5 Set, relatively compact 5 Set, relatively sequentially compact 5 Set, sequentially compact 5 Set, uniformly integrable 10 Set, weakly equicontinuous 9 Sobolev 112 247 250 Solution, generalized 30 Solution, integral 32 Solution, mild 34 124 265 283 Solution, mild in the sense of Browder 39 Solution, noncontinuable 125 232 2 70 Solution, p-approximate 136 Solution, strong 29 125 150 265 284 Solution, weak in the sense of Ball 39 Stefan 24 101 Stokes 195 256 306 Subdifferential 26 Tartar 2 105 192 Temam 306 318 Tesei 300 305 310 Thiele 146 Topology, strong 4 Topology, weak 4 Topology, weak star 4 Travis 307 318 Tychonoff 8 308 Uhl 8 11 298 313 Valadier 301 312 Veron 43 299 300 310 318 Vescan 308 318 Vitali 93 94 Volterra 71 264 265 272 Von Wahl 260 263 306 318 Vrabie 299 300 301 302 305 306 307 308 313 315 316 318 Vulikh 93 291 320 Webb 307 318 Weil 1 11 Yosida 5 7 18 20 45 298 320 Z 18 320 Zorn 126 127 233 323
Реклама
 |
|
О проекте
|
|
О проекте



 mulian
mulian  odory
odory  )
)  -demiclosed
-demiclosed  lder
lder 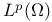
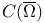
 lya
lya 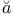 linescu
linescu