|
|
 |
| Авторизация |
|
|
 |
| Поиск по указателям |
|
 |
|
 |
|
|
 |
 |
|
 |
|
| Stein E.M. — Singular Integrals and Differentiability Properties of Functions |
|
|
 |
| Предметный указатель |
 spaces 220—235 spaces 220—235
 , ,  73 117 73 117
Almost-everywhere convergence 9 42 63
Approximation to the identity 62 see
Area integral of Lusin, also S function 89 205 224 265
Bessel potentials 130 133 149 see
Bounded mean oscillation 164
Calderon and Zygmund lemma 17
Cone 89 197
Cone, truncated 201
Conjugate harmonic functions 65 143 212
Convolutions 27
Covering lemma 9
Decomposition of open sets into cubes 16 167
Derivatives in the  sense, pointwise 242 sense, pointwise 242
Derivatives in the harmonic sense 246
Derivatives in the weak sense 121 180 241
Derivatives, ordinary, pointwise 241
Derivatives, symmetric 268
Desymmetrization 257 265
Dilations 37 50 55 110 118
Distance function, 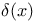 13 170 13 170
Distribution function 4 21 50 121 272
Domain with minimally smooth boundary 189 see
Dyadic decomposition 103
Elliptic differential operators 77 114
Extension operators, 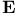 193 193
Extension operators, 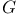 182 191 194 182 191 194
Extension operators, 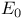 171 171
Extension operators, 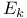 176 176
Fatou's theorem 199
Fatou's theorem, local version 201
Fourier transform 28 56 71
Fractional integration see "Riesz potentials"
Function spaces see "Lipschitz spaces" "Potential "Sobolov
Green's theorem 69 87 208
Hardy inequalities 272
Harmonic functions 60 68 78 196—239 274—276
Hecke's identity 71
Hilbert transform 26 30 38 42 49 50 54
Integral of Marcinkiewicz involving the distance function 14 32 253
Laplacean,  59 60 69 117 59 60 69 117
Lebesgue, set 10 197
Lebesgue, theorem 4
| Lipschitz spaces,  173 176 173 176
Lipschitz spaces, 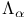 141 163 141 163
Lipschitz spaces,  150 161 193 150 161 193
Littlewood and Paley functions,  88 96 115 162 224 233 88 96 115 162 224 233
Littlewood and Paley functions, 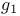 83 96 112 162 83 96 112 162
Littlewood and Paley functions, 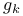 86 86
Littlewood and Paley functions, 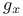 83 112 83 112
Littlewood and Paley functions, g 82 112 155
Marcinkiewicz multiplier theorem 108 114
Maximal functions 4 22—25 42 62 87 92 197 221 236
Minkowski's inequality for integrals 271
Modulus of continuity,  norm 138 140 norm 138 140
Modulus of continuity, regular 175
Multiplier transformations 28 43 75 94 108—114 232
Non-measurable sets 251
Non-tangential boundedness 201
Non-tangential convergence 197 213 236 246
Operators commuting with translations see "Multiplier transformations"
Partial sum operator 99
Partition of unity 170
Point of density 12 251 259
Poisson integral 61 82 87 92 142 197
Poisson kernel 61 142 146 197
Potential spaces,  135 154 161—162 192—193 135 154 161—162 192—193
Principal-value integrals 35
Rademacher functions 104 276—278
rectangle 99
Regular family 10
Regularization 123
Regularized distance 170 182
Restriction to linear sub-varieties 192—193
Riesz potentials 117—121 130 133
Riesz transforms 57 65 75 78—79 112 125 136 143 213 242
Rotations 56 79
Singular integral operators 26—53 66 80 83 232 254
Sobolov spaces, 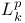 122 135 159—160 180 122 135 159—160 180
Sobolov's theorem 124
Special Lipschitz domain 181 249
Spherical harmonics 68 71 73
Splitting of functions 246 267
Strict definition of a function 192
Symbol 80
vector-valued functions 45
Weak-type estimates 6 20 29—33 42 115 120 272
Young's inequality 271
|
|
 |
| Реклама |
 |
|
|
 |
|
О проекте
|
|
О проекте



 spaces
spaces  ,
, 
 sense, pointwise
sense, pointwise 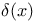
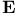
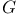
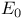
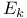


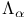


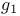
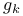
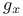
 norm
norm