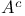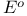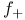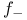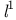|
|
 |
| Авторизация |
|
|
 |
| Поиск по указателям |
|
 |
|
 |
|
|
 |
 |
|
 |
|
| Saxe K. — Beginning functional analysis |
|
|
 |
| Предметный указатель |
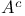 185 185
 16 16
 8 8
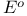 16 16
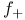 45 45
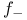 45 45
 96 96
 96 96
 47 47
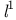 9 9
 132 132
 9 9
 8 8
 61 61
Additive set function 36
Algebra 99
Almost everywhere 48
Analytic Banach space valued function 103
Analytic function 103
Approximation problem 110 123
Arzela, Cesare 12 19
Ascoli — Arzell theorem 17 20
Ascoli, Giulio 12 19
Axiom of Choice 154 159
Baire category theorem 148
Baire, Rene 43
Banach algebra 99
Banach contraction mapping principle 161
Banach space 23
Banach — Steinhaus theorem 153
Banach — Taraki paradox 28
Banach, Stefan 1 28
Band-limited signal 172
Basis 11
Basis problem 110 123
Bell Labs 172
Bernoulli sequence 33
Bernoulli, Daniel 75
Bernoulli, James 33
Bernstein polynomial 136
Bernstein, Sergei 136
Berthollet, Claude Louis 80
Bessel, Friedrich 82
Bessel’s inequality 82 84
Birkhoff, George David 146
Bishop, Errett 144
Bishop’s theorem 144
Bohr, Niels 167
Borel sets 42 58
Borel, Emile 18 43
Boundary of a set 158
Bounded linear operator 95
Bounded set 16
Brouwer, Luitzen 160
Bunyakovskii, Viktor 7
C([a,b]) 9 136
Cantor set 30 34
Cantor, Georg 12
Cauchy sequence in a metric spucc 23
Cauchy — Schwarz inequality 7
Cauchy, Augustin Louis 7 32
Cesaro operator 129
Characteristic function 45
Clopen set 29
Closed set in a metric space 16
Closure of a set 16
Compact linear operator 107
Compact set 16
Compact support, function with 73
Complement of a set 185
Complete metric space 23
Complete orthonormal sequence 77
Complete subset in a metric space 23
Complex conjugate of a complex number 183
Composition operator 129
Connected set 158
Continuous function on a metric space 5
Contraction mapping 161
Convergence in a metric space 5
Convergence in mean 86
Convolution 132
Countable set 186
Countably additive set function 36
Countably subadditive set function 38
Counting measure 58
Cover of sets 16
de Branges, Louis 144
de Morgan’s laws 186
Dense set 16
Diagonal operator 102
Diagonal operator, characterization of compactness of 108
Dirac, Paul 167
Discrete metric 4
Discrete probability space 34
Disjoint sets 185
Dual space 154
Dunham, William 150
Einstein, Albert 27
Enflo, Per 110 112 122
Equicontinuous function 19
Equivalent norms 30
Equivalent sets 186
Essentially bounded function 61
Euclidean norm 5
Eudoxos 32
Fatou, Pierre 52
Fatou’s Lemma 52 64
Fields Medals 26
Finite-dimensional 11
Finite-rank operator 110
Finitely subadditive set function 69
First category set 147
Fourier coefficients 82
Fourier series 82
Fourier transform 167
Fourier, Joseph 4 75 79
Frechet, Maurice 1 11 33
Fredholm operator of the first kind 94
Fredholm operator of the second kind 94
Fredholm, Erik Ivar 12 68 94
Fundamental theorem of algebra 104
Fundamental theorem of calculus 56
Grabiner, Sandy 145
Gram — Schmidt process 77
Haar functions 78
Haar, Alfred 79
Hadamard, Jacques 12
Hahn — Banach theorem (real case) 155
Hahn, Hans 151
Hahn-Banach theorem (complex case) 156
Hamel basis 11
Hausdorff, Felix 13
Heine — Borel theorem 17 18
Heine, Eduard 18
Heisenberg, Werner 167
Helly, Eduard 154
Hermite functions 78
Hermite, Charles 78 114
Hermitian operator 114
Hermitian symmetry property of an inner product 6
Hilbert space 23
Hilbert, David, I 24 33 128
Hilbert’s 23
Hilden, Hugh 120
Hoelder conjugate 60
Hoelder, Otto Ludwig 60
Hoelder’s inequality 60
Ideal 165
Imaginary part of a complex number 183
Index set 185
| Infimum of a set 9
Infinite-dimensional 11
Inner product 6
Inner product space 6
Institut d’Egypte 80
Int(E) 16
Interior of a set 16
Interior point 16
International Congress of Mathematicians 26
Intersection of sets 185
Invariant subspace 112
Invariant subspace problem 112 123
Invertible linear operator 100
Isolated point in a metric space 16
Jordan, Camille 43
Klein, Felix 27
Kolmogorov, Andrei 33
Laguerre functions 78
Laguerre, Edmond 78
Laurent series 105
Law of Large Numbers 33
Lebesgue integral of a function on M 46
Lebesgue measurable subsets 42
Lebesgue measure 34 42
Lebesgue space  59 59
Lebesgue, Henri 9 32 42
Lebesgue’s dominated convergence, theorem 53 64
Lebesgue’s monotone convergence, theorem 50
Left shift 93 100
Left shift is not compact 110
Left shift, spectrum of 111
Legendre polynomials 78
Legendre, Adrien — Marie 78
Levy, Paul P. 1
Limit point in a metric space 16
Linear functional 94 151 153
Linear operator 92
Linear space 3
Liouville, Joseph 103
Liouville’s theorem 103 104
Lipschitz condition 163
Lipschitz, Rudolf Otto Sigismund 163
Lomonosov, Victor 120
Lomonosov’s theorem 120
Machado, Silvio 145
Maximal ideal 165
Measurable function on R 44
Measurable sets 34
Measure 34 36
Measure space 36
Metric 4
Metric space 4
Minkowski, Hermann 26
Minkowski’s inequality 64
Monge, Gaspard 80
Morgenstem, Oskar 175
Multiplication operator 110
Norm 5
Norm of a bounded operator 96
Normalized vector in an inner product, space 76
Normed algebra 99
Normed linear space 5
Nowhere dense set 147
Observable 101 167
Open ball 14
Open ball in a metric space 16
Open cover of sets 16
Open mapping theorem 151
Open set 16
Orthogonal complement 115
Orthogonal vectors in an inner product, space 76
Orthonormal basis 77
Orthonormal sequence 76
Oscillation of a function 149
Outer measure 37 38
Pairwise disjoint collection of sets 186
Parallelogram equality 7 14
Parseval, Marc — Antoine 83
Parseval’s theorem 83
Partially ordered set 154
Peano, Giuseppe 3
Picard, Emile 160
Picard’s iterative method 161
Plancherel identity 168
Plancherel, Michel 168
Poincare, Henri 160
probability 33 136
Probability measure 58
Problems 26
Projection in an inner product space 90
Projection operator 128
Proper ideal 165
quantum mechanics 101 167
Quasinilpotent operator 112 121 132
R-ball centered at x 16
Ransford, Thomas 145
Read, Charles 112
Real part of a complex number 183
Riemann integral 54
Riemann, Georg Friedrich Bernhard 32
Riesz theory for compact operators 108
Riesz — Fischer theorem 84
Riesz — Frechet theorem 115
Riesz, Frigyes 22 32 59 67 68 107 128
Riesz, Marcel 22
Right shift 93 100
Right shift, invariant subspace of 113
Right shift, spectrum of 111
Ring 165
Ring of sets 35
Ring with unit 165
Rosetta stone 80
Runge, Carle 145
Runge’s theorem 145
Schauder basis 11 123
Schauder fixed point theorem 121
Schrodinger, Erwin 167
Schwarz, Hermann 7
Scottish Cafe 28
Second category set 147
Separable metric space 22
Sequentially compact 16
Shift operator 93
Simple function 45
Spectral radius 105
Spectral radius formula 105 112
Spectral theorem for compact Hermitian operators 127
Spectral theory 101
Spectrum 101
State function 167
Steinhaus, Hugo 28 61
Step function 67
Stone — Weierstrass theorem 140
Stone, Marshall 128 145
Strong Law of Large Numbers 35
Subcover of sets 16
Submultiplicative norm 99
Supremum of a set 9
Symmetric difference of sets 38
Symmetric, A-symmetric subset 144
Time-limited signal 172
Totally bounded set 18
Totally ordered set 155
Translation invariance of Lebesgue, measure 158
Trigonometric polynomials 86
Trigonometric system 76 86 88
Uncertainty principle 169 172
Uncountable set 186
Uniform algebra 145
Uniform boundedness principle 153
|
|
 |
| Реклама |
 |
|
|
 |
|
О проекте
|
|
О проекте