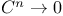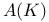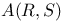Авторизация
Поиск по указателям
Schneider H. (ed.) — Recent advances in matrix theory
Обсудите книгу на научном форуме Нашли опечатку?
Название: Recent advances in matrix theoryАвтор: Schneider H. (ed.) Аннотация: The theory of matrices goes back to Sylvester and Cayley, particularly to Cayley's famous memoir of 1858. The subject Is thus classical and central In the mathematical tradition. This century, mathematical progress has been richer and more rapid than ever before.
Язык: Рубрика: Математика /Серия: Сделано в холле Статус предметного указателя: Готов указатель с номерами страниц ed2k: ed2k stats Год издания: 1964Количество страниц: 142Добавлена в каталог: 17.03.2011Операции: Положить на полку |
Скопировать ссылку для форума | Скопировать ID
Предметный указатель
133 Absolute 49 Afriat 137 Albert 137 Alpha-width 107 Aronszajne 136 arrow 133 Bartels 132 Bauer, F.L. 130 Bellman 132 136 137 Birkhoff 86 Bordering 136 Brauer, A. 127 130 132 138 Brenner 136 137 138 Browne 130 Bueckner's theorem 45 Carlson 133 Characteristic polynomials of product of matrices 138 Characteristic roots, simplicity of 83 Class 122 Class 110 Class 104 Column sum vector 104 Comparability 98 Comparability, constant 94 Comparable 94 Completely non negative 137 Compound matrix 136 Condition number 130 Convex body 46 Convex body, cone 87 Convexity 134 Cournat 136 Cross-ratio 87 91 David 122 Davis, C. 126 135 Decomposable 62 Diagonals 137 Dominant 137 Dominant root 127 Double interchange 113 Downing 132 Drazin 134 Dungey 134 Elementary divisors 84 Elements, rearrangements of 138 Elements, replacing of 136 Equilibrated 46 Exclusion region 39 Exclusion region theorem 39 53 Exponent, of even 4 Factor analysis 137 Fan 129 130 135 136 137 Farahat 138 Feingold 55 137 Fiedler 55 125 126 136 137 Fisher, M.E. 132 Flanders 134 Ford 122 Forsythe 125 130 132 137 Foster 131 Frebenius 134 Friedman 136 Fulkerson 115 121 122 123 Fuller, A.F. 132 Functional analysis 81 Functionals 94 Functionals, linear positive 94 Functionals, r-multilinear 61 Gale 123 Gantmacher 123 137 Gauss Seidel iteration method 132 Gauss transformation 137 Generalized stochastic matrix 10 Gershgorin 39 57 128 Gershgorin circle sets 129 Gershgorin theorem 53 Gerstenhaber 134 135 givens 136 Goddard 138 Goldstine 130 Golub 130 Gross 121 Gruenberg 134 Haber 108 123 Hadamard inequality 137 Hadamard tournament matrix 122 Hadamard — Schur product 136 Haynsworth 137 Hermitian matrices, products of 133 hilbert 87 Hilbert matrix 137 Hoffman, A.J. 115 122 126 130 136 137 Hopf, E. 92 Horn 130 132 136 137 Householder 128 133 136 137 Incidence matrix 103 132 Inclusion region 39 Inclusion region, theorem 39 42 47 Indecomposable 127 Initial part of row 115 Interchange 106 Interchange, theorem 106 Invariant 103 106 Inverse-characteristic value problem 132 Irreducible class 121 Irreducible class, components 120 Irreducible class, matrix 119 Johnson 123 Kac 134 Kantorovich 57 Kato 127 Kingman 135 Koenig 107 123 Kotelyanskii 127 128 136 137 Krein 137 Kronecker, product of 136 Krylov iterates 43 Landau 110 123 Lax, A. 127 135 Least square problem 130 Lebesque — Stieltjes integral 92 Lederman 138 Lehmann 41 Lewis, D.C. 136 Liapunov, theorem of 133 Lidskii 132 135 Lie algebras 134 Liederman 136 Line of matrix 106 Localization theorem 39 Loewy 132 lotkin 137 Lowner 135 Majorization 104 127 Marcus 121 123 132 135 136 137 Matrices 127 Matrices with identical characteristic equations 138 Matrices, commuting 134 Matrices, moment 44 Matrix function 64 Matrix, block diagonal 136 Matrix, commuting 136 Matrix, double stodhastic 66 Maximal comparable part 98 Maximal matrix 104 McAndrew 115 122 McCoy 134 Miller 135 Minc 123 135 Minimax theorems 41 Minkowski inequality 137 Moez, Amir 130 132 Monotone column sum vector 104 Monotone row sum vector 104 Monotone tournament matrix 110 Monotonic 49 Monotonicity 134 Moon 123 Moser 121 124 Motzkin 134 137 Nirschl 39 41 55 137 Non negative matrices 127 Norm 46 Normalized class 107 Normalized class, matrix 109 Nudel’man 132 Number theoretical problem 131 Oldenburger, R., lemma of 81 Operator, completely symmetric 63 Operator, permutation 63 Operator, skew symmetric 63 Operator, symmetry 63 Oppenheim 137 Ore 123 Osborne, E.E. 138 Ostrowski 55 126 128 130 132 133 136 137 138 Pall 137 Parker, W.V. 130 138 Permanent 64 Perron's theorems 81 Perturbation 125 Polar decomposition 135 Positive matrices 81 Positive matrices, operators 92 Power-positive 4 Power-positive of odd exponent 4 Projective metric 86 Property L 127 134 Property P 134 Ptak 55 Rayleigh quotient theorem 39 42 Reduced 4 Reducible matrix 119 Relaxation method 137 Rellich 126 Robertson 138 Rothe, W.E. 138 Round robin matrix 109 Round robin tournament 109 Row sum vector 104 Ryser 123 Schneider, H. 39 41 133 134 Schroeder 57 schur 83 Schwarz, B. 138 Separation locus 43 Separation locus, polynomial 43 Separation locus, theorems 42 Shoda 132 Singular values 130 Size of matrix 103 Skew symmetric 63 Stable 132 Stable, stable D 133 Stable, stable H 133 Stable, stable S 133 Stein, P. 134 Steinberg, R. 136 Stochastic 128 Strang 136 Straus 130 132 Subdeterminants 136 Svarcman 132 Swanson 49 Symmetric product 63 Symmetrization 129 Symmetry class 63 Taussky 127 130 132 133 134 136 138 Tensor product 62 Tensors 4 Tensors, contravariant 62 Term rank 106 Terminal part of row 115 Thompson, R.C. 136 132 Todd, J. 130 133 Tournament matrix 109 Transition point 118 Triangle inequality 98 Uniformly positive 99 Unreduced 4 Van der Waerden, conjecture of 66 Varga 55 128 133 136 137 Vector lattices 87 Vector, oscillation of a 97 Vector-lattice 97 von Neumann 130 Weinberger 135 Weyl, H. 130 135 Wiegmann 135 Wielandt 49 126 132 133 135 136 Williamson 137 Young D., successive overrelaxation 132 Zassenhaus 133 Zero-one matrix 103
Реклама
 |
|
О проекте
|
|
О проекте