|
|
 |
| Авторизация |
|
|
 |
| Поиск по указателям |
|
 |
|
 |
|
|
 |
 |
|
 |
|
| Streater R.F., Wightman A.S. — PCT, spin and statistics and all that |
|
|
 |
| Предметный указатель |
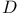 -state 26 -state 26
 -field 27 -field 27
Abnormal commutation relations 154 158
Adjoint 88
Algebra, polynomial 137 138
Algebra, von Neumann 142 169
Analytic 48
Analytic completion 77
Analytic continuation 49 66
Analytic continuation of  16 64 16 64
Analytic function 48
Annihilation operator 26 139 165
Anti-commutation relation 100
Anti-commute 100
Anti-linear 8
Anti-unitary 7 8 17
Asymptotic completeness 26 102
Asymptotic completeness, condition 169
Asymptotic field 26
Bare mass 2
Bare vacuum 2
Baryon number 5
Baryon number, superselection rule 5
Borchers class 168
Bose — Einstein statistics 146
Bounded operator 5 89 142
Bounded set 36
Canonical commutation relations 100
Cauchy sequence 85 121 122
Cauchy — Riemann equations 56
Causality 100
Charge conjugation 16 113
Closed graph theorem 90
Cluster decomposition property 111 117 148 153
Coherent subspace 6
Collision states 24 98 126
Commutant 6 139
Commutation relations 100
Commutation relations, abnormal 154 158
Commutation relations, normal 145 154 156
Commutative superselection rule 6 7
Commutativity 101
Commutativity, local 100 109 118 134
Compact set 35
Complete commuting set 6
Complete space 36 85
Complex Lorentz group 13
Complex Poincar 6
Complex Poincar, group 14
Component of a group 10
Composite particle 24
Conservation law 130
Constant field 100
Continuation, analytic 49
Continuous linear functional 32
Convergence in 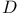 33 33
Convergence, in  35 35
Convergence, strong (in norm) 107
Convolution 41
Creation operator 26 139
Cyclic 101 141
Dense set 85
Dirac 8
Dirac,  -function 31 -function 31
Dirac, equation 18—20
Dirac, field 99
Direct product 16
Direct sum 86
distribution 31 32
Domains of definition 87 88 98
Dotted index 15
Edge of the wedge 74—84 116
Elementary system 22—24
Energy 29 97
Environment, real 49
Equivalence, class 168
Equivalence, S- 170
Essentially self-adjoint 89
Even-odd rule 153 154
expectation value 106 114
Extended tube 64 114 143
Extension 88 89 98 99
Fast decrease 40 47 54
Fermi — Dirac statistics 146
Field 100
Field theory 100 101
Form-invariant 16
Four-vector 17 99
Fourier transform 43
Free field 27 102 116
Generalized free field 105
Generalized Haag’s theorem 166
Graph 88
Haag — Ruelle theory 2 142 168
Haag’s theorem 163 165
Heisenberg picture 4
Hermitian operator 4 89
Hilbert space 84—93
Holomorphic 48
Homomorphism 12
Identity representation 22
Infrared problem 24
Inhomogenous groups, Lorentz 14
| Interaction picture 4 161 166
Invariance principle 7
Irreducible representation 15 23
Irreducible representation, set of operators 101 140 141
Jost point 70—72 74 143 144
Kernel 42
Klein transformation 147 155 157
Laplace transformation 52
Linear functional 32
Linear operator 4 8
Linear program 116
Local commutativity 100 109 118 134
Local, relative 168
Lorentz group 9
Mass 97
Mass gap 111
Matrix representation 15
Maximal Abelian 6
Microscopic causality 100 109 118 134
Midden 2
Mixed state 4
Mixed state, (ftnt), Momentum 25 29 97
Monomial 156
Multiplicative symmetry 129
Multiplicity 25 29 97
Neighborhood 64 66
Nonlinear program 117
Nonseparable 86 87
Norm 33
Normal commutation relations 145 154 156
Nuclear theorem 42 43 83 108 138
Observable 4—6
One-particle state 22
One-sided Laplace transform 53
Operator 88
Orthochorous 10 11
Orthochronous 10 11
Orthogonal complement 88
Out states 26
Painlev 6
Painlev, stheorem 74
Parity of numbers 156
Pauli — Lueders 142 143
Permuted extended tube 73
Physically realizable 4
Picture, Heisenberg 4
Picture, interaction 4
Picture, Schroedinger 6
Restricted Lorentz group 11 14 168
Ruelle — Haag theory 2 142
S-equivalence 170
S-equivalence, matrix 26
Scalar field 24 117 127
Scalar product 23 85 103 119
Schlieder — Reeh 138
Schrodinger picture 4
Schwartz nuclear theorem 42 83 138
Schwarz inequality 120 121
Schwarz reflection principle 76
Second-rank spinor 17
Self-adjoint 89 98
Separable 36 85 87
Separating vector 139
Single-valued 49
Smeared fields 97 164
Smooth 31
SNAG-theorem 2
Space inversion 8 10 16
Space-time inversion 10
Special linear group 11
Spectral condition 97 108 117
Spin 22 23
Spin, and statistics 146
Spinor 11 15
Spinor, Dirac 18
Substitution law 16 17 20
Superposition principle 45
Superselection rule 5
Support 32
Symmetry 7 8 16 17 126 129
Tempered 33
Tensor product 40 87
Test function 31
Time inversion 10 16
Time slice 105 142
Totally space-like 116
Transformation law 17—19 99
Transition amplitude 4
Transitive 172
Translation 14
Tube 48 54 59
Two-sided Laplace transform 53
Unbounded operator 88 90 97 98
Unit ray 4
Unit vector 4
Unitary operator 7
Univalence 5 153
VACUUM 21 97
Vector 4
Vector correspondence 8
Weak local commutativity (WLC) 142 143 145 146
Weakly local fields 105
Wick polynomial 104 105 169
|
|
 |
| Реклама |
 |
|
|
 |
|
О проекте
|
|
О проекте



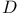 -state
-state  -field
-field 
 -function
-function