Авторизация
Поиск по указателям
Blanchard P., Bruening E. — Mathematical Methods in Physics: Distributions, Hilbert Space Operators, and Variational Method
Обсудите книгу на научном форуме Нашли опечатку?
Название: Mathematical Methods in Physics: Distributions, Hilbert Space Operators, and Variational MethodАвторы: Blanchard P., Bruening E. Аннотация: Physics has long been regarded as a wellspring of mathematical problems. "Mathematical Methods in Physics" is a self-contained presentation, driven by historic motivations, excellent examples, detailed proofs, and a focus on those parts of mathematics that are needed in more ambitious courses on quantum mechanics and classical and quantum field theory. A comprehensive bibliography and index round out the work. Key Topics:
Язык: Рубрика: Физика /Статус предметного указателя: Готов указатель с номерами страниц ed2k: ed2k stats Год издания: 2003Количество страниц: 471Добавлена в каталог: 09.04.2010Операции: Положить на полку |
Скопировать ссылку для форума | Скопировать ID
Предметный указатель
Adjoint 47 Adjoint operator 251 Almost periodic functions 224 Angle between two vectors 189 Baire space 448 Banach, reflexive, examples of 381 Bilinear form 455 Bilinear form, continuous 455 Bilinear form, separately continuous 455 Boundary value 154 Bounded linear function 17 Bounded pointwise 241 449 Bounded uniformly or norm 241 Breit — Wigner formula 37 Calculus of variations 373 Caratheodory functions 420 Carrier 163 Cauchy estimates 120 Cauchy sequence in 35 Cauchy — Riemann equations 126 Cauchy's integral formula I 118 Cauchy's integral formula II 119 Cauchy's principal value 33 Chain rule 55 Change of variables 54 Class 390 Coercive 383 Commutation relations of Heisenberg 280 Compact, locally 237 Compact, relatively 381 Compact, sequentially 380 complete 190 214 Complete, sequentially 16 Completeness relation 214 Completion of a normed space 443 Completion of an inner product space 443 Constraint 403 Continuous at 17 Continuous on X 17 Convergence of sequences of distributions 35 Convergence of series of distributions 40 Convex 396 Convex minimization 382 Convolution - product of functions 83 Convolution in 85 Convolution of distributions 92 Convolution, equation 99 Core of a linear operator 257 Core of a quadratic form 266 Delta function, Dirac's 3 Delta sequences 36 Density matrix or statistical operator 308 Derivative 388 Derivative of a distribution 48 Derivative, weak or distributional 64 Dido 374 Dido's problem 409 Differentiable, twice 390 Direct method of the calculus of variations 376 Direct orthogonal sum 201 Direct sum of Hilbert spaces 228 Dirichlet boundary condition 376 Dirichlet boundary conditions 418 Dirichlet form 267 Dirichlet integral 375 Dirichlet problem 375 417 Dirichlet, Laplacian 419 Distribution with support in 57 Distribution, Dirac's delta 33 Distribution, local order 30 Distribution, order 30 Distribution, periodic 55 Distribution, regular 5 Distribution, tempered 42 Distributions 29 Distributions of compact support 29 43 Distributions, regular 31 32 Distributions, tempered 29 Divergence type 423 Domain of a linear operator 248 Dual, algebraic 9 Dual, topological 18 27 Eigenvalue problem, nonlinear 423 Elementary solution, advanced resp. retarded 112 Equi-continuous 449 Euclidean spaces 193 Euler 373 Euler — Lagrange equation 376 401 Euler — Lagrange, necessary condition of 394 Existence of a minimum 380 Extension of a linear operator 249 Extension of a quadratic form 266 Fock space, Boson 234 Fock space, fermion 234 Form domain 266 Form norm 266 Form sum 273 Fourier expansion 214 Fourier hyperfunction 164 Fourier transform 129 Fourier transformation 129 Fourier transformation on 142 Fourier transformation on 137 Fourier transformation on 136 Fourier transformation, inverse 133 Frechet differentiable 388 Fredholm alternative 330 Friedrich's extension 272 Fubini's theorem for distributions 79 Function, Heaviside 49 Function, strongly decreasing 20 Functional 375 Functional calculus of self-adjoint operators 356 Functional, analytic 163 Fundamental theorem of algebra 121 Fundamental theorem of calculus 392 Gateaux derivative 398 Gateaux differential 397 Generalized functions 5 Generalized functions, Gelfand type 162 Gram determinant 204 205 Gram — Schmidt orthonormalization 213 Green's function 106 Hadamard's principal value 34 Hamilton operator, free 261 Heat equation 110 147 Heisenberg's uncertainty relation 311 Helmholtz differential operator 146 Hermite functions 221 Hermite polynomials 221 Hilbert cube 304 Hilbert space 191 Hilbert space basis 212 Hilbert space, separable 211 212 Hilbert sum or direct sum of Hilbert spaces 228 Hilbert transform 96 Hilbert — Schmidt operator 308 Hlctvs, metrizable 445 Holomorphic 117 Hyperfunctions 164 Hypo-elliptic 107 Hypo-ellipticity of 116 Inequality, Bessel's 188 Inequality, Cauchy — Schwarz — Bunjakowski 189 Inequality, Schwarz' 188 Inequality, triangle 189 Infinitesimal generator 299 Inner product space 187 Integral equation 179 Integral of functions 391 Integral operators of Hilbert — Schmidt 278 Integral with respect to a spectral family 344 Isometry 297 Isoperimetric problem 374 Kato perturbation 314 Kato perturbation of free Hamiltonian 316 Knotensatz 220 Lagrange multiplier 403 Lagrange multipliers, existence of 407 Laguerre functions 222 Laguerre polynomials 221 Laplace operator 145 Laplace operator, fundamental solution 108 Laplace transform of distributions 158 Laplace transform of functions 158 Laurent expansion 123 Lebesgue space 195 Legendre polynomials 222 Leibniz 373 Leibniz formula 53 Lemma of Riemann — Lebesgue 129 Lemma of Riesz 329 Lemma of Weyl 105 Lemma of Wielandt 281 Length 187 Level surface 404 Linear hull or span 200 Linear operator from 248 Linear operator of multiplication 259 Linear operator, bounded 250 275 Linear operator, closable 255 Linear operator, closed 253 Linear operator, closure of 255 Linear operator, core of 257 Linear operator, essentially self-adjoint 256 258 Linear operator, positive 288 Linear operator, product or composition 250 Linear operator, self-adjoint 256 258 Linear operator, symmetric 256 Linear operator, unbounded 250 276 Local extrema, necessary and sufficient conditions for 394 Locally integrable 15 Lower semi-continuous 379 Matrix spaces 193 Maximal 214 Maximal self-adjoint part of A 351 Maxwell's equations in vacuum 112 Metric 18 Metric space 441 Metric space, completion 442 Metrizable, HLCTVS 18 Minimax principle 415 Minimizer, existence 380 Minimizer, uniqueness 380 Minimizing point 396 Minimizing sequence 376 Minimum (maximum), global 394 Minimum (maximum), local 393 Modulus of an operator 289 Momentum operator 260 Monotone 396 Muliplicator space 53 Multiplication operator, bounded 277 Multiplication operator, unbounded 278 Neumann series 287 Newton 373 Norm 8 Norm of a bounded linear operator 275 Norm topology 190 Norm, Hilbertian 192 Norm, induced by an inner product 189 o 388 ONB, characterization of 213 Open ball 441 Operator of finite rank 302 Operator, compact 301 Operator, completely continuous 301 Operator, inverse 248 Operator, trace 305 Operator, trace class 304 Orthogonal 187 Orthogonal complement 199 Orthonormal 187 Orthonormal basis 212 Orthonormal polynomials 219 Orthonormal system 187 p-ball, open 10 Parallelogram law 192 Parseval relation 214 Partial differential operator, linear constant coefficients 50 Partial differential operator, linear elliptic 420 Pauli matrices 234 Poisson equation 109 Polar decomposition 289 Polarization identity 192 Pole of finite order 124 Pre-Hilbert space 187 Product of distributions and functions 52 Product, inner or scalar 186 Product, rule 52 Projection theorem for closed subspaces 201 Projection theorem for convex sets 413 Projector or projection operator 293 Pseudo function 35 Pythagoras, Theorem of 188 Quadratic form 265 Quadratic form, closable 266 Quadratic form, closed 266 Quadratic form, continuous 266 Quadratic form, densely defined 265 Quadratic form, first representation theorem 269 Quadratic form, lower bound 266 Quadratic form, minimization 383 Quadratic form, positive 266 Quadratic form, second representation theorem 271 Quadratic form, semi-bounded, from below 265 Quadratic form, symmetric 265 Quadratic functional 383 Radon measure 67 Regular (critical) point 394 Regular points of an operator A 318 Regularization of distributions 87 Regularizing sequence 88 Renormalization 58 Residue 124 Resolution of the identity 295 341 Resolvent 318 Resolvent, identity 319 Resolvent, set 318 Restriction of a linear operator 249 Riemann integral 311 Rodrigues' formula 221 Schroedinger operator free 148 Schwartz distributions 159 Self-adjoint, geometric characterization 339 Semi-metric 18 Semi-norm 8 Semi-norm, comparable 11 Semi-norm, smaller than 10 Semi-norms, system of 11 Semi-norms, system of, filtering 11 Semi-norms, systems of, equivalent 12 Sequence space 194 Sequence, Cauchy 15 190 Sequence, converges 15 190 Set, open 8 Singularity, essential 124 Singularity, isolated 124 Singularity, removable 124 Sobolev constant 417
Реклама
 |
|
О проекте
|
|
О проекте



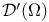

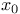
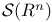

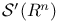


 into
into 
