|
|
 |
| Авторизация |
|
|
 |
| Поиск по указателям |
|
 |
|
 |
|
|
 |
 |
|
 |
|
| Friedman H.L. — Ionic Solution Theory Based on Cluster Expansion Methods |
|
|
 |
| Предметный указатель |
Activity 82
Analytical continuation 137
Association of ions 220
At least doubly connected, ALDC 48
At least singly connected, ALSO 46
Bicycle graphs 185
Binary electrolyte 196
Binomial theorem 24
BOND 6 43 127
Cavendish experiment 133
Chain 45 50 127
Class of graphs 155
Classification of sets 22
Cluster expansion of configuration integral 8 41
Cluster expansion of correlation function 96 169
Cluster expansion of excess free energy 91 165
Cluster expansion of potential of average force 96 169
Cluster functions of ionic solution theory 163
Cluster functions, expansion of 147
Cluster integrals in correlation function expansion 96 169
Cluster integrals in potential of average force expansion 96 170
Cluster integrals, convergence of modified 165
Cluster integrals, divergence of 13 115 123 136
Cluster integrals, irreducible 47 51 60 89
Cluster integrals, modified irreducible 148 159 173
Cluster integrals, reducible 47 51 60 89
Cluster integrals, singularity of modified 173
Cluster, irreducible 48
Cluster, reducible 46
Common ion 230
Component potentials 36
Component potentials, cluster expansion of 104
Component potentials, examples of higher 38
Composition set 19
Concentration set 19
Connectivity 45 153
Convergence of cluster expansion, series 86 91 137 173
Convolution approximation 166
Convolution integral 127
Convolution theorem 127 182
Coordinate set 20
Correlation function 78
Correlation function for ionic solutions 167
Correlation function, relation to thermodynamic excess functions 98
Correlation function, spatial 79 85 93
Correlation function, uses 98 172
Coulomb potential 115 118
Coulomb potential, definition of 136 143
Covering of a set 28
Cumulant 31 47 48 89
CYCLE 124 127 149
Debye Hiickel limiting law 14 17 151 174 208 209
Debye Hiickel theory 139
Debye potential 17 137 141 142 159
Debye potential, correction to 170
Debye — Huckel limiting laws expansions, for mixed electrolyte solutions 200
Debye — Huckel limiting laws for 209
Debye — Huckel limiting laws for electrolyte solutions 196
Debye — Huckel limiting laws, partial 191 194
Debye — Huckel limiting laws, relation of Gex to 203
Debye — Huckel limiting laws, total 191 202
Dirac delta function 129
Dirac delta function, method to determine q(r) 143
Direct potential 36
Direct potential for ionic solutions 3 116 117
Direct potential, sectionally uniform  214 214
Direct potential, solvent contributions to  119 119
Direct potential, square-well form for  215 215
Directly connected 45
Disjoint set 20
Distinguishable graphs 7 46
Donnan equilibrium 105
EDGE 46
Elements of a protograph 152
Elements of a set 18 263 264
Empty set 19
Excess Gibbs free energy, calculation from data for electrolyte mixtures 199
Excess Gibbs free energy, relation to 203
Excess thermodynamic functions 67 ff. 191
Excess thermodynamic functions, cluster expansions for 91 98 163
Fourier transform of 132
Fourier transforms 16 126
Fourier transforms, convolution theorem 127
Fourier transforms, inversion formula 128
Fourier transforms, table 131
Fuchs’s derivation of the cluster, theory of multicomponent systems 59
Fugacity 68
Functional derivative 94
g-bond chains 136 148
g-bond chains, sum over 136 137
Generating function 53
Generating function for partitions 32
Generating function for tree coefficients 56
Generic probability density 76
grand canonical ensemble 72
| Grand partition function 71
Grand partition function, generalization of 75
graphs 6 43
Graphs, expansion of 147 177
Guggenheim’s deduction of the ideal solution equation from the cluster theory 101
Haga’s calculation of higher terms 222
Harned coefficients 201 236
Harned’s rule 201 226 230 235
Hetero ion 230
Higher-order limiting law 210 235 243
Hill’s theory of the Donnan equilibrium 105
Husimi tree 48 90
Hypothetical states (see Standard states)
Indirectly connected 45
Inversion of Fourier transforms 128
k bonds 147 163
Kappagraph 163 177
Kirkwood - Scatchard function B(L,O) 218
Kirkwood superposition approximation 103
Labeled vertices 7 43 151
Lebowitz and Percus method to calculate the cluster expansion of the correlation function 94
Levine and Wrigley’s calculated values of  122 122
Limiting laws 208 235 237 246
Mayer’s ionic solution theory 147 ff.
Mayer’s summation procedure (rearrangement) 14 166 145 148 173
McMillan — Mayer theory 72 84
Mean solute quantity 194
Mixed electrolyte solutions 225
Mixed electrolyte solutions, symmetric 234
Mixed electrolyte solutions, unsymmetric 85 244
Mixing fraction 197
Mixtures, symmetric and unsymmetric 85 100 192 195
Molal concentration scale 193 194
Molal ionic strength 196
Moment of a distribution 31
Moment of the concentration of charges 179
Multinomial theorem 25
nodes 148
Notation 17
Notation, brackets 22
Notation, dimensionless forms of functions for extensive thermodynamic variables 67
Notation, dimensionless forms of functions for scales on figures and entries in tables 237
Notation, dimensionless forms of functions for sets 18
Notation, dimensionless forms of functions sums, products, and integrals 22 31
Notation, dimensionless forms of functions thermodynamics of ionic solutions 193
Ordered products 158 178
Osmotic equilibrium 81 105 118 191 203 225
Partition 28 47 51 89
Partition set 22 29
Partition, ordered 57
Phase transition 86 98
Poirier’s calculations using the cluster theory 216 220
Poisson — Boltzmann equation 140 143 144 145
Poisson’s equation 134 139 140 143
Potential of average force 34 103
Potential of average force, cluster expansion of 96 104 169
Primitive model 1 214 239 246
Protograph 151
Prototype graph 153
Radial functions 130
Salpeter-Mattis method to calculate q(r) 143
Scatchard, Vonnegut, and Beaumont freezing point data for LaCUCaq) 213
Scatchard-Prentiss equation for electrolytic mixtures 243
Sequences of series 25
Sequences of series, compared to Taylor’s series 86
Sets 18
Sets as summation indices 22 23 31
Sets, classification of 22
Sets, notation for various 19
Sets, operations with 20
Shedlovsky and Maclnnes emf data for 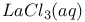 213 213
Skeleton 6 43
Solutions (see Mixtures)
Solvent, Solute 36 81 83 85 192
Specific probability density 76
Standard states 70
Standard states, hypothetical c=1 gas state 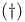 192 204 192 204
Standard states, hypothetical m=1 solution state 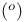 192 204 192 204
Standard states, ideal gas state 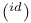 68 68
Standard states, solution reference state (*) 194
Surface terms 46 53 60 91 116 164
Thermodynamic functions 67 191
Thiele seminvariant 31
topology 6 45
TREE 22 49
Tree coefficient 53
Tree coefficient, generating function for 56
Tree coefficient, recursion formula for 53
Tree Set t]{n} 51
van’t Hoff model 82
Vertex 6
Vertex cluster 50
Virial coefficient 13
Virial expansion 13 165
Virial, generalized 99
Yukawa potential 115
|
|
 |
| Реклама |
 |
|
|
 |
|
О проекте
|
|
О проекте