We present a bidomain fire-diffuse-fire model that facilitates mathematical analysis of propagating waves of elevated intracellular calcium (
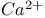
) in living cells. Modeling
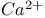
release as a threshold process allows the explicit construction of traveling wave solutions to probe the dependence of
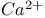
wave speed on physiologically important parameters such as the threshold for
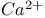
release from the endoplasmic reticulum (ER) to the cytosol, the rate of
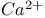
resequestration from the cytosol to the ER, and the total [
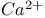
] (cytosolic plus ER). Interestingly, linear stability analysis of the bidomain fire-diffuse-fire model predicts the onset of dynamic wave instabilities leading to the emergence of
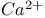
waves that propagate in a back-and-forth manner. Numerical simulations are used to confirm the presence of these so-called ‘tango waves’ and the dependence of
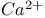
wave speed on the total [
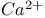
].
 |
|
О проекте
|
|
О проекте


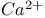 ) in living cells. Modeling
) in living cells. Modeling 