|
|
 |
| Авторизация |
|
|
 |
| Поиск по указателям |
|
 |
|
 |
|
|
 |
 |
|
 |
|
| Veblen O., Young J.W. — Projective Geometry. Vol 1 |
|
|
 |
| Предметный указатель |
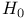 , assumption 45 , assumption 45
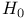 , role of 81 261 , role of 81 261
Abelian group 67
Abscissa 170
Abstract science 2
Addition, of points 142 231
Addition, other definitions of 167 exs.3 4
Addition, theorems on 142—144
Adjacent sides or vertices of simple n-line 37
Algebraic curve 259
Algebraic problem 238
Algebraic surface 259
Alignment, assumptions of 16
Alignment, consistency of assumptions of 17
Alignment, theorems of, for 3-space 20—24
Alignment, theorems of, for 4-space 25 ex.
Alignment, theorems of, for n-space 29—33
Alignment, theorems of, for the plane 17—20
Amodeo, F. 120 294
Anharmonic ratio 159
Apollonius 286
Associative law, for addition of points 143
Associative law, for correspondences 66
Associative law, for multiplication of points 146
Assumption 298
Assumption, 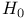 45 45
Assumption, 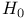 , role of 81 261 , role of 81 261
Assumption, of projectivity 95
Assumption, of projectivity, alternative forms of 105 106 exs.10—12
Assumptions, are necessary 2
Assumptions, categoricalness of 6
Assumptions, consistency of 3
Assumptions, examples of, for a mathematical science 2
Assumptions, for an n-space 33
Assumptions, independence of 6
Assumptions, of alignment 16
Assumptions, of alignment, consistency of 17
Assumptions, of closure 24
Assumptions, of extension 18 24
Axial pencil 55
Axial perspectivity 57
Axis, of coordinates 169 191
Axis, of homology 104
Axis, of pencil 55
Axis, of perspective collineation 72
Axis, of perspectivity 36
Axis, of projectivity on conic 218
Base, of pencil of complexes 332
Base, of plane of points or lines 55
Bilinear equation, binary, represents projectivity on a line 156
Bilinear equation, ternary, represents correlation in a plane 267
Binary form 251 252 254
Bocher, M. 156 272 289 330
Braikenridge 119
Brianchon point 111
Brianchon's theorem 111
Bundle, of complexes 334 ex.
Bundle, of conies 297 exs.9—12
Bundle, of planes or lines 27 55
Bundle, of quadrics 311
Burnside, W. 150
Bussey, W.H. 202
Canonical forms, of collineations in plane 274—276
Canonical forms, of correlations in a plane 281
Canonical forms, of pencils of conies 287—293
Castelnuovo, G. 139 140 237 297
Categorical set of assumptions 6
Cayley, A. 52 140
Center, of bundle 55
Center, of coordinates 170
Center, of flat pencil 55
Center, of homology 104
Center, of perspective collineation in plane 72
Center, of perspective collineation in space 75
Center, of perspectivity 36
Center, of projectivity on conic 218
Central perspectivity 57
Characteristic constant of parabolic projectivity 207
Characteristic equation of matrix 165
Characteristic throw and cross ratio 212 exs.5 7
Characteristic throw and cross ratio, of involution 206
Characteristic throw and cross ratio, of one-dimensional projectivity 205 211
Characteristic throw and cross ratio, of parabolic projectivity 206
Chasles 125
Class, elements of 2
Class, notation for 57
Class, notion of 2
Class, relation of belonging to a 2
Class, subclass of a 2
Class, undefined 15
Clebseh, A. 289
Cogredient n-line 84 ex.
Cogredient triangle 84 exs.7 10
Collineation, between two planes, analytic form of 190
Collineation, characteristic equation of 272
Collineation, defined 71
Collineation, double elements of, in plane 271
Collineation, in plane, analytic form of 189 190 268
Collineation, in space, analytic form of 200
Collineation, invariant figure of, is self-dual 272
Collineation, is the product of two polarities 265
Collineation, leaving conic invariant 214 220 235 ex.
Collineation, perspective, in plane 72
Collineation, perspective, in space 75
Collineation, transforming a conic into a conic 132
Collineation, transforming a five-point into a five-point 77
Collineation, transforming a quadrangle into a quadrangle 74
Collineation, which is the product of two reflections 282 ex.
Collineations 135 ex. 136 ex.
Collineations, associated with two conies of a pencil 131 exs.2 4 6
Collineations, canonical forms of 274—276
Collineations, group of, in plane 268
Collineations, represented by matrices 268—270
Collineations, two, not in general commutative 268
Collineations, types of, in plane 106 273
Commutative correspondence 66
Commutative group 67 70 ex. 228
Commutative law of multiplication 148
Commutative projectivities 70 210 228
Compass, constructions with 246
Complete n-line, in plane 37
Complete n-line, on point 38
Complete n-plane, in space 37
Complete n-plane, on point 38
Complete n-point, in plane 37
Complete n-point, in space 36
Complete quadrangle and quadrilateral 44
Complex, determined by correspondence between points and planes of space 324
Complex, determined by five independent lines 324
Complex, determined by skew pentagon 319
Complex, determined by two projective fiat pencils 323
Complex, equation of 329 331
Complex, general and special 320
Complex, generated by involution on regulus 326 ex.
Complex, linear 312
Complex, null system of 324
Complexes, bundle of 334 ex.
Complexes, in involution 333
Complexes, pencil of 332
Con well, G.M. 204
Concrete representation or application of an abstract science 2
concurrent 16
Cone 118
Cone, as degenerate case of quadric 308
Cone, of lines 109
Cone, of planes 109
Cone, section of, by plane, is conic 109
Configuration 38
Configuration, of Desargues 40 51
Configuration, of Mobius 326 ex.
| Configuration, of Pappus 98 249
Configuration, quadrangle-quadrilateral 44
Configuration, symbol of 38
Congruence, determined by four independent lines 317
Congruence, determined by projective planes 317
Congruence, determined by two complexes 325
Congruence, elliptic, hyperbolic, parabolic, degenerate 315
Congruence, equation of 329 330
Congruence, linear 312
conic 109 118 294 exs.3 4
Conic section 118
Conic, determined by conjugate points 293 ex.
Conic, equation of 185 245
Conic, intersection of line with 240 242 246
Conic, polar system of 120—124
Conic, projectivity on 217
Conic, theorems on 109—140
Conic, through four points and meeting given line in two points harmonic with two given points 250 ex.
Conic, through four points and tangent to line 250 ex.
Conic, through three points and tangent to two lines 250 ex.
Conies, pencils and ranges of 128—136 287—293
Conies, projective 212 304
Conjugate groups 209
Conjugate pair of involution 102
Conjugate points (lines) 140 ex. 293 ex.
Conjugate points (lines), on line (point), form involution 124
Conjugate points (lines), with regard to a pencil of conies 136 ex.
Conjugate points (lines), with regard to conic 122
Conjugate projectivities 208
Conjugate projectivities, conditions for 208 209
Conjugate subgroups 211
Consistency, of a set of assumptions 3
Consistency, of assumptions of alignment 17
Consistency, of notion of elements at infinity 9
Construct 45
Constructions, linear (first degree) 236
Constructions, of second degree 245 249—250
Constructions, of third and fourth degrees 294—296
Contact, of second order between two conies 134
Contact, of third order between two conies 136
Contact, point of, of line of line conic 112
Coordinates, homogeneous, in space 194
Coordinates, homogeneous, of points and lines in plane 174
Coordinates, homogeneous, of points on line 163
Coordinates, in a bundle 179 ex.
Coordinates, Klein's line 333
Coordinates, nonhomogeneous, in space 190
Coordinates, nonhomogeneous, of lines in plane 170
Coordinates, nonhomogeneous, of points in plane 169
Coordinates, nonhomogeneous, of points on line 152
Coordinates, of quadrangle-quadrilateral configuration 181 ex.
Coordinates, Pliicker's line 327
Coplanar 24
Copunctal 16
Correlation, between two planes, analytic representation of 266 267
Correlation, between two-dimensional forms 262 263
Correlation, between two-dimensional forms determined by four pairs of homologous elements 264
Correlation, double pairs of a 278—281
Correlation, induced 262
Correlation, represented by matrices 270
Correlation, represented by ternary bilinear form 267
Correlation, which interchanges vertices and sides of triangle is polarity 264
Correlations and duality 268
Correspondence 293 ex.
Correspondence, (1, 1) of two figures 35
Correspondence, as a logical term 5
Correspondence, general theory of 64—66
Correspondence, identical 65
Correspondence, inverse of 65
Correspondence, involutoric or reflexive 66
Correspondence, period of 66
Correspondence, periodic or cyclic 66
Correspondence, perspective 12
Correspondence, perspective between two planes 71
Correspondence, quadratic 139 exs.22 24
Correspondences, associative law for 66
Correspondences, commutative 66
Correspondences, groups of 67
Correspondences, leaving a figure invariant form a group 68
Correspondences, resultant or product of two 65
Corresponding elements 35
Corresponding elements, doubly 102
Covariant 257
Covariant, example of 258
Cremona, L. 137 138
Cross ratio 159
Cross ratio, as an invariant of two quadratic binary forms 254 ex.
Cross ratio, characteristic, of involution 206
Cross ratio, characteristic, of projectivity 205
Cross ratio, definition of 160
Cross ratio, expression for 160
Cross ratio, in homogeneous coordinates 165
Cross ratio, of four complexes 332
Cross ratio, of harmonic set 159 161
Cross ratio, theorems on 167 168
Cross ratios, the six, defined by four elements 161
Curve, algebraic 259
Curve, of third order 217 exs.7 8 9
Cyclic correspondence 66
Darboux, G. 95
Degenerate conies 126
Degenerate regulus 311
Degree of geometric problem 236
Derivative 255
Desargues, configuration of 40 51
Desargues, theorem on conies 127 128
Desargues, theorem on perspective triangles 41 180
Descartes, R. 285
Diagonal point (line), of complete n-point (n-line) in plane 44
Diagonal point (line), of complete quadrangle (quadrilateral) 44
Diagonal triangle of quadrangle (quadrilateral) 44
Dickson, L.E. 66
Difference of two points 148
Differential operators 256
Dimensions, assumptions for space of n 33
Dimensions, space of five 331
Dimensions, space of n 30
Dimensions, space of three 20
Directrices, of a congruence 315
Directrices, of a regulus 299
Directrices, of a special complex 324
Distributive law for multiplication with respect to addition 147
Division of points 149
Domain of rationality 238
Double element (point, line, plane) of correspondence 68
Double pairs of a correlation 97
Double points, and lines of collineation in plane 271 295
Double points, of a projectivity on a line satisfy a quadratic equation 156
Double points, of involution determined by covariant 258
Double points, of projectivity always exist in extended space 242
Double points, of projectivity on a line, construction of 246
Double points, of projectivity on a line, homogeneous coordinates of 164
Double ratio 159
Doubly parabolic point 274
Duality, a consequence of existence of correlations 268
Duality, at a point 29
Duality, in four-space 29
Duality, in plane 29
Duality, in three-space 28
Edge of n-point or n-plane 36 37
Elation, in plane 72
Elation, in space 75
Element, fundamental 1
Element, ideal 7
Element, invariant, or double, or fixed 68
Element, lineal 107
Element, of a figure 1
Element, simple, of space 34
Element, undefined 1
Eleven-point, plane section of 53 ex.
|
|
 |
| Реклама |
 |
|
|
 |
|
О проекте
|
|
О проекте



 , assumption
, assumption