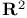|
|
 |
| Авторизация |
|
|
 |
| Поиск по указателям |
|
 |
|
 |
|
|
 |
 |
|
 |
|
| Kreyszig E. — Introductory functional analysis with applications |
|
|
 |
| Предметный указатель |
 11 11
 118 118
 , ,  18 18
![$BV[a, b]$](/math_tex/7a2cfdf3997617b0b55ddeeed22fbc1082.gif) 226 226
![$B[a, b]$](/math_tex/862a27fa7eb09b4733889e23d9c4ac2e82.gif) 11 25 11 25
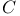 34 271 34 271
 411 411
![$C[a, b]$](/math_tex/e77e794b3859783600b5f8f2bfed341e82.gif) 17 36 52 61 106 134 329 333 342 17 36 52 61 106 134 329 333 342
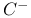 22 28 51 395 22 28 51 395
 70 126 255 70 126 255
![$C^{1}[a, b]$](/math_tex/1ec63caf4649885762aa33e225a3c37682.gif) 110 110
![$C^{2}[a, b]$](/math_tex/88923bf94e4f079413bfed91e552b3ad82.gif) 357 357
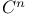 6 33 52 60 131 6 33 52 60 131
![$C’[a, b]$](/math_tex/fddbbe8db3e0861ee6b1c7994531bb2b82.gif) 110 110
 118 118
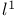 61 121 243 61 121 243
 11 52 106 133 154 194 265 409 11 52 106 133 154 194 265 409
 562 573 562 573
![$L^{2}[a, b]$](/math_tex/8ce01fd9d31de2453d51fb128c6d122182.gif) 62 132 62 132
 11 23 35 61 122 133 261 11 23 35 61 122 133 261
![$L^{p}[a, b]$](/math_tex/a9f8fe062c6b8b887ca149c041c850fe82.gif) 62 62
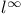 6 22 33 61 6 22 33 61
 378 378
 378 378
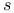 9 63 9 63
 18 18
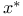 106 106
 120 120
 3 3
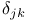 114 114
 -neighborhood 19 -neighborhood 19
 -net 412 -net 412
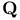 29 37 29 37
 5 22 28 51 395 5 22 28 51 395
 6 33 52 60 121 131 6 33 52 60 121 131
 83 83
 494 494
 292 292
 83 83
 83 83
 371 371
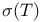 371 371
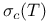 371 371
 371 371
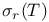 371 371
Abelian algebra 394
Absolute convergence 68
Abstract function 613
Abstract space 1
Accumulation point 21
Adjoint Hilbert 196 236
Adjoint operator 232 416
Adjoint space 120
Algebra 394
Algebraic complement 146
Algebraic dual space 106
Algebraically reflexive 109 115
Alternating set 345
Analytic function 386
Annihilator 126 148 194 238 239
Appolonius’ identity 135
Approximate eigenvalues 545
Approximation 327
Ascoti’s thorem 454
Associated Laguerre polynomials 604
Associated Legendre functions 602
Augmented matrix 450
Axiom of Choice 211
Axioms for a metric 3
Axioms for a norm 59
Axioms for a topology 19
Axioms for a vector space 50
Axioms for an inner product 129
Baire category theorem 247
Ball 18
Banach algebra 395
Banach fixed point theorem 300 303
Banach space 58
Banach — Steinhaus theorem 246 249
Basis 55 68
Basis, dual 114
Basis, for a normea space 68
Basis, for a vector space 55
Basis, Hamel 55 211
Basis, orthonormal 168
Basis, Schauder 68
Bessel inequality 157 169
Best approximation 328
Bidual space 239
Bijection, bijective 615
Bilinear form 191
Binary relation 618
Biorthogonal system 444
Bohr radius 604
Bolzano — Weierstrass theorem 620
Boundary 24
Bounded inverse theorem 286
Bounded linear extension 100
Bounded linear functional 104 188 225
Bounded linear operator 91
Bounded sequence 26
Bounded sesquilinear form 191
Bounded set 16 26 66
Bounded variation 225
Canonical basis 54
Canonical embedding 108
Canonical mapping 108 240
Cartesian product 612
Category 247
Category theorem 247
Cauchy convergence criterion 620
Cauchy determinant 343
Cauchy sequence 28
Cauchy — Schwarz inequality 14
Cayley transform 556
Center of an algebra 398
Ces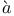 ro summabiiity method 214 275 ro summabiiity method 214 275
Chain 210
Characteristic determinant 365
Characteristic equation 365
Characteristic polynomial 365
Chebyshev approximation 336
Chebyshev polynomials 348
Closable operator 537
Closed ball 18
Closed extension 297
Closed graph theorem 292
Closed linear operator 292 535
Closed set 18 30
Closed subspace 30
Closure of a set 21 30
Closure of an operator 537
Codimension 57
Coefficient field 51
Column sum criterion 314
Commutative algebra 394
Commutator 578
Commuting operators 90 561
Compact 77
Compact, countably 619
Compact, extension 415
Compact, locally 82
Compact, metric space 77
Compact, operator 405
Compact, relatively 406
Compact, sequentially 619
Compact, set 77 619
Compact, topological space 619
Compactness of unit ball 80
Comparable elements 210
| Compatible norm 102
Complement 609
Complementary pair of subspaces 146
Complete inner product space 128
Complete metric space 28
Complete normed space 59 73
Complete orthonormal sequence 168
Complete orthonormal set 168
Completely continuous operator 405
Completeness of spaces, 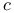 34 34
Completeness of spaces, ![$C[a, b]$](/math_tex/e77e794b3859783600b5f8f2bfed341e82.gif) 36 36
Completeness of spaces, 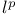 35 35
Completeness of spaces, 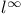 33 33
Completeness of spaces, 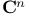 33 33
Completeness of spaces,  33 33
Completion of a metric space 41
Completion of a normed space 69
Completion of an inner product space 139
Complex  -dimensional space -dimensional space 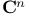 6 33 52 60 131 6 33 52 60 131
Complex plane 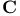 6 22 28 395 6 22 28 395
Complex vector space 51
Composition of mappings 616
Conjugate exponents 12
Conjugate linear 130 191
Conjugate space 120
Continuity of a norm 60
Continuity of an inner product 138
Continuity of vector space operations 70
Continuous extension 100
Continuous functional 104
Continuous functions 7 36 38
Continuous mapping 20 25 30 81
Continuous operator 96
Continuous spectrum 371
Continuous, completely 405
Contraction 300 303
Convergence, absolute 68
Convergence, in metric spaces 25
Convergence, in normed spaces 67
Convergence, of a sequence 25 67 620
Convergence, of a series 68
Convergence, of numerical integration processes 278
Convergence, strong 256 266
Convergence, strong operator 264
Convergence, uniform 37
Convergence, uniform operator 263
Convergence, weak 257 266
Convergence, weak operator 264
Convergence, weak 266 266
Convex function 334
Convex set 65 143 331
Convex strictly 332
Coset 57
Countable set 612
Countably compact 619
Cover, covering 618
Cross product 85
De la Vall e — Poussin theorem 343 e — Poussin theorem 343
de Morgan’s laws 612
Decomposition of unity 494
Decreasing sequence of operators 473
Deficiency index 562
Definite integral 105
Degenerate kernel 457
Dense 21
Densely defined 527
Diameter of a set 16
Difference of projections 487
Difference of sets 609
Differential equation 315
Differentiation operator 84 93 294 567
Dimension, Hilbert 168 173
Dimension, of a vector space 55
Dimension, orthogonal 168
Direct method 307
Direct sum 146 433
Discrete metric 8 22
Discrete spectrum 371
Distance between points 4
Distance between sublets 16
Distance from a subspace 142
Distance function 3
Divergent sequence 269
Division algebra 403
Domain in the complex plane 386
Domain of a mapping 613
Domain of an operator 83
Dot product 85 105 131
Dual basis 114
Dual space 120
Dual space, algebraic 106
Eigenfunction 372
Eigenspace 365 372
Eigenvalue 364 366
Eigenvector 364 372
Embedding 109 241
Empty set 609
Equality of operators 99
Equicontinuity 454
Equivalence relation 618
Equivalent norms 75 291
Euclidean metric 5 6
Euclidean plane 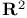 5 5
Euclidean space  6 33 52 60 121 131 6 33 52 60 121 131
Euler formulas 160
Euler summabiiity method 275
Existence theorem for best approximations 328
Existence theorem for differential equations 315
Existence theorem for fixed points 300
Existence theorem for minimizing vectors 144
Existence theorem for splines 358
expansion 68
Extension ,proper 616
Extension of a functional 117 214 219 221
Extension of a mapping 616
Extension of an operator 99 297 526
Extension problem 2 3
Extremal point 337 338
Extreme point 336
Factor space 57
Family of sets 617
Finite dimensional normed space 72 96 111
Finite dimensional subspace 73
Finite dimensional vector space 54
Finite rank 408
Finite set 612
First category 247
Fixed point 299 323
Fourier coefficients 157 165
Fourier series 160 251
Fredholm alternative 451
Fredholm integral equation 319
Fredholm theorems 442
Fredholm theory 405
Frobenius theorem 469
Frontier 24
Function space 7
Functional 103
Functional, bounded linear 104
Functional, linear 104
Functional, positive homogeneous 214
Functional, relation 613
Functional, subadditive 214
Functional, sublinear 213
Fundamental orthonormal set 168
Fundamental sequence 28
Fundamental set 168
Gauss — Seidel iteration 311
GENERATED 53
Generating function 186 187
|
|
 |
| Реклама |
 |
|
|
 |
|
О проекте
|
|
О проекте





 ,
, 
![$BV[a, b]$](/math_tex/7a2cfdf3997617b0b55ddeeed22fbc1082.gif)
![$B[a, b]$](/math_tex/862a27fa7eb09b4733889e23d9c4ac2e82.gif)
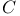

![$C[a, b]$](/math_tex/e77e794b3859783600b5f8f2bfed341e82.gif)
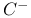

![$C^{1}[a, b]$](/math_tex/1ec63caf4649885762aa33e225a3c37682.gif)
![$C^{2}[a, b]$](/math_tex/88923bf94e4f079413bfed91e552b3ad82.gif)
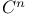
![$C’[a, b]$](/math_tex/fddbbe8db3e0861ee6b1c7994531bb2b82.gif)

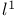


![$L^{2}[a, b]$](/math_tex/8ce01fd9d31de2453d51fb128c6d122182.gif)

![$L^{p}[a, b]$](/math_tex/a9f8fe062c6b8b887ca149c041c850fe82.gif)
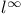


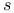

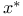


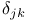
 -neighborhood
-neighborhood 









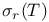
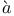 ro summabiiity method
ro summabiiity method 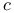
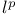
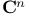
 -dimensional space
-dimensional space 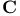

 e — Poussin theorem
e — Poussin theorem