Due to their special structure beamlike components in multibody systems may undergo elastic deformations on the influence of inertia forces and imposed loads. Often it is not possible to estimate the loading and the resulting deformations in advance. For this a genuine nonlinear formulation is derived which treats small and large deformations in the same way and which is suitable for static as well as dynamic computations.
The equations for a geometrically nonlinear beam with shear flexibility are derived in a consistent manner from the three-dimensional theory of elasticity. As a result a beam configuration is considered as a parameterised curve on the Lie Group SE(3) = SO(3) к
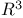
. Suitable quantities for velocity, variations and strain are defined on the Lie Algebra SE(3) through left reduction with respect to the semidirect product. In this way the corresponding equations of motion and variational principles appear in an SE(3)-invariant form. With the same systematic approach the dynamics of a free rigid body is addressed, and by this an Euclidean extension of Kirchhoff's kinetic analogy is obtained.
The reduction to an intrinsic beam equation is separated entirely from the following discretisation process. For this a modified version of the co-rotational Finite Element method is used. As in the conventional method the interpolation is carried out with respect to the co-rotating system, but with the difference that the local nodal variables are retained and not transformed to absolute nodes. As the main advantages reliable linear shape functions can be used, and a connection to a minimal formulation via the local nodal variables can be established. In addition, the shape functions depend on shear deformation parameters that allow for switching to the normal-hypothesis on element level without changing the mechanical formulation.
Such an elastic element is then interpreted as a kinetostatic transmission element with the local nodal variables as internal variables. In this setting the forward kinematics as well as the backward kinetics are expressed entirely in terms of Lie algebraic operations. In addition to the elastic element a rigid element and a joint element are presented as further examples. For the assembly of a system from its transmission elements several recursive methods from multibody dynamics are unified. With these methods the inverse dynamics, the forward dynamics or single multibody terms can be calculated by choice. As a special case this unified formulation also contains the assembly of the beam elements and the integration of the beam in an entire system.
For numerical applications the number of beam elements is used to control the convergence of the solution, but also to tune the time step integration according to the stiffness of the structure. Due to the local calculation of the element forces and the recursive schemes used, the numerical evaluation can be done in real-time as long as the beams are moderately stiff.
Several test examples are used to demonstrate the power of the proposed formulation for static and dynamic problems with large deformations. The numerical results are validated by analytic calculations and compared with other methods.
 |
|
О проекте
|
|
О проекте


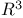 . Suitable quantities for velocity, variations and strain are defined on the Lie Algebra SE(3) through left reduction with respect to the semidirect product. In this way the corresponding equations of motion and variational principles appear in an SE(3)-invariant form. With the same systematic approach the dynamics of a free rigid body is addressed, and by this an Euclidean extension of Kirchhoff's kinetic analogy is obtained.
. Suitable quantities for velocity, variations and strain are defined on the Lie Algebra SE(3) through left reduction with respect to the semidirect product. In this way the corresponding equations of motion and variational principles appear in an SE(3)-invariant form. With the same systematic approach the dynamics of a free rigid body is addressed, and by this an Euclidean extension of Kirchhoff's kinetic analogy is obtained.
