|
|
 |
| Авторизация |
|
|
 |
| Поиск по указателям |
|
 |
|
 |
|
|
 |
 |
|
 |
|
| Ruelle D. — Statistical Mechanics |
|
|
 |
| Предметный указатель |
 — Algebra 145 168—178 199—201 — Algebra 145 168—178 199—201
 — Algebra, classical continuous systems 170 172 — Algebra, classical continuous systems 170 172
 — Algebra, classical lattice systems 168 — Algebra, classical lattice systems 168
 — Algebra, quantum continuous systems 176—178 — Algebra, quantum continuous systems 176—178
 — Algebra, quantum lattice systems 172 173 — Algebra, quantum lattice systems 172 173
 — Algebra, representations 203 204 — Algebra, representations 203 204
 — Algebra, with group of automorphisms 147 — Algebra, with group of automorphisms 147
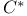 — Algebras 200 — Algebras 200
 -Body interaction or potential 24 30 31 -Body interaction or potential 24 30 31
Activity 6
Affine function 199
Alaoglu — Birkhoff, theorem of 149 150
Alloys 7
Alternating bound property 95
Antiferromagnetism 134 144
Asymptotically Abelian  -algebra 151 -algebra 151
Bogoliubov inequality 130
Boltzmann factors 87 88
Catastrophic interaction or potential 35
chemical potential 5
Close packing 70
Cluster functions 92 107
Cluster property 88 91 92 156 162
Coarse graining 161
Configuration 168 170
Continuous systems 4—6 8—10
Convexity 199
Correlation function 72 77 143 170
Crystal 162
Curie temperature 128
Dyson — Lennard, theorem of 63
Energy 4
Ensembles, canonical 5 9 54 55
Ensembles, classical 3—7
Ensembles, equivalence of 3 11 29 56
Ensembles, grand canonical 5 6 9 10 56 57
Ensembles, microcanonical 4 5 9 52 53
Ensembles, quantum 7—11 (see also thermodynamic limit)
entropy 12 41 54 187
Entropy, of a state 178—184
Equicontinuity 198
Factors 206
ferromagnetism 133 134 144
Field operator 102 172
Finite range interaction or potential 16 31 105
Finite systems 11
Fisher convergence 14 58 69
Fluctuation 161
Fock space and Fock representation 10 173—176
Free energy 56
Friedrichs extension 61 62
Function 199
G — Abelian  -aIgebras 148 -aIgebras 148
Gallavotti — Miracle equations 82 83
Gauge group 163
Gel’fand isomorphism 204 205
Gel’fand — Segal construction 101 147 203
Gibbs phase rule 190—192
Griffiths inequalities 119—128 143
Ground state 67 68
Group with an invariant mean 151—153 155 208 209
Hamiltonian 8 60
Hard core interaction or potential 6 10 30 37 38 58 69 96 106 138 139
Heisenberg model 129
Inequalities 143
Infinite systems 11
Inner automorphisms 151
Integral representations 206—208
Interactions, classical continuous systems 29—33
Interactions, quantum lattice systems 15—18
Invariance group 146
Ising model 127 128
Kac’s formula 97
Kadison’s transitivity theorem 204
Kaplansky’s density theorem 206
Kato, theorem of 60 61
Kirkwood — Salsburg equations 72—74
Kubo — Martin — Schwinger boundary condition 192—196
| Large group of automorphisms 164
Lattice gases 7 127 137
Lattice systems 6 7 10 11 58 79—82 108 134 135
Lee and Yang, theorem of 108—112
Lennard — Jones type potential 40 58 68
Lieb’s inequalities 93
Long range order 128 162
M — Nets 208 209
Magnetic field 7 128
magnetization 128 134 143
Mayer series 84 95 99 105
Mayer — Montroll equations 79 106
Mean ergodic theorem 152
Mermin — Wagner, theorem of 131 133
Minimax principle 25 26 64
Morse potential 68
Nelson, theorem of 103
Observables 145
One-dimensional systems 134—141
Pair interaction or potential 23 31
Partition function 12 (see also Zeros of grand partition function)
Partition function, canonical 54
Partition function, grand 33 56
Partition function, microcanonical 41 52
Peierls’ theorem 27
Periodic boxes 20 23
Perron — Frobenius, theorem of 136 141
Phase 1
Phase transition 108—144
Phase transition, first order 113—119
Phase, pure thermodynamic 161—163 191
Positive interaction or potential 33 58 86 93—96 106
Positive-type pair potentials 38
Pressure 57
Pressure, continuity of 58
Quasilocal algebras 148 151
Reduced density matrices 98 174
Regular potential 72
Segal, theorem of 147
Semicontinuity, upper and lower 198
simplex 207
Spin systems 7 23 127 128
Stable interaction or potential 33—41 62 63
State 11 145 146
State, E1, E2, and E3 163
State, equilibrium 11 168—197
State, ergodic 154—167
State, invariant 146—153
State, on  -algebras 201—205 -algebras 201—205
State, thermodynamic limit 184—187
Statistics 8 63 64
Superstable potential 40
Symmetry breakdown 162 163
System of density distributions 169 170
System of density matrices 173 176 178
Temperature 5
Tempered interaction or potential 32 58 69
Thermodynamic behavior 1
Thermodynamic function 12
Thermodynamic limit 2
Thermodynamic limit, classical ensembles 52—60
Thermodynamic limit, classical lattice systems 20—25
Thermodynamic limit, configurational microcanonical ensemble 41—52
Thermodynamic limit, correlation functions 72
Thermodynamic limit, of states 184—187
Thermodynamic limit, quantum ensembles 60—68
Thermodynamic limit, quantum lattice systems 18—20
Thermodynamic stability 52
Traces 151 165
Ursell functions 87 88 95 106
Van Hove convergence 14 58 69
Van Hove, theorem of 139—141
Variational Principle 187—190
Virial expansion 85 99
Von Neumann algebras 205 206
Von Neumann algebras, enveloping 204
Weakly asymptotically Abelian  -algebra 151 165 -algebra 151 165
Wightman construction 101
Zeros of grand partition function 69 77 110
|
|
 |
| Реклама |
 |
|
|
 |
|
О проекте
|
|
О проекте



 — Algebra
— Algebra 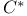 — Algebras
— Algebras  -Body interaction or potential
-Body interaction or potential