Авторизация
Поиск по указателям
Domb C., Lebowitz J.L. — Phase transitions and critical phenomena (Vol. 11)
Обсудите книгу на научном форуме Нашли опечатку?
Название: Phase transitions and critical phenomena (Vol. 11)Авторы: Domb C., Lebowitz J.L. Аннотация: This series of publications was first planned by Domb and Green in 1970. During the previous decade the research literature on phase transitions and critical phenomena had grown rapidly and, because of the interdisciplinary nature of the field, it was scattered among physical, chemical, mathematical and other journals. Much of this literature was of ephemeral value, and was rapidly rendered obsolete. However, a body of established results had accumulated, and the aim was to produce articles that would present a coherent account of all that was definitely known about phase transitions and critical phenomena, and that could serve as a standard reference, particularly for graduate students.
Язык: Рубрика: Физика /Статус предметного указателя: Готов указатель с номерами страниц ed2k: ed2k stats Год издания: 1987Количество страниц: 210Добавлена в каталог: 19.03.2006Операции: Положить на полку |
Скопировать ссылку для форума | Скопировать ID
Предметный указатель
see "Ashkin — Teller" see "Clock models" 86 Abelian, groups 200 Amplitude ratios 71 ANNNI model 168 Antivortex 22 Asano contractions 195 Ashkin — Teller (1943) model 2 3 26 35—36 51 71 115 Ashkin — Teller (1943) model, partition sum 34 Baxter's exact solution 32 Baxter's exact solution, Potts model 200 Baxter's exact solution, scaling dimensions 70 Baxter's exact solution, symmetric eight-model vertex 70 BCSOS model 30—32 34—40 43 45 BKL (Bicmont, Kuroda and Lebowitz) 167 Blume — Capel 133 Bose — Einstein statistics 4 Boundary conditions, antiperiodic 77 118 Boundary conditions, free 84 Boundary conditions, Gibbs states see "Gibbs" Boundary conditions, periodic 85 95 Boundary conditions, twisted 77 BPZ (Belavin, Polyakov and Zamolodchikov) 56—59 68 94 102—103 107 115 118—119 Charge, asymmetry 17 Charge, inversion symmetry 16 Charge, neutrality condition 5 18 19 106 Classical Heisenberg Ferromagnetic, two-dimensional anisotropic 166 Clock Models 23 24 28 201 Clock models, 2 23 26 Clock models, four-state 33 Cones (conical geometry) 86 88 Conformal transformations 56 64—65 78 79 80 Conformal transformations in two dimensions 89 Conformal transformations, bootstrap 103 contours 152—153 Contours with a parameter 161—162 Contours with interactions 167 Contours, contour models 155—156 Contours, rarefied ensembles 157 166 Contours, renormalized 168 Corner transfer matrix 88 Corrections to scaling 76 Correlation function, four-point 67 100—103 106 108 119 Correlation function, six-point 67 Correlation function, three-point 66 74 102 110 Correlation function, two-point 19 84 Correlation function, XY model 25 Correlation, length amplitudes 69 Coulomb gas (CG) model 2—51 59 102 104 119 Coulomb gas (CG) model, electromagnetic CG 16 Coulomb gas (CG) model, magnetic CG 14 Coulomb gas (CG) model, modified 105 Critical exponents 14 35 56 Critical exponents, points (line of) 29 45 Critical exponents, three-state Potts 42 Cubic model 3 50 Delta squared model 133—134 139 145 152 165 176 Delta squared model with an infinite spin 169 Delta squared model, Phase diagram 178 Delta squared model, spin-1 177 179 Delta squared model, spin-2 178 den Nijs formula 110 Dimer models 3 Directed percolation 60 Dirichlet series 170 172 domain walls 37 40—41 44 48 Dual string model 56 Dumbbell 12 Dynamic critical phenomena 79 Eight-vertex (8V) (symmetric) model 2 3 30 32 115 Energy-momentum tensor 56 Euler's relation 37 F model 30 31 35 39 F model, Kagome 46 Finite-size scaling 57 68 69 72 79 88 115 121 Finite-size scaling, corrections to 75 First-order phase transitions 137 139 Fixed points 11 13 15 16 18 60 81 90 160 163—164 Fixed points, Hamiltonian 61—3 65 67 FQS (Friedan, Qiu and Shenker) 57 58 99—100 103 107 111 113—116 Free fermions 108 Fugacity 4 6 8 9 11—19 25 26 31 38 45 49 Fugacity, electric 28 G (Griffiths, 1972) 131 G-phase 150 Gauge theories 140 172 197 200 Gaussian model 2 24 42 70 71 84 104 107 115 166 Gaussian model, discrete 27 28 29 Gibbs states 184—185 187—188 193—195 Gibbs states, boundary conditions 135—136 140—142 Gibbs states, phase rule 138 GKS inequalities (Griffiths — Sherman — Kelly) 183 184 Ground state entropy 200 Hard Hexagon problem 51 Hard square model 51 Heisenberg model 2 high-temperature expansion 48—49 Ice rule 31 Ising models (IM) 26 51 71 73 76—77 88 109 116 119 132 143 186 191 Ising models (IM) on a triangular lattice 144 172 Ising models (IM) on the sub-lattice 199 Ising models (IM), antiferromagnetic (FCC) lattice 134 139 145 168 Ising models (IM), ferromagnetic 139 144 Ising models (IM), frustrated 3 50 Ising models (IM), Hamiltonian 137 Ising models (IM), one-dimensional 142 193—194 Ising models (IM), phase diagram 148 180 Ising models (IM), spin- 145 196 Ising models (IM), spin-1 model 128 130 Ising models (IM), spontaneous magnetization 50 Ising models (IM), three-dimensional 142 Ising models (IM), tricritical 59 111—121 118 Ising models (IM), two-dimensional 82 187 195 Ising models (IM), universality class 35 Isotropic n-vector see "O(n) model" Kac-determinant 56 57 Kac-determinant, formula 96 100 103 106 107 112 116 Kagome SOS (KSOS) 43 Kagome SOS (KSOS), F-model 46 Kagome SOS (KSOS), lattice 44—45 Kirkwood — Salsburg equations 157 Kosterlitz and Thouless transition (KT) 20 22 25—29 Landau — Ginzburg Hamiltonian 98 Landau — Ginzburg Hamiltonian, theory 114 Lattice-gas 5 Lattice-gas, animals 116 Lattice-gas, Lifshitz points 60 Line defects 88 Mean field theory 80 86 Melting 2 Minlos — Sinai method 157—158 161 Mobius transformation 66 97 Monomials 190 Monte Carlo method 73 Monte Carlo method, simulations 85 86 Multi-point correlation function 56 58 62 Multi-spin interactions 183 Multicritical point 81 113 114 Multiple correlation functions 50 Multiplicity functions 136 173 Neveu — Schwartz algebra 118 O(n) models 3 42 45—46 48—50 82 112 121 Operator product coefficients, expansion 63 Operator product coefficients, universal 75 Parafermion see "Spinor" Particle annihilation 9 Particle annihilation, fusion 8 Partition functions, crystal rarefied 154 Pecherski's model 149—150 172 Peierls, argument 130 167 168 193 Peierls, bound 130—131 155 Peierls, condition 129 141—145 147—149 Percolation 98 110 Periodic boundary conditions 68 Pirogov — Sinai (PS) theory 128—131 133—4 138 140—2 152 161 164 166 167—169 178 180 181 193 Polymers 42 50 Potts models 37—41 51 152 200 Potts models on the triangular lattice 45 Potts models, antiferromagnets 3 50 Potts models, critical point 45 51 Potts models, estate 3 36 59 70 78 108 111 121 167 201 Potts models, four-state 77 84 Potts models, RG flow lines 46 Potts models, three-state 26 84—85 102 111 115 Potts models, vector model 201 see Quantum field theories (QFT) 56 166 Quantum Ising chain, tricritical 71 Quenched random systems 98 Ramond algebra 118 Recursion relations (Coulomb gas) 10 Reduced models 193 Renormalization group (Coulomb gas) 6 56 57 60 Renormalization group (Coulomb gas) in real space 183 Renormalization group (Coulomb gas), trajectories 15 Restricted geometries 68 Roughening models 27 Roughening models, transition 28 S (Sinai, 1982) 131 Scaling, Baxter model 70 Scaling, densities 61 Scaling, dimensions 62 68 77—78 86 88 93—94 96—97 103—104 106—107 110 117 121 Scaling, fields 19 57 60 Scaling, finite-size 115 Scaling, functions 82 85 Scaling, operators 73 Scaling, relation 115 Schwartz — Christoffel transformation 85 Schwinger — Dyson equations 68 Self-avoiding walks 83 84 112 Semi-infinite geometry 79 Semi-infinite systems 58 59 119 Six-vertex (6V) model 30 32 33 35 36 38—39 43 45 Solid-on-solid models (SOS) 28 29 43 59 113 Spherical model 79 Spinor operator or parafermion operator 42 Square Model 186—188 193 Star-triangle transformations 88 Stress tensor 90 105 Stress tensor, superstress 118 Structure factor 86 Super-symmetry 59 116 Superconformal transformations 117 Surface, critical behaviour 80 119 Surface, critical behaviour, critical exponents 82 120 Surface, critical behaviour, extraordinary transition 81 82 120 Surface, ordinary transition 120 Surface, ordinary transition, roughening 2 Surface, ordinary transition, scaling exponents 59 Surface, ordinary transition, special transition 81 82 Symmetric eight-vertex Baxter model 70 Symmetry-breaking, cubic and uniaxial 48 49 50 Tetracritical point 115 Toroidal geometry 76 Transfer matrix 58 70 72 73 74 79 88 94 108 109 118 Transfer matrix, corner 88 transitions see "Surface" Tricritical, cross-over exponent 40 Tricritical, indices (Ising) 51 Tricritical, Ising model 118 Tricritical, O(n) model 116 Tricritical, point 39 Tricritical, three-state Potts 42 Triple point 128 TSOS (Triangular solid-on-solid) model 44 46 Ultraviolet (uv) cut-off 4 Universal-amplitude 84 Universal-amplitude, quantities 72 Universality 3 39 51 59 71 96 97 100 107 Ursell functions 173 174 Van Hove theory 80 Virasoro algebra 56 58 95 97 98 100 117 Vortex excitations 2 Vortex excitations, fugacity 26 Vortex-antivortex correlation function 46 Vortices 22 29 Ward identities 58 93 110 Ward identities, conformal 89 91 105 119 Wedge geometry 86 88 Whitney polynomial 37 Widom — Rowlinson model 167 XY model 20 22 24 28 48 49 XY model in a field 26 XY model, Correlation functions 25 Yang — Lee edge singularity 115
Реклама
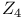model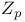 
models 
-expansion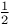 
model 
|
|
О проекте